In mathematics, the concept of a measure is a generalization and formalization of geometrical measures and other common notions, such as magnitude, mass, and probability of events. These seemingly distinct concepts have many similarities and can often be treated together in a single mathematical context. Measures are foundational in probability theory, integration theory, and can be generalized to assume negative values, as with electrical charge. Far-reaching generalizations of measure are widely used in quantum physics and physics in general.
In mathematical analysis and in probability theory, a σ-algebra on a set X is a nonempty collection Σ of subsets of X closed under complement, countable unions, and countable intersections. The ordered pair is called a measurable space.
In mathematics, the Lp spaces are function spaces defined using a natural generalization of the p-norm for finite-dimensional vector spaces. They are sometimes called Lebesgue spaces, named after Henri Lebesgue, although according to the Bourbaki group they were first introduced by Frigyes Riesz.
In the mathematical field of real analysis, the monotone convergence theorem is any of a number of related theorems proving the convergence of monotonic sequences that are also bounded. Informally, the theorems state that if a sequence is increasing and bounded above by a supremum, then the sequence will converge to the supremum; in the same way, if a sequence is decreasing and is bounded below by an infimum, it will converge to the infimum.
In mathematical analysis, Hölder's inequality, named after Otto Hölder, is a fundamental inequality between integrals and an indispensable tool for the study of Lp spaces.
In mathematics, specifically measure theory, the counting measure is an intuitive way to put a measure on any set – the "size" of a subset is taken to be the number of elements in the subset if the subset has finitely many elements, and infinity if the subset is infinite.
In mathematics, Fatou's lemma establishes an inequality relating the Lebesgue integral of the limit inferior of a sequence of functions to the limit inferior of integrals of these functions. The lemma is named after Pierre Fatou.
In mathematics, the Radon–Nikodym theorem is a result in measure theory that expresses the relationship between two measures defined on the same measurable space. A measure is a set function that assigns a consistent magnitude to the measurable subsets of a measurable space. Examples of a measure include area and volume, where the subsets are sets of points; or the probability of an event, which is a subset of possible outcomes within a wider probability space.
In measure theory, Lebesgue's dominated convergence theorem provides sufficient conditions under which almost everywhere convergence of a sequence of functions implies convergence in the L1 norm. Its power and utility are two of the primary theoretical advantages of Lebesgue integration over Riemann integration.
In mathematics, particularly in functional analysis, a projection-valued measure is a function defined on certain subsets of a fixed set and whose values are self-adjoint projections on a fixed Hilbert space. A projection-valued measure (PVM) is formally similar to a real-valued measure, except that its values are self-adjoint projections rather than real numbers. As in the case of ordinary measures, it is possible to integrate complex-valued functions with respect to a PVM; the result of such an integration is a linear operator on the given Hilbert space.
In mathematics, signed measure is a generalization of the concept of (positive) measure by allowing the set function to take negative values, i.e., to acquire sign.
In mathematics, the Bochner integral, named for Salomon Bochner, extends the definition of Lebesgue integral to functions that take values in a Banach space, as the limit of integrals of simple functions.
In mathematics, a π-system on a set is a collection of certain subsets of such that
In mathematics, a positive (or signed) measure μ defined on a σ-algebra Σ of subsets of a set X is called a finite measure if μ(X) is a finite real number (rather than ∞). A set A in Σ is of finite measure if μ(A) < ∞. The measure μ is called σ-finite if X is a countable union of measurable sets each with finite measure. A set in a measure space is said to have σ-finite measure if it is a countable union of measurable sets with finite measure. A measure being σ-finite is a weaker condition than being finite, i.e. all finite measures are σ-finite but there are (many) σ-finite measures that are not finite.
In mathematics, the support of a measure on a measurable topological space is a precise notion of where in the space the measure "lives". It is defined to be the largest (closed) subset of for which every open neighbourhood of every point of the set has positive measure.
Convergence in measure is either of two distinct mathematical concepts both of which generalize the concept of convergence in probability.
In mathematics, in particular in measure theory, a content is a real-valued function defined on a collection of subsets such that
In mathematics, the Pettis integral or Gelfand–Pettis integral, named after Israel M. Gelfand and Billy James Pettis, extends the definition of the Lebesgue integral to vector-valued functions on a measure space, by exploiting duality. The integral was introduced by Gelfand for the case when the measure space is an interval with Lebesgue measure. The integral is also called the weak integral in contrast to the Bochner integral, which is the strong integral.
In mathematics, especially measure theory, a set function is a function whose domain is a family of subsets of some given set and that (usually) takes its values in the extended real number line which consists of the real numbers and

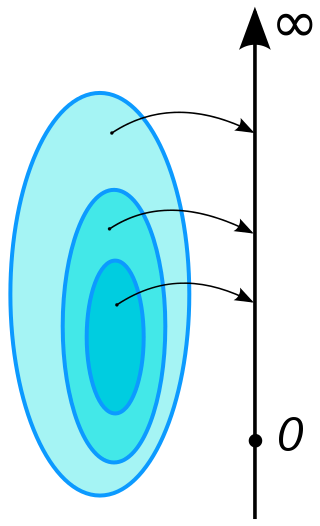
In mathematics, the integral of a non-negative function of a single variable can be regarded, in the simplest case, as the area between the graph of that function and the X axis. The Lebesgue integral, named after French mathematician Henri Lebesgue, extends the integral to a larger class of functions. It also extends the domains on which these functions can be defined.





















