In mathematics, the concept of a measure is a generalization and formalization of geometrical measures and other common notions, such as magnitude, mass, and probability of events. These seemingly distinct concepts have many similarities and can often be treated together in a single mathematical context. Measures are foundational in probability theory, integration theory, and can be generalized to assume negative values, as with electrical charge. Far-reaching generalizations of measure are widely used in quantum physics and physics in general.
In mathematics, particularly linear algebra and functional analysis, a spectral theorem is a result about when a linear operator or matrix can be diagonalized. This is extremely useful because computations involving a diagonalizable matrix can often be reduced to much simpler computations involving the corresponding diagonal matrix. The concept of diagonalization is relatively straightforward for operators on finite-dimensional vector spaces but requires some modification for operators on infinite-dimensional spaces. In general, the spectral theorem identifies a class of linear operators that can be modeled by multiplication operators, which are as simple as one can hope to find. In more abstract language, the spectral theorem is a statement about commutative C*-algebras. See also spectral theory for a historical perspective.
In calculus and real analysis, absolute continuity is a smoothness property of functions that is stronger than continuity and uniform continuity. The notion of absolute continuity allows one to obtain generalizations of the relationship between the two central operations of calculus—differentiation and integration. This relationship is commonly characterized in the framework of Riemann integration, but with absolute continuity it may be formulated in terms of Lebesgue integration. For real-valued functions on the real line, two interrelated notions appear: absolute continuity of functions and absolute continuity of measures. These two notions are generalized in different directions. The usual derivative of a function is related to the Radon–Nikodym derivative, or density, of a measure. We have the following chains of inclusions for functions over a compact subset of the real line:
In mathematics, the Radon–Nikodym theorem is a result in measure theory that expresses the relationship between two measures defined on the same measurable space. A measure is a set function that assigns a consistent magnitude to the measurable subsets of a measurable space. Examples of a measure include area and volume, where the subsets are sets of points; or the probability of an event, which is a subset of possible outcomes within a wider probability space.
In the mathematical field of measure theory, an outer measure or exterior measure is a function defined on all subsets of a given set with values in the extended real numbers satisfying some additional technical conditions. The theory of outer measures was first introduced by Constantin Carathéodory to provide an abstract basis for the theory of measurable sets and countably additive measures. Carathéodory's work on outer measures found many applications in measure-theoretic set theory, and was used in an essential way by Hausdorff to define a dimension-like metric invariant now called Hausdorff dimension. Outer measures are commonly used in the field of geometric measure theory.
In mathematics, the ba space of an algebra of sets is the Banach space consisting of all bounded and finitely additive signed measures on . The norm is defined as the variation, that is
In mathematics, the total variation identifies several slightly different concepts, related to the (local or global) structure of the codomain of a function or a measure. For a real-valued continuous function f, defined on an interval [a, b] ⊂ R, its total variation on the interval of definition is a measure of the one-dimensional arclength of the curve with parametric equation x ↦ f(x), for x ∈ [a, b]. Functions whose total variation is finite are called functions of bounded variation.
In mathematics and functional analysis a direct integral or Hilbert integral is a generalization of the concept of direct sum. The theory is most developed for direct integrals of Hilbert spaces and direct integrals of von Neumann algebras. The concept was introduced in 1949 by John von Neumann in one of the papers in the series On Rings of Operators. One of von Neumann's goals in this paper was to reduce the classification of von Neumann algebras on separable Hilbert spaces to the classification of so-called factors. Factors are analogous to full matrix algebras over a field, and von Neumann wanted to prove a continuous analogue of the Artin–Wedderburn theorem classifying semi-simple rings.
In functional analysis, an abelian von Neumann algebra is a von Neumann algebra of operators on a Hilbert space in which all elements commute.
In mathematics, the Hahn decomposition theorem, named after the Austrian mathematician Hans Hahn, states that for any measurable space and any signed measure defined on the -algebra , there exist two -measurable sets, and , of such that:
- and .
- For every such that , one has , i.e., is a positive set for .
- For every such that , one has , i.e., is a negative set for .
In mathematics, specifically measure theory, a complex measure generalizes the concept of measure by letting it have complex values. In other words, one allows for sets whose size is a complex number.
In mathematics, more precisely in measure theory, an atom is a measurable set which has positive measure and contains no set of smaller positive measure. A measure which has no atoms is called non-atomic or atomless.
In measure theory, given a measurable space and a signed measure on it, a set is called a positive set for if every -measurable subset of has nonnegative measure; that is, for every that satisfies holds.
In mathematics, a positive (or signed) measure μ defined on a σ-algebra Σ of subsets of a set X is called a finite measure if μ(X) is a finite real number (rather than ∞). A set A in Σ is of finite measure if μ(A) < ∞. The measure μ is called σ-finite if X is a countable union of measurable sets each with finite measure. A set in a measure space is said to have σ-finite measure if it is a countable union of measurable sets with finite measure. A measure being σ-finite is a weaker condition than being finite, i.e. all finite measures are σ-finite but there are (many) σ-finite measures that are not finite.
In mathematics, more precisely in measure theory, Lebesgue's decomposition theorem states that for every two σ-finite signed measures and on a measurable space there exist two σ-finite signed measures and such that:
In mathematics, a vector measure is a function defined on a family of sets and taking vector values satisfying certain properties. It is a generalization of the concept of finite measure, which takes nonnegative real values only.
In measure theory, a pushforward measure is obtained by transferring a measure from one measurable space to another using a measurable function.
In mathematics, especially measure theory, a set function is a function whose domain is a family of subsets of some given set and that (usually) takes its values in the extended real number line which consists of the real numbers and

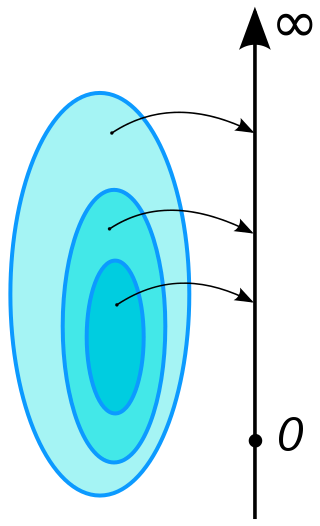
In mathematics, the integral of a non-negative function of a single variable can be regarded, in the simplest case, as the area between the graph of that function and the X axis. The Lebesgue integral, named after French mathematician Henri Lebesgue, extends the integral to a larger class of functions. It also extends the domains on which these functions can be defined.
In mathematics, the Freudenthal spectral theorem is a result in Riesz space theory proved by Hans Freudenthal in 1936. It roughly states that any element dominated by a positive element in a Riesz space with the principal projection property can in a sense be approximated uniformly by simple functions.




















