In mathematics, Pascal's triangle is a triangular array of the binomial coefficients arising in probability theory, combinatorics, and algebra. In much of the Western world, it is named after the French mathematician Blaise Pascal, although other mathematicians studied it centuries before him in Persia, India, China, Germany, and Italy.
2 (two) is a number, numeral and digit. It is the natural number following 1 and preceding 3. It is the smallest and only even prime number. Because it forms the basis of a duality, it has religious and spiritual significance in many cultures.
In combinatorial mathematics, the Bell numbers count the possible partitions of a set. These numbers have been studied by mathematicians since the 19th century, and their roots go back to medieval Japan. In an example of Stigler's law of eponymy, they are named after Eric Temple Bell, who wrote about them in the 1930s.

In mathematics, a partition of a set is a grouping of its elements into non-empty subsets, in such a way that every element is included in exactly one subset.
In mathematics, Euler's pentagonal number theorem relates the product and series representations of the Euler function. It states that

In mathematical set theory, Cantor's theorem is a fundamental result which states that, for any set , the set of all subsets of the power set of has a strictly greater cardinality than itself.
90 (ninety) is the natural number following 89 and preceding 91.

In mathematics, a singleton, also known as a unit set or one-point set, is a set with exactly one element. For example, the set is a singleton whose single element is .
1000 or one thousand is the natural number following 999 and preceding 1001. In most English-speaking countries, it can be written with or without a comma or sometimes a period separating the thousands digit: 1,000.
700 is the natural number following 699 and preceding 701.
The On-Line Encyclopedia of Integer Sequences (OEIS) is an online database of integer sequences. It was created and maintained by Neil Sloane while researching at AT&T Labs. He transferred the intellectual property and hosting of the OEIS to the OEIS Foundation in 2009. Sloane is the chairman of the OEIS Foundation.
126 is the natural number following 125 and preceding 127.

1,000,000,000 is the natural number following 999,999,999 and preceding 1,000,000,001. With a number, "billion" can be abbreviated as b, bil or bn.

In mathematics, particularly in combinatorics, a Stirling number of the second kind is the number of ways to partition a set of n objects into k non-empty subsets and is denoted by or . Stirling numbers of the second kind occur in the field of mathematics called combinatorics and the study of partitions. They are named after James Stirling.
162 is the natural number between 161 and 163.
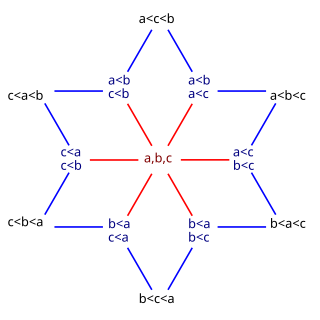
In number theory and enumerative combinatorics, the ordered Bell numbers or Fubini numbers count the number of weak orderings on a set of elements. Weak orderings arrange their elements into a sequence allowing ties, such as might arise as the outcome of a horse race). Starting from , these numbers are
In mathematics, a sequence of natural numbers is called a complete sequence if every positive integer can be expressed as a sum of values in the sequence, using each value at most once.

In mathematics and computing, a triangular array of numbers, polynomials, or the like, is a doubly indexed sequence in which each row is only as long as the row's own index. That is, the ith row contains only i elements.
In mathematics, the fibbinary numbers are the numbers whose binary representation does not contain two consecutive ones. That is, they are sums of distinct and non-consecutive powers of two.
In the number theory of integer partitions, the numbers denote both the number of partitions of into exactly parts, and the number of partitions of into parts of maximum size exactly . These two types of partition are in bijection with each other, by a diagonal reflection of their Young diagrams. Their numbers can be arranged into a triangle, the triangle of partition numbers, in which the th row gives the partition numbers :







