
Georg Ferdinand Ludwig Philipp Cantor was a German mathematician. He played a pivotal role in the creation of set theory, which has become a fundamental theory in mathematics. Cantor established the importance of one-to-one correspondence between the members of two sets, defined infinite and well-ordered sets, and proved that the real numbers are more numerous than the natural numbers. In fact, Cantor's method of proof of this theorem implies the existence of an infinity of infinities. He defined the cardinal and ordinal numbers and their arithmetic. Cantor's work is of great philosophical interest, a fact he was well aware of.
In the philosophy of mathematics, intuitionism, or neointuitionism, is an approach where mathematics is considered to be purely the result of the constructive mental activity of humans rather than the discovery of fundamental principles claimed to exist in an objective reality. That is, logic and mathematics are not considered analytic activities wherein deep properties of objective reality are revealed and applied, but are instead considered the application of internally consistent methods used to realize more complex mental constructs, regardless of their possible independent existence in an objective reality.
In the philosophy of mathematics, the abstraction of actual infinity involves the acceptance of infinite entities as given, actual and completed objects. These might include the set of natural numbers, extended real numbers, transfinite numbers, or even an infinite sequence of rational numbers. Actual infinity is to be contrasted with potential infinity, in which a non-terminating process produces a sequence with no last element, and where each individual result is finite and is achieved in a finite number of steps. As a result, potential infinity is often formalized using the concept of limit.
Finitism is a philosophy of mathematics that accepts the existence only of finite mathematical objects. It is best understood in comparison to the mainstream philosophy of mathematics where infinite mathematical objects are accepted as legitimate.

Foundations of mathematics is the study of the philosophical and logical and/or algorithmic basis of mathematics, or, in a broader sense, the mathematical investigation of what underlies the philosophical theories concerning the nature of mathematics. In this latter sense, the distinction between foundations of mathematics and philosophy of mathematics turns out to be quite vague. Foundations of mathematics can be conceived as the study of the basic mathematical concepts and how they form hierarchies of more complex structures and concepts, especially the fundamentally important structures that form the language of mathematics also called metamathematical concepts, with an eye to the philosophical aspects and the unity of mathematics. The search for foundations of mathematics is a central question of the philosophy of mathematics; the abstract nature of mathematical objects presents special philosophical challenges.
The Royal Society Science Books Prize is an annual £25,000 prize awarded by the Royal Society to celebrate outstanding popular science books from around the world. It is open to authors of science books written for a non-specialist audience, and since it was established in 1988 has championed writers such as Stephen Hawking, Jared Diamond, Stephen Jay Gould and Bill Bryson. In 2015 The Guardian described the prize as "the most prestigious science book prize in Britain".

White Light is a work of science fiction by Rudy Rucker published in 1980 by Virgin Books in the UK and Ace Books in the US. It was written while Rucker was teaching mathematics at the University of Heidelberg from 1978 to 1980, at roughly the same time he was working on the non-fiction book Infinity and the Mind.
The infinity symbol is a mathematical symbol representing the concept of infinity. This symbol is also called a lemniscate, after the lemniscate curves of a similar shape studied in algebraic geometry, or "lazy eight", in the terminology of livestock branding.
In mathematics, 0.999... denotes the repeating decimal consisting of an unending sequence of 9s after the decimal point. This repeating decimal represents the smallest number no less than every decimal number in the sequence ; that is, the supremum of this sequence. This number is equal to 1. In other words, "0.999..." is not "almost exactly" or "very, very nearly but not quite" 1 – rather, "0.999..." and "1" represent exactly the same number.
Popular mathematics is mathematical presentation aimed at a general audience. Sometimes this is in the form of books which require no mathematical background and in other cases it is in the form of expository articles written by professional mathematicians to reach out to others working in different areas.
Divine Proportions: Rational Trigonometry to Universal Geometry is a 2005 book by the mathematician Norman J. Wildberger on a proposed alternative approach to Euclidean geometry and trigonometry, called rational trigonometry. The book advocates replacing the usual basic quantities of trigonometry, Euclidean distance and angle measure, by squared distance and the square of the sine of the angle, respectively. This is logically equivalent to the standard development. The author claims his approach holds some advantages, such as avoiding the need for irrational numbers.
In philosophy and theology, infinity is explored in articles under headings such as the Absolute, God, and Zeno's paradoxes.
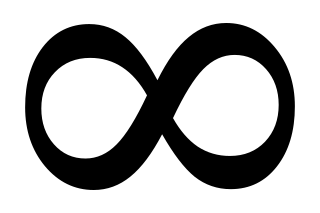
Infinity is that which is boundless, endless, or larger than any natural number. It is often denoted by the infinity symbol .

Adrian William Moore is a British philosopher and broadcaster. He is Professor of Philosophy at the University of Oxford and tutorial fellow of St Hugh's College, Oxford. His main areas of interest are Kant, Wittgenstein, history of philosophy, metaphysics, philosophy of mathematics, philosophy of logic and language, ethics and philosophy of religion.

Eugenia Loh-Gene Cheng is a British mathematician and concert pianist. Her mathematical interests include higher category theory, and as a pianist she specialises in lieder and art song. She is also passionate about explaining mathematics to non-mathematicians to rid the world of math phobia, often using entertaining analogies with food and baking. Cheng is a scientist-in-residence at the School of the Art Institute of Chicago.
In logic, an infinite-valued logic is a many-valued logic in which truth values comprise a continuous range. Traditionally, in Aristotle's logic, logic other than bivalent logic was abnormal, as the law of the excluded middle precluded more than two possible values for any proposition. Modern three-valued logic allows for an additional possible truth value and is an example of finite-valued logic in which truth values are discrete, rather than continuous. Infinite-valued logic comprises continuous fuzzy logic, though fuzzy logic in some of its forms can further encompass finite-valued logic. For example, finite-valued logic can be applied in Boolean-valued modeling, description logics, and defuzzification of fuzzy logic.

In mathematics, division by infinity is division where the divisor (denominator) is infinity. In ordinary arithmetic, this does not have a well-defined meaning, since infinity is a mathematical concept that does not correspond to a specific number, and moreover, there is no nonzero real number that, when added to itself an infinite number of times, gives a finite number. However, "dividing by infinity" can be given meaning as an informal way of expressing the limit of dividing a number by larger and larger divisors.

Closing the Gap: The Quest to Understand Prime Numbers is a book on prime numbers and prime gaps by Vicky Neale, published in 2017 by the Oxford University Press (ISBN 9780198788287). The Basic Library List Committee of the Mathematical Association of America has suggested that it be included in undergraduate mathematics libraries.
Playing with Infinity: Mathematical Explorations and Excursions is a book in popular mathematics by Hungarian mathematician Rózsa Péter, published in German in 1955 and in English in 1961.
Beyond Infinity may refer to:








