Related Research Articles

In complex analysis, the Riemann mapping theorem states that if U is a non-empty simply connected open subset of the complex number plane C which is not all of C, then there exists a biholomorphic mapping f from U onto the open unit disk

In mathematics, a conformal map is a function that locally preserves angles, but not necessarily lengths.

Lars Valerian Ahlfors was a Finnish mathematician, remembered for his work in the field of Riemann surfaces and his text on complex analysis.

In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed versions of the complex plane: locally near every point they look like patches of the complex plane, but the global topology can be quite different. For example, they can look like a sphere or a torus or several sheets glued together.
In mathematics, the uniformization theorem says that every simply connected Riemann surface is conformally equivalent to one of three Riemann surfaces: the open unit disk, the complex plane, or the Riemann sphere. The theorem is a generalization of the Riemann mapping theorem from simply connected open subsets of the plane to arbitrary simply connected Riemann surfaces.

Dennis Parnell Sullivan is an American mathematician known for his work in algebraic topology, geometric topology, and dynamical systems. He holds the Albert Einstein Chair at the City University of New York Graduate Center and is a distinguished professor at Stony Brook University.
In mathematics, the Schwarz alternating method or alternating process is an iterative method introduced in 1869–1870 by Hermann Schwarz in the theory of conformal mapping. Given two overlapping regions in the complex plane in each of which the Dirichlet problem could be solved, Schwarz described an iterative method for solving the Dirichlet problem in their union, provided their intersection was suitably well behaved. This was one of several constructive techniques of conformal mapping developed by Schwarz as a contribution to the problem of uniformization, posed by Riemann in the 1850s and first resolved rigorously by Koebe and Poincaré in 1907. It furnished a scheme for uniformizing the union of two regions knowing how to uniformize each of them separately, provided their intersection was topologically a disk or an annulus. From 1870 onwards Carl Neumann also contributed to this theory.
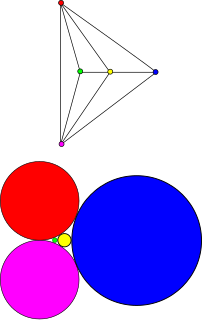
The circle packing theorem describes the possible tangency relations between circles in the plane whose interiors are disjoint. A circle packing is a connected collection of circles whose interiors are disjoint. The intersection graph of a circle packing is the graph having a vertex for each circle, and an edge for every pair of circles that are tangent. If the circle packing is on the plane, or, equivalently, on the sphere, then its intersection graph is called a coin graph; more generally, intersection graphs of interior-disjoint geometric objects are called tangency graphs or contact graphs. Coin graphs are always connected, simple, and planar. The circle packing theorem states that these are the only requirements for a graph to be a coin graph:
Stefan Emanuel "Steve" Warschawski was a mathematician, a professor and department chair at the University of Minnesota and the founder of the mathematics department at the University of California, San Diego.
Theodore Frankel was a mathematician who introduced the Andreotti–Frankel theorem and the Frankel conjecture.
Carl David Minda is an American mathematician, the Charles Phelps Taft Professor of Mathematics at the University of Cincinnati.
Leo Reino Sario was a Finnish-born mathematician who worked on complex analysis and Riemann surfaces.
Paul C. Yang is a Taiwanese-American mathematician specializing in differential geometry, partial differential equations and CR manifolds. He is best known for his work in Conformal geometry for his study of extremal metrics and his research on scalar curvature and Q-curvature. In CR Geometry he is known for his work on the CR embedding problem, the CR Paneitz operator and for introducing the Q' curvature in CR Geometry.
Clifford John Earle, Jr. was an American mathematician who specialized in complex variables and Teichmüller spaces.
Ravindra Shripad Kulkarni is an Indian mathematician, specializing in differential geometry. He is known for the Kulkarni–Nomizu product.
Maurice Haskell Heins was an American mathematician, specializing in complex analysis and harmonic analysis.
Albert Marden is an American mathematician, specializing in complex analysis and hyperbolic geometry.
Introduction to Circle Packing: The Theory of Discrete Analytic Functions is a mathematical monograph concerning systems of tangent circles and the circle packing theorem. It was written by Kenneth Stephenson and published in 2005 by the Cambridge University Press.

In the geometry of circle packings in the Euclidean plane, the ring lemma gives a lower bound on the sizes of adjacent circles in a circle packing.
References
- ↑ "Burton Rodin - The Mathematics Genealogy Project". www.genealogy.ams.org.
- ↑ "Website for systolic geometry and topology". www.cs.biu.ac.il.
- ↑ The method of extremal length: invited hour address presented at the 705th meeting of the American Mathematical Society. Bull. Amer. Math. Soc. 80, 1974, 587–606
- ↑ B. Rodin and S. E. Warschawski, “On the derivative of the Riemann mapping function near a boundary point and the Visser-Ostrowski problem”, Mathematische Annalen, 248, (1980), 125–137.
- ↑ B. Rodin and D. Sullivan, “The convergence of circle packings to the Riemann mapping”, Journal of Differential Geometry, 26 (1987), 349–360.
- ↑ List of Fellows of the American Mathematical Society, retrieved 2013-01-27.