
A gluon is a type of massless elementary particle that mediates the strong interaction between quarks, acting as the exchange particle for the interaction. Gluons are massless vector bosons, thereby having a spin of 1. Through the strong interaction, gluons bind quarks into groups according to quantum chromodynamics (QCD), forming hadrons such as protons and neutrons.

In particle physics, a hadron is a composite subatomic particle made of two or more quarks held together by the strong interaction. They are analogous to molecules, which are held together by the electric force. Most of the mass of ordinary matter comes from two hadrons: the proton and the neutron, while most of the mass of the protons and neutrons is in turn due to the binding energy of their constituent quarks, due to the strong force.
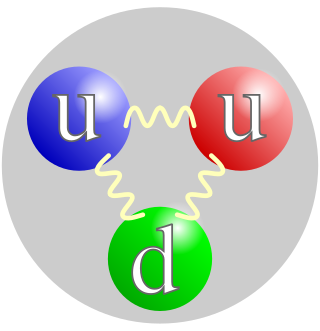
A proton is a stable subatomic particle, symbol
p
, H+, or 1H+ with a positive electric charge of +1 e (elementary charge). Its mass is slightly less than the mass of a neutron and approximately 1836 times the mass of an electron (the proton-to-electron mass ratio). Protons and neutrons, each with a mass of approximately one atomic mass unit, are jointly referred to as nucleons (particles present in atomic nuclei).

In theoretical physics, quantum chromodynamics (QCD) is the study of the strong interaction between quarks mediated by gluons. Quarks are fundamental particles that make up composite hadrons such as the proton, neutron and pion. QCD is a type of quantum field theory called a non-abelian gauge theory, with symmetry group SU(3). The QCD analog of electric charge is a property called color. Gluons are the force carriers of the theory, just as photons are for the electromagnetic force in quantum electrodynamics. The theory is an important part of the Standard Model of particle physics. A large body of experimental evidence for QCD has been gathered over the years.

The Standard Model of particle physics is the theory describing three of the four known fundamental forces in the universe and classifying all known elementary particles. It was developed in stages throughout the latter half of the 20th century, through the work of many scientists worldwide, with the current formulation being finalized in the mid-1970s upon experimental confirmation of the existence of quarks. Since then, proof of the top quark (1995), the tau neutrino (2000), and the Higgs boson (2012) have added further credence to the Standard Model. In addition, the Standard Model has predicted various properties of weak neutral currents and the W and Z bosons with great accuracy.
Hadronization is the process of the formation of hadrons out of quarks and gluons. There are two main branches of hadronization: quark-gluon plasma (QGP) transformation and colour string decay into hadrons. The transformation of quark-gluon plasma into hadrons is studied in lattice QCD numerical simulations, which are explored in relativistic heavy-ion experiments. Quark-gluon plasma hadronization occurred shortly after the Big Bang when the quark–gluon plasma cooled down to the Hagedorn temperature when free quarks and gluons cannot exist. In string breaking new hadrons are forming out of quarks, antiquarks and sometimes gluons, spontaneously created from the vacuum.

In particle physics, quarkonium is a flavorless meson whose constituents are a heavy quark and its own antiquark, making it both a neutral particle and its own antiparticle. The name "quarkonium" is analogous to positronium, the bound state of electron and anti-electron. The particles are short-lived due to matter-antimatter annihilation.
Quark matter or QCD matter refers to any of a number of hypothetical phases of matter whose degrees of freedom include quarks and gluons, of which the prominent example is quark-gluon plasma. Several series of conferences in 2019, 2020, and 2021 were devoted to this topic.
The QCD vacuum is the quantum vacuum state of quantum chromodynamics (QCD). It is an example of a non-perturbative vacuum state, characterized by non-vanishing condensates such as the gluon condensate and the quark condensate in the complete theory which includes quarks. The presence of these condensates characterizes the confined phase of quark matter.

Exotic hadrons are subatomic particles composed of quarks and gluons, but which – unlike "well-known" hadrons such as protons, neutrons and mesons – consist of more than three valence quarks. By contrast, "ordinary" hadrons contain just two or three quarks. Hadrons with explicit valence gluon content would also be considered exotic. In theory, there is no limit on the number of quarks in a hadron, as long as the hadron's color charge is white, or color-neutral.
The nuclear force is a force that acts between hadrons, most commonly observed between protons and neutrons of atoms. Neutrons and protons, both nucleons, are affected by the nuclear force almost identically. Since protons have charge +1 e, they experience an electric force that tends to push them apart, but at short range the attractive nuclear force is strong enough to overcome the electrostatic force. The nuclear force binds nucleons into atomic nuclei.
In particle physics, a diquark, or diquark correlation/clustering, is a hypothetical state of two quarks grouped inside a baryon. Corresponding models of baryons are referred to as quark–diquark models. The diquark is often treated as a single subatomic particle with which the third quark interacts via the strong interaction. The existence of diquarks inside the nucleons is a disputed issue, but it helps to explain some nucleon properties and to reproduce experimental data sensitive to the nucleon structure. Diquark–antidiquark pairs have also been advanced for anomalous particles such as the X(3872).
In particle physics, the parton model is a model of hadrons, such as protons and neutrons, proposed by Richard Feynman. It is useful for interpreting the cascades of radiation produced from quantum chromodynamics (QCD) processes and interactions in high-energy particle collisions.

Quark–gluon plasma is an interacting localized assembly of quarks and gluons at thermal and chemical (abundance) equilibrium. The word plasma signals that free color charges are allowed. In a 1987 summary, Léon Van Hove pointed out the equivalence of the three terms: quark gluon plasma, quark matter and a new state of matter. Since the temperature is above the Hagedorn temperature—and thus above the scale of light u,d-quark mass—the pressure exhibits the relativistic Stefan-Boltzmann format governed by temperature to the fourth power and many practically massless quark and gluon constituents. It can be said that QGP emerges to be the new phase of strongly interacting matter which manifests its physical properties in terms of nearly free dynamics of practically massless gluons and quarks. Both quarks and gluons must be present in conditions near chemical (yield) equilibrium with their colour charge open for a new state of matter to be referred to as QGP.
In high-energy nuclear physics, strangeness production in relativistic heavy-ion collisions is a signature and diagnostic tool of quark–gluon plasma (QGP) formation and properties. Unlike up and down quarks, from which everyday matter is made, heavier quark flavors such as strange and charm typically approach chemical equilibrium in a dynamic evolution process. QGP is an interacting localized assembly of quarks and gluons at thermal (kinetic) and not necessarily chemical (abundance) equilibrium. The word plasma signals that color charged particles are able to move in the volume occupied by the plasma. The abundance of strange quarks is formed in pair-production processes in collisions between constituents of the plasma, creating the chemical abundance equilibrium. The dominant mechanism of production involves gluons only present when matter has become a quark–gluon plasma. When quark–gluon plasma disassembles into hadrons in a breakup process, the high availability of strange antiquarks helps to produce antimatter containing multiple strange quarks, which is otherwise rarely made. Similar considerations are at present made for the heavier charm flavor, which is made at the beginning of the collision process in the first interactions and is only abundant in the high-energy environments of CERN's Large Hadron Collider.

The NA49 experiment was a particle physics experiment that investigated the properties of quark–gluon plasma. The experiment's synonym was Ions/TPC-Hadrons. It took place in the North Area of the Super Proton Synchrotron (SPS) at CERN from 1991-2002.

In strong interaction physics, light front holography or light front holographic QCD is an approximate version of the theory of quantum chromodynamics (QCD) which results from mapping the gauge theory of QCD to a higher-dimensional anti-de Sitter space (AdS) inspired by the AdS/CFT correspondence proposed for string theory. This procedure makes it possible to find analytic solutions in situations where strong coupling occurs, improving predictions of the masses of hadrons and their internal structure revealed by high-energy accelerator experiments. The most widely used approach to finding approximate solutions to the QCD equations, lattice QCD, has had many successful applications; however, it is a numerical approach formulated in Euclidean space rather than physical Minkowski space-time.

The light-front quantization of quantum field theories provides a useful alternative to ordinary equal-time quantization. In particular, it can lead to a relativistic description of bound systems in terms of quantum-mechanical wave functions. The quantization is based on the choice of light-front coordinates, where plays the role of time and the corresponding spatial coordinate is . Here, is the ordinary time, is a Cartesian coordinate, and is the speed of light. The other two Cartesian coordinates, and , are untouched and often called transverse or perpendicular, denoted by symbols of the type . The choice of the frame of reference where the time and -axis are defined can be left unspecified in an exactly soluble relativistic theory, but in practical calculations some choices may be more suitable than others. The basic formalism is discussed elsewhere.

In nuclear physics, ab initio methods seek to describe the atomic nucleus from the bottom up by solving the non-relativistic Schrödinger equation for all constituent nucleons and the forces between them. This is done either exactly for very light nuclei or by employing certain well-controlled approximations for heavier nuclei. Ab initio methods constitute a more fundamental approach compared to e.g. the nuclear shell model. Recent progress has enabled ab initio treatment of heavier nuclei such as nickel.
In quantum field theory, a sum rule is a relation between a static quantity and an integral over a dynamical quantity. Therefore, they have a form such as:



















