
In mathematics, genus has a few different, but closely related, meanings. Intuitively, the genus is the number of "holes" of a surface. A sphere has genus 0, while a torus has genus 1.
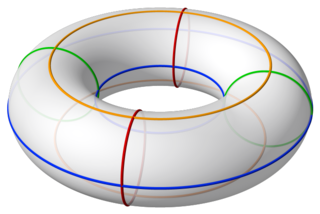
In geometry, a torus is a surface of revolution generated by revolving a circle in three-dimensional space one full revolution about an axis that is coplanar with the circle. The main types of toruses include ring toruses, horn toruses, and spindle toruses. A ring torus is sometimes colloquially referred to as a donut or doughnut.

In mathematics, a minimal surface is a surface that locally minimizes its area. This is equivalent to having zero mean curvature.

In differential geometry, the Gaussian curvature or Gauss curvatureΚ of a smooth surface in three-dimensional space at a point is the product of the principal curvatures, κ1 and κ2, at the given point:
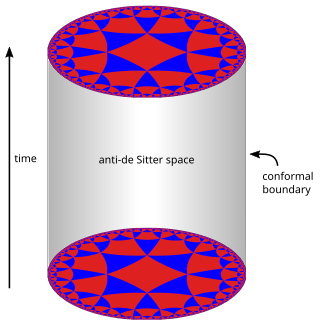
In mathematics and physics, n-dimensional anti-de Sitter space (AdSn) is a maximally symmetric Lorentzian manifold with constant negative scalar curvature. Anti-de Sitter space and de Sitter space are named after Willem de Sitter (1872–1934), professor of astronomy at Leiden University and director of the Leiden Observatory. Willem de Sitter and Albert Einstein worked together closely in Leiden in the 1920s on the spacetime structure of the universe. Paul Dirac was the first person to rigorously explore anti-de Sitter space, doing so in 1963.

The great-circle distance, orthodromic distance, or spherical distance is the distance along a great circle.
In mathematics, the mean curvature of a surface is an extrinsic measure of curvature that comes from differential geometry and that locally describes the curvature of an embedded surface in some ambient space such as Euclidean space.
In mathematics, a Killing vector field, named after Wilhelm Killing, is a vector field on a Riemannian manifold that preserves the metric. Killing fields are the infinitesimal generators of isometries; that is, flows generated by Killing fields are continuous isometries of the manifold. More simply, the flow generates a symmetry, in the sense that moving each point of an object the same distance in the direction of the Killing vector will not distort distances on the object.
In mathematics, the Thom space,Thom complex, or Pontryagin–Thom construction of algebraic topology and differential topology is a topological space associated to a vector bundle, over any paracompact space.
In differential geometry, the Laplace–Beltrami operator is a generalization of the Laplace operator to functions defined on submanifolds in Euclidean space and, even more generally, on Riemannian and pseudo-Riemannian manifolds. It is named after Pierre-Simon Laplace and Eugenio Beltrami.
In mathematics, an isotropic manifold is a manifold in which the geometry does not depend on directions. Formally, we say that a Riemannian manifold is isotropic if for any point and unit vectors , there is an isometry of with and . Every connected isotropic manifold is homogeneous, i.e. for any there is an isometry of with This can be seen by considering a geodesic :[0,2]\to M} from to and taking the isometry which fixes and maps to

Curved space often refers to a spatial geometry which is not "flat", where a flat space has zero curvature, as described by Euclidean geometry. Curved spaces can generally be described by Riemannian geometry, though some simple cases can be described in other ways. Curved spaces play an essential role in general relativity, where gravity is often visualized as curved space. The Friedmann–Lemaître–Robertson–Walker metric is a curved metric which forms the current foundation for the description of the expansion of space and shape of the universe. The fact that photons have no mass yet are distorted by gravity, means that the explanation would have to be something besides photonic mass. Hence, the belief that large bodies curve space and so light, traveling on the curved space will, appear as being subject to gravity. It is not, but it is subject to the curvature of space.
A parametric surface is a surface in the Euclidean space which is defined by a parametric equation with two parameters :\mathbb {R} ^{2}\to \mathbb {R} ^{3}} . Parametric representation is a very general way to specify a surface, as well as implicit representation. Surfaces that occur in two of the main theorems of vector calculus, Stokes' theorem and the divergence theorem, are frequently given in a parametric form. The curvature and arc length of curves on the surface, surface area, differential geometric invariants such as the first and second fundamental forms, Gaussian, mean, and principal curvatures can all be computed from a given parametrization.
In the field of differential geometry in mathematics, mean curvature flow is an example of a geometric flow of hypersurfaces in a Riemannian manifold. Intuitively, a family of surfaces evolves under mean curvature flow if the normal component of the velocity of which a point on the surface moves is given by the mean curvature of the surface. For example, a round sphere evolves under mean curvature flow by shrinking inward uniformly. Except in special cases, the mean curvature flow develops singularities.

In mathematics, the differential geometry of surfaces deals with the differential geometry of smooth surfaces with various additional structures, most often, a Riemannian metric. Surfaces have been extensively studied from various perspectives: extrinsically, relating to their embedding in Euclidean space and intrinsically, reflecting their properties determined solely by the distance within the surface as measured along curves on the surface. One of the fundamental concepts investigated is the Gaussian curvature, first studied in depth by Carl Friedrich Gauss, who showed that curvature was an intrinsic property of a surface, independent of its isometric embedding in Euclidean space.

Geographical distance or geodetic distance is the distance measured along the surface of the Earth, or the shortest arch length.
In mathematics, the Thurston boundary of Teichmüller space of a surface is obtained as the boundary of its closure in the projective space of functionals on simple closed curves on the surface. The Thurston boundary can be interpreted as the space of projective measured foliations on the surface.
In mathematics, a harmonic morphism is a (smooth) map :(M^{m},g)\to (N^{n},h)} between Riemannian manifolds that pulls back real-valued harmonic functions on the codomain to harmonic functions on the domain. Harmonic morphisms form a special class of harmonic maps i.e. those that are horizontally (weakly) conformal.
Arithmetic Fuchsian groups are a special class of Fuchsian groups constructed using orders in quaternion algebras. They are particular instances of arithmetic groups. The prototypical example of an arithmetic Fuchsian group is the modular group . They, and the hyperbolic surface associated to their action on the hyperbolic plane often exhibit particularly regular behaviour among Fuchsian groups and hyperbolic surfaces.
In geometry, a valuation is a finitely additive function from a collection of subsets of a set to an abelian semigroup. For example, Lebesgue measure is a valuation on finite unions of convex bodies of Other examples of valuations on finite unions of convex bodies of are surface area, mean width, and Euler characteristic.

















































