
In the mathematical field of graph theory, the Petersen graph is an undirected graph with 10 vertices and 15 edges. It is a small graph that serves as a useful example and counterexample for many problems in graph theory. The Petersen graph is named after Julius Petersen, who in 1898 constructed it to be the smallest bridgeless cubic graph with no three-edge-coloring.
10 (ten) is the even natural number following 9 and preceding 11. Ten is the base of the decimal numeral system, the most common system of denoting numbers in both spoken and written language.
104 is the natural number following 103 and preceding 105.
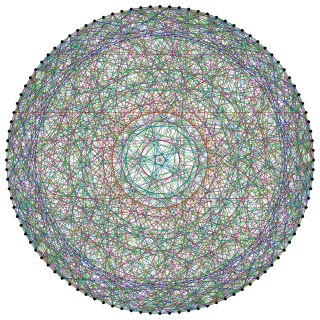
In mathematical graph theory, the Higman–Sims graph is a 22-regular undirected graph with 100 vertices and 1100 edges. It is the unique strongly regular graph srg(100,22,0,6), where no neighboring pair of vertices share a common neighbor and each non-neighboring pair of vertices share six common neighbors. It was first constructed by Mesner (1956) and rediscovered in 1968 by Donald G. Higman and Charles C. Sims as a way to define the Higman–Sims group, a subgroup of index two in the group of automorphisms of the Higman–Sims graph.
In chemistry, water(s) of crystallization or water(s) of hydration are water molecules that are present inside crystals. Water is often incorporated in the formation of crystals from aqueous solutions. In some contexts, water of crystallization is the total mass of water in a substance at a given temperature and is mostly present in a definite (stoichiometric) ratio. Classically, "water of crystallization" refers to water that is found in the crystalline framework of a metal complex or a salt, which is not directly bonded to the metal cation.

In graph theory, the degree of a vertex of a graph is the number of edges that are incident to the vertex; in a multigraph, a loop contributes 2 to a vertex's degree, for the two ends of the edge. The degree of a vertex is denoted or . The maximum degree of a graph is denoted by , and is the maximum of 's vertices' degrees. The minimum degree of a graph is denoted by , and is the minimum of 's vertices' degrees. In the multigraph shown on the right, the maximum degree is 5 and the minimum degree is 0.

A tournament is a directed graph (digraph) obtained by assigning a direction for each edge in an undirected complete graph. That is, it is an orientation of a complete graph, or equivalently a directed graph in which every pair of distinct vertices is connected by a directed edge with any one of the two possible orientations.

The chromatic polynomial is a graph polynomial studied in algebraic graph theory, a branch of mathematics. It counts the number of graph colorings as a function of the number of colors and was originally defined by George David Birkhoff to study the four color problem. It was generalised to the Tutte polynomial by Hassler Whitney and W. T. Tutte, linking it to the Potts model of statistical physics.

Euclidean plane tilings by convex regular polygons have been widely used since antiquity. The first systematic mathematical treatment was that of Kepler in his Harmonices Mundi.
5 (five) is a number, numeral and digit. It is the natural number, and cardinal number, following 4 and preceding 6, and is a prime number. It has garnered attention throughout history in part because distal extremities in humans typically contain five digits.
Polyhedral combinatorics is a branch of mathematics, within combinatorics and discrete geometry, that studies the problems of counting and describing the faces of convex polyhedra and higher-dimensional convex polytopes.
In polyhedral combinatorics, a branch of mathematics, Steinitz's theorem is a characterization of the undirected graphs formed by the edges and vertices of three-dimensional convex polyhedra: they are exactly the 3-vertex-connected planar graphs. That is, every convex polyhedron forms a 3-connected planar graph, and every 3-connected planar graph can be represented as the graph of a convex polyhedron. For this reason, the 3-connected planar graphs are also known as polyhedral graphs.

The Abelian sandpile model (ASM) is the more popular name of the original Bak–Tang–Wiesenfeld model (BTW). The BTW model was the first discovered example of a dynamical system displaying self-organized criticality. It was introduced by Per Bak, Chao Tang and Kurt Wiesenfeld in a 1987 paper.

In the mathematical field of graph theory, the McGee graph or the (3-7)-cage is a 3-regular graph with 24 vertices and 36 edges.
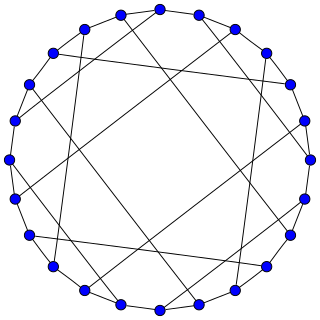
In the mathematical field of graph theory, the Nauru graph is a symmetric, bipartite, cubic graph with 24 vertices and 36 edges. It was named by David Eppstein after the twelve-pointed star in the flag of Nauru.
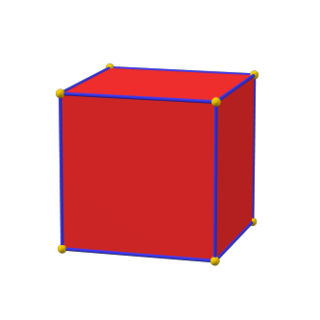
In geometry and polyhedral combinatorics, an integral polytope is a convex polytope whose vertices all have integer Cartesian coordinates. That is, it is a polytope that equals the convex hull of its integer points. Integral polytopes are also called lattice polytopes or Z-polytopes. The special cases of two- and three-dimensional integral polytopes may be called polygons or polyhedra instead of polytopes, respectively.
A Euclidean graph is periodic if there exists a basis of that Euclidean space whose corresponding translations induce symmetries of that graph. Equivalently, a periodic Euclidean graph is a periodic realization of an abelian covering graph over a finite graph. A Euclidean graph is uniformly discrete if there is a minimal distance between any two vertices. Periodic graphs are closely related to tessellations of space and the geometry of their symmetry groups, hence to geometric group theory, as well as to discrete geometry and the theory of polytopes, and similar areas.
In graph theory, a partial cube is a graph that is isometric to a subgraph of a hypercube. In other words, a partial cube can be identified with a subgraph of a hypercube in such a way that the distance between any two vertices in the partial cube is the same as the distance between those vertices in the hypercube. Equivalently, a partial cube is a graph whose vertices can be labeled with bit strings of equal length in such a way that the distance between two vertices in the graph is equal to the Hamming distance between their labels. Such a labeling is called a Hamming labeling; it represents an isometric embedding of the partial cube into a hypercube.

In graph theory, an acyclic orientation of an undirected graph is an assignment of a direction to each edge that does not form any directed cycle and therefore makes it into a directed acyclic graph. Every graph has an acyclic orientation.
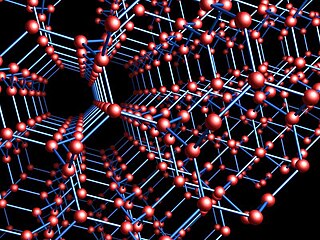
In geometry and crystallography, the Laves graph is an infinite and highly symmetric system of points and line segments in three-dimensional Euclidean space, forming a periodic graph. Three equal-length segments meet at 120° angles at each point, and all cycles use ten or more segments. It is the shortest possible triply periodic graph, relative to the volume of its fundamental domain. One arrangement of the Laves graph uses one out of every eight of the points in the integer lattice as its points, and connects all pairs of these points that are nearest neighbors, at distance . It can also be defined, divorced from its geometry, as an abstract undirected graph, a covering graph of the complete graph on four vertices.



















