Definition
Let denote the inner product on an inner product space and let be a nonempty subset of . A correspondence is called cyclically monotone if for every set of points with it holds that [3]
In mathematics, cyclical monotonicity is a generalization of the notion of monotonicity to the case of vector-valued function. [1] [2]
Let denote the inner product on an inner product space and let be a nonempty subset of . A correspondence is called cyclically monotone if for every set of points with it holds that [3]
For the case of scalar functions of one variable the definition above is equivalent to usual monotonicity. Gradients of convex functions are cyclically monotone. In fact, the converse is true. [4] Suppose is convex and is a correspondence with nonempty values. Then if is cyclically monotone, there exists an upper semicontinuous convex function such that for every , where denotes the subgradient of at . [5]
In mathematics, more specifically in functional analysis, a Banach space is a complete normed vector space. Thus, a Banach space is a vector space with a metric that allows the computation of vector length and distance between vectors and is complete in the sense that a Cauchy sequence of vectors always converges to a well-defined limit that is within the space.
The Riesz representation theorem, sometimes called the Riesz–Fréchet representation theorem after Frigyes Riesz and Maurice René Fréchet, establishes an important connection between a Hilbert space and its continuous dual space. If the underlying field is the real numbers, the two are isometrically isomorphic; if the underlying field is the complex numbers, the two are isometrically anti-isomorphic. The (anti-) isomorphism is a particular natural isomorphism.
In mathematics, weak topology is an alternative term for certain initial topologies, often on topological vector spaces or spaces of linear operators, for instance on a Hilbert space. The term is most commonly used for the initial topology of a topological vector space with respect to its continuous dual. The remainder of this article will deal with this case, which is one of the concepts of functional analysis.
Distributions, also known as Schwartz distributions or generalized functions, are objects that generalize the classical notion of functions in mathematical analysis. Distributions make it possible to differentiate functions whose derivatives do not exist in the classical sense. In particular, any locally integrable function has a distributional derivative.
In mathematics, a linear form is a linear map from a vector space to its field of scalars.

In mathematics, the Legendre transformation, first introduced by Adrien-Marie Legendre in 1787 when studying the minimal surface problem, is an involutive transformation on real-valued functions that are convex on a real variable. Specifically, if a real-valued multivariable function is convex on one of its independent real variables, then the Legendre transform with respect to this variable is applicable to the function.
In functional analysis and related branches of mathematics, the Banach–Alaoglu theorem states that the closed unit ball of the dual space of a normed vector space is compact in the weak* topology. A common proof identifies the unit ball with the weak-* topology as a closed subset of a product of compact sets with the product topology. As a consequence of Tychonoff's theorem, this product, and hence the unit ball within, is compact.
Multi-task learning (MTL) is a subfield of machine learning in which multiple learning tasks are solved at the same time, while exploiting commonalities and differences across tasks. This can result in improved learning efficiency and prediction accuracy for the task-specific models, when compared to training the models separately. Inherently, Multi-task learning is a multi-objective optimization problem having trade-offs between different tasks. Early versions of MTL were called "hints".
In linear algebra, the Gram matrix of a set of vectors in an inner product space is the Hermitian matrix of inner products, whose entries are given by the inner product . If the vectors are the columns of matrix then the Gram matrix is in the general case that the vector coordinates are complex numbers, which simplifies to for the case that the vector coordinates are real numbers.
In mathematics and mathematical optimization, the convex conjugate of a function is a generalization of the Legendre transformation which applies to non-convex functions. It is also known as Legendre–Fenchel transformation, Fenchel transformation, or Fenchel conjugate. The convex conjugate is widely used for constructing the dual problem in optimization theory, thus generalizing Lagrangian duality.
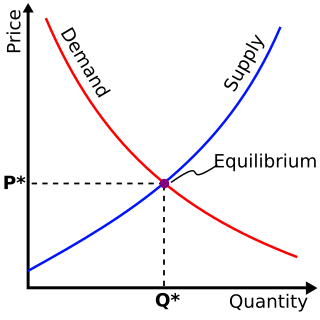
In mathematical economics, the Arrow–Debreu model is a theoretical general equilibrium model. It posits that under certain economic assumptions there must be a set of prices such that aggregate supplies will equal aggregate demands for every commodity in the economy.
In functional and convex analysis, and related disciplines of mathematics, the polar set is a special convex set associated to any subset of a vector space lying in the dual space The bipolar of a subset is the polar of but lies in .
Convex analysis is the branch of mathematics devoted to the study of properties of convex functions and convex sets, often with applications in convex minimization, a subdomain of optimization theory.

In geometry, the hyperplane separation theorem is a theorem about disjoint convex sets in n-dimensional Euclidean space. There are several rather similar versions. In one version of the theorem, if both these sets are closed and at least one of them is compact, then there is a hyperplane in between them and even two parallel hyperplanes in between them separated by a gap. In another version, if both disjoint convex sets are open, then there is a hyperplane in between them, but not necessarily any gap. An axis which is orthogonal to a separating hyperplane is a separating axis, because the orthogonal projections of the convex bodies onto the axis are disjoint.
A self-concordant function is a function satisfying a certain differential inequality, which makes it particularly easy for optimization using Newton's method A self-concordant barrier is a particular self-concordant function, that is also a barrier function for a particular convex set. Self-concordant barriers are important ingredients in interior point methods for optimization.
In mathematics, the Fortuin–Kasteleyn–Ginibre (FKG) inequality is a correlation inequality, a fundamental tool in statistical mechanics and probabilistic combinatorics, due to Cees M. Fortuin, Pieter W. Kasteleyn, and Jean Ginibre. Informally, it says that in many random systems, increasing events are positively correlated, while an increasing and a decreasing event are negatively correlated. It was obtained by studying the random cluster model.
A sum-of-squares optimization program is an optimization problem with a linear cost function and a particular type of constraint on the decision variables. These constraints are of the form that when the decision variables are used as coefficients in certain polynomials, those polynomials should have the polynomial SOS property. When fixing the maximum degree of the polynomials involved, sum-of-squares optimization is also known as the Lasserre hierarchy of relaxations in semidefinite programming.
In mathematics, a dual system, dual pair or a duality over a field is a triple consisting of two vector spaces, and , over and a non-degenerate bilinear map .
This is a glossary for the terminology in a mathematical field of functional analysis.
In computable analysis, Weihrauch reducibility is a notion of reducibility between multi-valued functions on represented spaces that roughly captures the uniform computational strength of computational problems. It was originally introduced by Klaus Weihrauch in an unpublished 1992 technical report.
{{cite book}}: CS1 maint: location missing publisher (link) CS1 maint: multiple names: authors list (link) CS1 maint: numeric names: authors list (link)[ page needed ]