
Risk management is the identification, evaluation, and prioritization of risks, followed by the minimization, monitoring, and control of the impact or probability of those risks occurring. Risks can come from various sources including uncertainty in international markets, political instability, dangers of project failures, legal liabilities, credit risk, accidents, natural causes and disasters, deliberate attack from an adversary, or events of uncertain or unpredictable root-cause.

Monte Carlo methods, or Monte Carlo experiments, are a broad class of computational algorithms that rely on repeated random sampling to obtain numerical results. The underlying concept is to use randomness to solve problems that might be deterministic in principle. The name comes from the Monte Carlo Casino in Monaco, where the primary developer of the method, mathematician Stanisław Ulam, was inspired by his uncle's gambling habits.
Critical chain project management (CCPM) is a method of planning and managing projects that emphasizes the resources required to execute project tasks. It was developed by Eliyahu M. Goldratt. It differs from more traditional methods that derive from critical path and PERT algorithms, which emphasize task order and rigid scheduling. A critical chain project network strives to keep resources levelled, and requires that they be flexible in start times.

The program evaluation and review technique (PERT) is a statistical tool used in project management, which was designed to analyze and represent the tasks involved in completing a given project.

A Gantt chart is a bar chart that illustrates a project schedule. It was designed and popularized by Henry Gantt around the years 1910–1915. Modern Gantt charts also show the dependency relationships between activities and the current schedule status.
In statistics, Markov chain Monte Carlo (MCMC) is a class of algorithms used to draw samples from a probability distribution. Given a probability distribution, one can construct a Markov chain whose elements' distribution approximates it – that is, the Markov chain's equilibrium distribution matches the target distribution. The more steps that are included, the more closely the distribution of the sample matches the actual desired distribution.
Monte Carlo methods are used in corporate finance and mathematical finance to value and analyze (complex) instruments, portfolios and investments by simulating the various sources of uncertainty affecting their value, and then determining the distribution of their value over the range of resultant outcomes. This is usually done by help of stochastic asset models. The advantage of Monte Carlo methods over other techniques increases as the dimensions of the problem increase.
In mathematical finance, a Monte Carlo option model uses Monte Carlo methods to calculate the value of an option with multiple sources of uncertainty or with complicated features. The first application to option pricing was by Phelim Boyle in 1977. In 1996, M. Broadie and P. Glasserman showed how to price Asian options by Monte Carlo. An important development was the introduction in 1996 by Carriere of Monte Carlo methods for options with early exercise features.
Graphical Evaluation and Review Technique (GERT) is a network analysis technique used in project management that allows probabilistic treatment both network logic and estimation of activity duration. The technique was first described in 1966 by Dr. Alan B. Pritsker of Purdue University and WW Happ.
Uncertainty quantification (UQ) is the science of quantitative characterization and estimation of uncertainties in both computational and real world applications. It tries to determine how likely certain outcomes are if some aspects of the system are not exactly known. An example would be to predict the acceleration of a human body in a head-on crash with another car: even if the speed was exactly known, small differences in the manufacturing of individual cars, how tightly every bolt has been tightened, etc., will lead to different results that can only be predicted in a statistical sense.

A risk register is a document used as a risk management tool and to fulfill regulatory compliance acting as a repository for all risks identified and includes additional information about each risk, e.g., nature of the risk, reference and owner, mitigation measures. It can be displayed as a scatterplot or as a table.

Event chain diagrams are visualizations that show the relationships between events and tasks and how the events affect each other.
When estimating the cost for a project, product or other item or investment, there is always uncertainty as to the precise content of all items in the estimate, how work will be performed, what work conditions will be like when the project is executed and so on. These uncertainties are risks to the project. Some refer to these risks as "known-unknowns" because the estimator is aware of them, and based on past experience, can even estimate their probable costs. The estimated costs of the known-unknowns is referred to by cost estimators as cost contingency.
A glossary of terms relating to project management and consulting.

In simple terms, risk is the possibility of something bad happening. Risk involves uncertainty about the effects/implications of an activity with respect to something that humans value, often focusing on negative, undesirable consequences. Many different definitions have been proposed. One international standard definition of risk is the "effect of uncertainty on objectives".
Risk management tools help address uncertainty by identifying risks, generating metrics, setting parameters, prioritizing issues, developing responses, and tracking risks. Without the use of these tools, techniques, documentation, and information systems, it can be challenging to effectively monitor these activities.
Criticality index is mainly used in risk analysis. The Criticality Index of an activity (task) can be expressed as a ratio but is more often expressed as a percentage. During a simulation tasks can join or leave the critical path for any given iteration. The Criticality Index expresses how often a particular task was on the Critical Path during the analysis. Tasks with a high Criticality Index are more likely to cause delay to the project as they are more likely to be on the Critical Path. If a task does not exist for some iterations then it is marked as not being critical. For example, a task that existed for 50% of the iterations and was critical 50% of the time it existed would have a Criticality Index of 25%.
The following outline is provided as an overview of and topical guide to project management:
Intaver Institute is a software development company that develops a suite of project management, risk analysis and risk managementapplications. Intaver Institute's product is project risk management and risk analysis software suite RiskyProject. Intaver Institute is a privately owned company headquartered in Naples, Florida, United States and Calgary, Alberta, Canada.
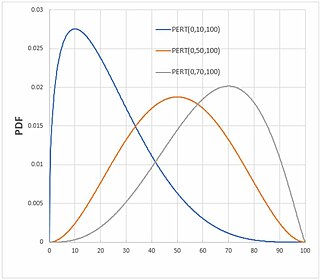
In probability and statistics, the PERT distributions are a family of continuous probability distributions defined by the minimum (a), most likely (b) and maximum (c) values that a variable can take. It is a transformation of the four-parameter beta distribution with an additional assumption that its expected value is













