| Isorrhoa triloxias | |
|---|---|
 | |
| Scientific classification | |
| Kingdom: | |
| Phylum: | |
| Class: | |
| Order: | |
| Family: | |
| Genus: | |
| Species: | I. triloxias |
| Binomial name | |
| Isorrhoa triloxias Meyrick, 1907 | |
Isorrhoa triloxias is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1907. It is found in Sri Lanka. [1]
In mathematics, convolution is a mathematical operation on two functions that produces a third function that expresses how the shape of one is modified by the other. The term convolution refers to both the result function and to the process of computing it. It is defined as the integral of the product of the two functions after one is reflected about the y-axis and shifted. The choice of which function is reflected and shifted before the integral does not change the integral result. The integral is evaluated for all values of shift, producing the convolution function.
G, or g, is the seventh letter in the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is gee, plural gees.
In physics, the kinetic energy of an object is the form of energy that it possesses due to its motion.
In mathematical analysis, the Dirac delta distribution, also known as the unit impulse, is a generalized function or distribution over the real numbers, whose value is zero everywhere except at zero, and whose integral over the entire real line is equal to one.
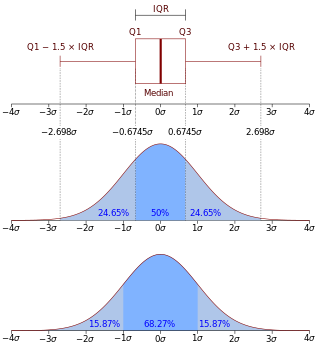
In probability theory, a probability density function (PDF), density function, or density of an absolutely continuous random variable, is a function whose value at any given sample in the sample space can be interpreted as providing a relative likelihood that the value of the random variable would be equal to that sample. Probability density is the probability per unit length, in other words, while the absolute likelihood for a continuous random variable to take on any particular value is 0, the value of the PDF at two different samples can be used to infer, in any particular draw of the random variable, how much more likely it is that the random variable would be close to one sample compared to the other sample.
Big O notation is a mathematical notation that describes the limiting behavior of a function when the argument tends towards a particular value or infinity. Big O is a member of a family of notations invented by German mathematicians Paul Bachmann, Edmund Landau, and others, collectively called Bachmann–Landau notation or asymptotic notation. The letter O was chosen by Bachmann to stand for Ordnung, meaning the order of approximation.
In physics, engineering and mathematics, the Fourier transform (FT) is an integral transform that converts a function into a form that describes the frequencies present in the original function. The output of the transform is a complex-valued function of frequency. The term Fourier transform refers to both this complex-valued function and the mathematical operation. When a distinction needs to be made the Fourier transform is sometimes called the frequency domain representation of the original function. The Fourier transform is analogous to decomposing the sound of a musical chord into the intensities of its constituent pitches.
In chemistry, the molar mass of a chemical compound is defined as the ratio between the mass and the amount of substance of any sample of said compound. The molar mass is a bulk, not molecular, property of a substance. The molar mass is an average of many instances of the compound, which often vary in mass due to the presence of isotopes. Most commonly, the molar mass is computed from the standard atomic weights and is thus a terrestrial average and a function of the relative abundance of the isotopes of the constituent atoms on Earth. The molar mass is appropriate for converting between the mass of a substance and the amount of a substance for bulk quantities.
In mathematics, a function from a set X to a set Y assigns to each element of X exactly one element of Y. The set X is called the domain of the function and the set Y is called the codomain of the function.
In thermodynamics, the Gibbs free energy is a thermodynamic potential that can be used to calculate the maximum amount of work, other than pressure-volume work, that may be performed by a thermodynamically closed system at constant temperature and pressure. It also provides a necessary condition for processes such as chemical reactions that may occur under these conditions. The Gibbs free energy is expressed as
Eudalaca is a genus of moths of the family Hepialidae. There are 35 described species, most restricted to South Africa but a few found further north in the continent.
The Momphidae, or mompha moths, is a family of moths with some 115 described species. It was described by Gottlieb August Wilhelm Herrich-Schäffer in 1857. These moths tend to be rather small with a wingspan of up to 21 mm. The wings are held folded over the body at rest. The larvae are concealed feeders, either as leaf miners or within seeds or stems.
Eudalaca isorrhoa is a species of moth of the family Hepialidae. It is known from South Africa.
Isorrhoa is a genus of moths in the family Cosmopterigidae.
Isorrhoa ancistrota is a moth in the family Cosmopterigidae. It was described by Turner in 1923. It is found in Australia, where it has been recorded from Queensland.
Isorrhoa antimetra is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1913. It is found in India.
Isorrhoa aphrosema is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1897. It is found in Australia, where it has been recorded from Queensland.
Isorrhoa implicata is a moth in the family Cosmopterigidae. It was described by Edward Meyrick in 1920. It is found in Australia, where it has been recorded from Queensland.
Isorrhoa loxoschema is a moth in the family Cosmopterigidae. It was described by Alfred Jefferis Turner in 1923. It is found in Australia.
Isorrhoa atmozona is a moth in the family Cosmopterigidae. It was described by Alfred Jefferis Turner in 1917. It is found in Australia.
