Related Research Articles

Origami is the Japanese art of paper folding. In modern usage, the word "origami" is often used as an inclusive term for all folding practices, regardless of their culture of origin. The goal is to transform a flat square sheet of paper into a finished sculpture through folding and sculpting techniques. Modern origami practitioners generally discourage the use of cuts, glue, or markings on the paper. Origami folders often use the Japanese word kirigami to refer to designs which use cuts.
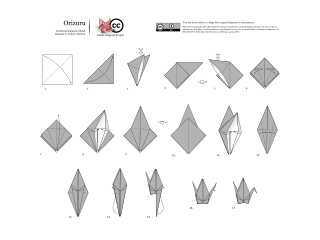
The Yoshizawa–Randlett system is a diagramming system used to describe the folds of origami models. Many origami books begin with a description of basic origami techniques which are used to construct the models. There are also a number of standard bases which are commonly used as a first step in construction. Models are typically classified as requiring low, intermediate or high skill depending on the complexity of the techniques involved in the construction.

The discipline of origami or paper folding has received a considerable amount of mathematical study. Fields of interest include a given paper model's flat-foldability, and the use of paper folds to solve up-to cubic mathematical equations.

Chinese paper folding, or zhezhi, is the art of paper folding that originated in medieval China.

Robert James Lang is an American physicist who is also one of the foremost origami artists and theorists in the world. He is known for his complex and elegant designs, most notably of insects and animals. He has studied the mathematics of origami and used computers to study the theories behind origami. He has made great advances in making real-world applications of origami to engineering problems.

John Montroll is an American origami artist, author, teacher, and mathematician. He has written many books on origami, promoting the single-square, no-cut, no glue approach. Montroll taught mathematics at St. Anselm's Abbey School in Washington, D.C. from 1990 to 2021.

Akira Yoshizawa was a Japanese origamist, considered to be the grandmaster of origami. He is credited with raising origami from a craft to a living art. According to his own estimation made in 1989, he created more than 50,000 models, of which only a few hundred designs were presented as diagrams in his 18 books. Yoshizawa acted as an international cultural ambassador for Japan throughout his career. In 1983, Emperor Hirohito awarded him the Order of the Rising Sun, 5th class, one of the highest honors bestowed in Japan.

Wet-folding is an origami technique developed by Akira Yoshizawa that employs water to dampen the paper so that it can be manipulated more easily. This process adds an element of sculpture to origami, which is otherwise purely geometric. Wet-folding is used very often by professional folders for non-geometric origami, such as animals. Wet-folders usually employ thicker paper than what would usually be used for normal origami, to ensure that the paper does not tear.
Satoshi Kamiya is a Japanese origami artist. Kamiya began folding at age two. Kamiya began designing origami models in 1995, and has since published hundreds of creations. Kamiya has drawn inspiration for his designs from manga, nature, and both eastern and western mythologies.
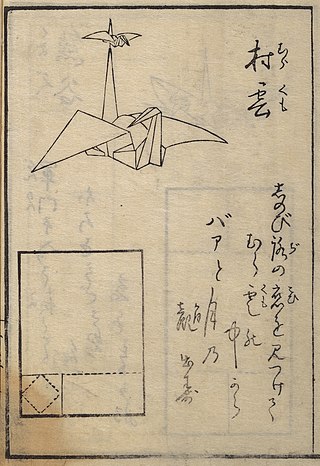
The history of origami followed after the invention of paper and was a result of paper's use in society. In the detailed Japanese classification, origami is divided into stylized ceremonial origami and recreational origami, and only recreational origami is generally recognized as origami. However, this page describes the history of both ceremonial and recreational origami.

Toshikazu Kawasaki is a Japanese paperfolder and origami theorist who is known for his geometrically innovative models. He is particularly famous for his series of fourfold symmetry "roses", all based on a twisting maneuver that allows the petals to seem to curl out from the center of the flower. Kawasaki also teaches mathematics at Sasebo Technical Junior College.
Lillian Vorhaus Oppenheimer was an origami pioneer from New York City. Becoming a leading figure in the art form in her later years, Oppenheimer is credited with popularizing it in the United States. She adopted the Japanese word origami instead of the English paper folding, and the foreign term became established in the English language due to her efforts.
The Bug Wars were origami contests among members of the Origami Detectives which started when one member made a bug, a horned beetle with outspread wings, from a single sheet of paper: this design provoked other members to design more complex origami in the shape of bugs, such as wasps and praying mantises.

Kawasaki's theorem or Kawasaki–Justin theorem is a theorem in the mathematics of paper folding that describes the crease patterns with a single vertex that may be folded to form a flat figure. It states that the pattern is flat-foldable if and only if alternatingly adding and subtracting the angles of consecutive folds around the vertex gives an alternating sum of zero. Crease patterns with more than one vertex do not obey such a simple criterion, and are NP-hard to fold.

Rigid origami is a branch of origami which is concerned with folding structures using flat rigid sheets joined by hinges. That is, unlike in traditional origami, the panels of the paper cannot be bent during the folding process; they must remain flat at all times, and the paper only folded along its hinges. A rigid origami model would still be foldable if it was made from glass sheets with hinges in place of its crease lines.
Ligia Montoya was an Argentinian paper-folding artist, who played an important role in all aspects of the 'golden age' of the international origami movement from the 1950s, from which developed modern artistic origami—that is, innovative paper-folding exploring a variety of different approaches, rather than repeating limited traditional figures.
Samuel L Randlett is an American origami artist who helped develop the modern system for diagramming origami folds. Together with Robert Harbin he developed the notation introduced by Akira Yoshizawa to form what is now called the Yoshizawa-Randlett system. This was first described in Samuel Randlett's Art of Origami in 1961.

Alice E. Gray was an American entomologist and origamist. She worked as an entomologist at the American Museum of Natural History (AMNH) in New York for 43 years, writing, illustrating, and creating large models of insects. Known as the "Bug Lady", she conducted outreach and education in the museum, in local schools, and appeared on The Tonight Show. She began practicing origami first as an extension of her interest in insects, starting a tradition of using origami creatures to decorate the museum's Christmas tree. In the 1960s, she became more involved with the origami community and, in 1978, co-founded the Friends of the Origami Center of America in New York with Lillian Oppenheimer and Michael Shall, now known as OrigamiUSA.
In the mathematics of paper folding, the big-little-big lemma is a necessary condition for a crease pattern with specified mountain folds and valley folds to be able to be folded flat. It differs from Kawasaki's theorem, which characterizes the flat-foldable crease patterns in which a mountain-valley assignment has not yet been made. Together with Maekawa's theorem on the total number of folds of each type, the big-little-big lemma is one of the two main conditions used to characterize the flat-foldability of mountain-valley assignments for crease patterns that meet the conditions of Kawasaki's theorem. Mathematical origami expert Tom Hull calls the big-little-big lemma "one of the most basic rules" for flat foldability of crease patterns.
Tomohiro Tachi is a Japanese academic who studies origami from an interdisciplinary perspective, combining approaches from the mathematics of paper folding, structural rigidity, computational geometry, architecture, and materials science. His work was profiled in "The Origami Revolution" (2017), part of the Nova series of US science documentaries. He is a professor at the University of Tokyo.
References
- 1 2 3 4 "11 Cutting-Edge Origami Artists Who Are Masters of Paper Folding". My Modern Met. 2017-04-05. Retrieved 2020-03-27.
- 1 2 3 4 5 6 7 8 "artists | Folding Paper | Japanese American National Museum". www.janm.org. Retrieved 2020-03-27.
- 1 2 3 Callahan, Sophia (2014-10-16). "8 Artists Pushing Origami To The Extreme". Vice. Retrieved 2020-03-27.
- ↑ "An Origami Artist Shows How to Fold Ultra-Realistic Creatures". Wired. ISSN 1059-1028 . Retrieved 2020-03-27.
- ↑ TASON. "Robert J. Lang Origami". Robert J. Lang Origami. Retrieved 2020-03-27.
- ↑ "www.mabonaorigami.com: startseite". www.mabonaorigami.com. Retrieved 2020-03-27.
- 1 2 "Math Unfolded: A series of presentations on the mathematics of origami – National Museum of Mathematics" . Retrieved 2020-03-27.
- ↑ "World Origami Days". Origami USA | World Origami Days. 2012-02-01. Retrieved 2020-12-11.
- ↑ "Nick Robinson - contributor". Britannica. Retrieved 4 September 2024.
- ↑ "Nick Robinson British Origami". British Origami. Retrieved 4 September 2024.