In mathematics, any vector space has a corresponding dual vector space consisting of all linear forms on together with the vector space structure of pointwise addition and scalar multiplication by constants.
In mathematics, weak topology is an alternative term for certain initial topologies, often on topological vector spaces or spaces of linear operators, for instance on a Hilbert space. The term is most commonly used for the initial topology of a topological vector space with respect to its continuous dual. The remainder of this article will deal with this case, which is one of the concepts of functional analysis.
In topology and related branches of mathematics, a topological space is called locally compact if, roughly speaking, each small portion of the space looks like a small portion of a compact space. More precisely, it is a topological space in which every point has a compact neighborhood.
The Baire category theorem (BCT) is an important result in general topology and functional analysis. The theorem has two forms, each of which gives sufficient conditions for a topological space to be a Baire space. It is used in the proof of results in many areas of analysis and geometry, including some of the fundamental theorems of functional analysis.

In topology and mathematics in general, the boundary of a subset S of a topological space X is the set of points in the closure of S not belonging to the interior of S. An element of the boundary of S is called a boundary point of S. The term boundary operation refers to finding or taking the boundary of a set. Notations used for boundary of a set S include and . Some authors use the term frontier instead of boundary in an attempt to avoid confusion with a different definition used in algebraic topology and the theory of manifolds. Despite widespread acceptance of the meaning of the terms boundary and frontier, they have sometimes been used to refer to other sets. For example, Metric Spaces by E. T. Copson uses the term boundary to refer to Hausdorff's border, which is defined as the intersection of a set with its boundary. Hausdorff also introduced the term residue, which is defined as the intersection of a set with the closure of the border of its complement.
In mathematics, a diffeology on a set generalizes the concept of smooth charts in a differentiable manifold, declaring what the "smooth parametrizations" in the set are.
In mathematics, a sheaf is a tool for systematically tracking data attached to the open sets of a topological space and defined locally with regard to them. For example, for each open set, the data could be the ring of continuous functions defined on that open set. Such data is well behaved in that it can be restricted to smaller open sets, and also the data assigned to an open set is equivalent to all collections of compatible data assigned to collections of smaller open sets covering the original open set.
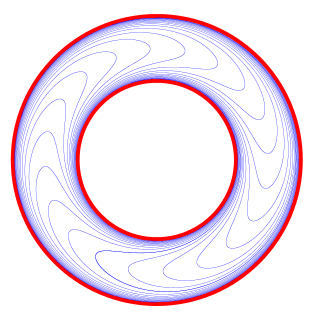
In mathematics, a foliation is an equivalence relation on an n-manifold, the equivalence classes being connected, injectively immersed submanifolds, all of the same dimension p, modeled on the decomposition of the real coordinate space Rn into the cosets x + Rp of the standardly embedded subspace Rp. The equivalence classes are called the leaves of the foliation. If the manifold and/or the submanifolds are required to have a piecewise-linear, differentiable, or analytic structure then one defines piecewise-linear, differentiable, or analytic foliations, respectively. In the most important case of differentiable foliation of class Cr it is usually understood that r ≥ 1. The number p is called the dimension of the foliation and q = n − p is called its codimension.
In mathematics, more specifically in topology, an open map is a function between two topological spaces that maps open sets to open sets. That is, a function is open if for any open set in the image is open in Likewise, a closed map is a function that maps closed sets to closed sets. A map may be open, closed, both, or neither; in particular, an open map need not be closed and vice versa.
In functional analysis and related areas of mathematics, locally convex topological vector spaces (LCTVS) or locally convex spaces are examples of topological vector spaces (TVS) that generalize normed spaces. They can be defined as topological vector spaces whose topology is generated by translations of balanced, absorbent, convex sets. Alternatively they can be defined as a vector space with a family of seminorms, and a topology can be defined in terms of that family. Although in general such spaces are not necessarily normable, the existence of a convex local base for the zero vector is strong enough for the Hahn–Banach theorem to hold, yielding a sufficiently rich theory of continuous linear functionals.

In mathematics, a submanifold of a manifold is a subset which itself has the structure of a manifold, and for which the inclusion map satisfies certain properties. There are different types of submanifolds depending on exactly which properties are required. Different authors often have different definitions.
In mathematics, an n-dimensional differential structure on a set M makes M into an n-dimensional differential manifold, which is a topological manifold with some additional structure that allows for differential calculus on the manifold. If M is already a topological manifold, it is required that the new topology be identical to the existing one.
In topology, a branch of mathematics, a topological manifold is a topological space that locally resembles real n-dimensional Euclidean space. Topological manifolds are an important class of topological spaces, with applications throughout mathematics. All manifolds are topological manifolds by definition. Other types of manifolds are formed by adding structure to a topological manifold. Every manifold has an "underlying" topological manifold, obtained by simply "forgetting" the added structure. However, not every topological manifold can be endowed with a particular additional structure. For example, the E8 manifold is a topological manifold which cannot be endowed with a differentiable structure.
In mathematics, a Banach manifold is a manifold modeled on Banach spaces. Thus it is a topological space in which each point has a neighbourhood homeomorphic to an open set in a Banach space. Banach manifolds are one possibility of extending manifolds to infinite dimensions.
In mathematics, subharmonic and superharmonic functions are important classes of functions used extensively in partial differential equations, complex analysis and potential theory.
In topology, a branch of mathematics, the ends of a topological space are, roughly speaking, the connected components of the "ideal boundary" of the space. That is, each end represents a topologically distinct way to move to infinity within the space. Adding a point at each end yields a compactification of the original space, known as the end compactification.
In the mathematical field of topology, a hyperconnected space or irreducible space is a topological space X that cannot be written as the union of two proper closed sets. The name irreducible space is preferred in algebraic geometry.
In topology, a branch of mathematics, local flatness is a smoothness condition that can be imposed on topological submanifolds. In the category of topological manifolds, locally flat submanifolds play a role similar to that of embedded submanifolds in the category of smooth manifolds. Violations of local flatness describe ridge networks and crumpled structures, with applications to materials processing and mechanical engineering.
In topology and related areas of mathematics, a subset A of a topological space X is said to be dense in X if every point of X either belongs to A or else is arbitrarily "close" to a member of A — for instance, the rational numbers are a dense subset of the real numbers because every real number either is a rational number or has a rational number arbitrarily close to it. Formally, is dense in if the smallest closed subset of containing is itself.
































