In mathematics, a modular form is a (complex) analytic function on the upper half-plane, , that satisfies:
In mathematics, a Lie superalgebra is a generalisation of a Lie algebra to include a ‑grading. Lie superalgebras are important in theoretical physics where they are used to describe the mathematics of supersymmetry.

In mathematics, the modular group is the projective special linear group of 2 × 2 matrices with integer coefficients and determinant 1. The matrices A and −A are identified. The modular group acts on the upper-half of the complex plane by fractional linear transformations, and the name "modular group" comes from the relation to moduli spaces and not from modular arithmetic.
In mathematics, in particular algebraic geometry, a moduli space is a geometric space whose points represent algebro-geometric objects of some fixed kind, or isomorphism classes of such objects. Such spaces frequently arise as solutions to classification problems: If one can show that a collection of interesting objects can be given the structure of a geometric space, then one can parametrize such objects by introducing coordinates on the resulting space. In this context, the term "modulus" is used synonymously with "parameter"; moduli spaces were first understood as spaces of parameters rather than as spaces of objects. A variant of moduli spaces is formal moduli. Bernhard Riemann first used the term "moduli" in 1857.

In mathematics, Felix Klein's j-invariant or j function, regarded as a function of a complex variable τ, is a modular function of weight zero for special linear group SL(2, Z) defined on the upper half-plane of complex numbers. It is the unique such function that is holomorphic away from a simple pole at the cusp such that
In mathematics, complex multiplication (CM) is the theory of elliptic curves E that have an endomorphism ring larger than the integers. Put another way, it contains the theory of elliptic functions with extra symmetries, such as are visible when the period lattice is the Gaussian integer lattice or Eisenstein integer lattice.
In number theory and algebraic geometry, a modular curveY(Γ) is a Riemann surface, or the corresponding algebraic curve, constructed as a quotient of the complex upper half-plane H by the action of a congruence subgroup Γ of the modular group of integral 2×2 matrices SL(2, Z). The term modular curve can also be used to refer to the compactified modular curvesX(Γ) which are compactifications obtained by adding finitely many points to this quotient. The points of a modular curve parametrize isomorphism classes of elliptic curves, together with some additional structure depending on the group Γ. This interpretation allows one to give a purely algebraic definition of modular curves, without reference to complex numbers, and, moreover, prove that modular curves are defined either over the field of rational numbers Q or a cyclotomic field Q(ζn). The latter fact and its generalizations are of fundamental importance in number theory.
The representation theory of groups is a part of mathematics which examines how groups act on given structures.
In mathematics and theoretical physics, the induced metric is the metric tensor defined on a submanifold that is induced from the metric tensor on a manifold into which the submanifold is embedded, through the pullback. It may be determined using the following formula, which is the component form of the pullback operation:
Eisenstein series, named after German mathematician Gotthold Eisenstein, are particular modular forms with infinite series expansions that may be written down directly. Originally defined for the modular group, Eisenstein series can be generalized in the theory of automorphic forms.
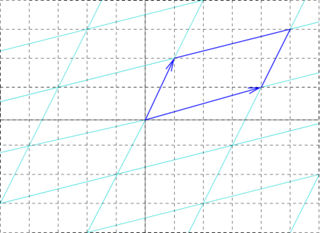
In mathematics, a fundamental pair of periods is an ordered pair of complex numbers that defines a lattice in the complex plane. This type of lattice is the underlying object with which elliptic functions and modular forms are defined.
In algebraic geometry, a moduli space of (algebraic) curves is a geometric space whose points represent isomorphism classes of algebraic curves. It is thus a special case of a moduli space. Depending on the restrictions applied to the classes of algebraic curves considered, the corresponding moduli problem and the moduli space is different. One also distinguishes between fine and coarse moduli spaces for the same moduli problem.

In mathematics, a complex torus is a particular kind of complex manifold M whose underlying smooth manifold is a torus in the usual sense. Here N must be the even number 2n, where n is the complex dimension of M.
In theoretical physics, Seiberg–Witten theory is an supersymmetric gauge theory with an exact low-energy effective action, of which the kinetic part coincides with the Kähler potential of the moduli space of vacua. Before taking the low-energy effective action, the theory is known as supersymmetric Yang–Mills theory, as the field content is a single vector supermultiplet, analogous to the field content of Yang–Mills theory being a single vector gauge field or connection.
In mathematics, the Harish-Chandra isomorphism, introduced by Harish-Chandra , is an isomorphism of commutative rings constructed in the theory of Lie algebras. The isomorphism maps the center of the universal enveloping algebra of a reductive Lie algebra to the elements of the symmetric algebra of a Cartan subalgebra that are invariant under the Weyl group .
In mathematics a stack or 2-sheaf is, roughly speaking, a sheaf that takes values in categories rather than sets. Stacks are used to formalise some of the main constructions of descent theory, and to construct fine moduli stacks when fine moduli spaces do not exist.
In mathematics, the ELSV formula, named after its four authors Torsten Ekedahl, Sergei Lando, Michael Shapiro, Alek Vainshtein, is an equality between a Hurwitz number and an integral over the moduli space of stable curves.
In the mathematical field of algebraic number theory, the concept of principalization refers to a situation when, given an extension of algebraic number fields, some ideal of the ring of integers of the smaller field isn't principal but its extension to the ring of integers of the larger field is. Its study has origins in the work of Ernst Kummer on ideal numbers from the 1840s, who in particular proved that for every algebraic number field there exists an extension number field such that all ideals of the ring of integers of the base field become principal when extended to the larger field. In 1897 David Hilbert conjectured that the maximal abelian unramified extension of the base field, which was later called the Hilbert class field of the given base field, is such an extension. This conjecture, now known as principal ideal theorem, was proved by Philipp Furtwängler in 1930 after it had been translated from number theory to group theory by Emil Artin in 1929, who made use of his general reciprocity law to establish the reformulation. Since this long desired proof was achieved by means of Artin transfers of non-abelian groups with derived length two, several investigators tried to exploit the theory of such groups further to obtain additional information on the principalization in intermediate fields between the base field and its Hilbert class field. The first contributions in this direction are due to Arnold Scholz and Olga Taussky in 1934, who coined the synonym capitulation for principalization. Another independent access to the principalization problem via Galois cohomology of unit groups is also due to Hilbert and goes back to the chapter on cyclic extensions of number fields of prime degree in his number report, which culminates in the famous Theorem 94.
In algebraic geometry, a level structure on a space X is an extra structure attached to X that shrinks or eliminates the automorphism group of X, by demanding automorphisms to preserve the level structure; attaching a level structure is often phrased as rigidifying the geometry of X.
Abelian varieties are a natural generalization of elliptic curves, including algebraic tori in higher dimensions. Just as elliptic curves have a natural moduli space over characteristic 0 constructed as a quotient of the upper-half plane by the action of , there is an analogous construction for abelian varieties using the Siegel upper half-space and the symplectic group .

























































































