In mathematics, specifically in measure theory, a Borel measure on a topological space is a measure that is defined on all open sets. Some authors require additional restrictions on the measure, as described below.
In mathematics, one can often define a direct product of objects already known, giving a new one. This induces a structure on the Cartesian product of the underlying sets from that of the contributing objects. More abstractly, one talks about the product in category theory, which formalizes these notions.
In mathematical logic, model theory is the study of the relationship between formal theories, and their models. The aspects investigated include the number and size of models of a theory, the relationship of different models to each other, and their interaction with the formal language itself. In particular, model theorists also investigate the sets that can be defined in a model of a theory, and the relationship of such definable sets to each other. As a separate discipline, model theory goes back to Alfred Tarski, who first used the term "Theory of Models" in publication in 1954. Since the 1970s, the subject has been shaped decisively by Saharon Shelah's stability theory.
In mathematical logic, the compactness theorem states that a set of first-order sentences has a model if and only if every finite subset of it has a model. This theorem is an important tool in model theory, as it provides a useful method for constructing models of any set of sentences that is finitely consistent.
In mathematics, the adele ring of a global field is a central object of class field theory, a branch of algebraic number theory. It is the restricted product of all the completions of the global field and is an example of a self-dual topological ring.
Rado's theorem is a theorem from the branch of mathematics known as Ramsey theory. It is named for the German mathematician Richard Rado. It was proved in his thesis, Studien zur Kombinatorik.
In mathematics, piecewise syndeticity is a notion of largeness of subsets of the natural numbers.
In mathematics, an IP set is a set of natural numbers which contains all finite sums of some infinite set.
In mathematics, the Milliken–Taylor theorem in combinatorics is a generalization of both Ramsey's theorem and Hindman's theorem. It is named after Keith Milliken and Alan D. Taylor.
In mathematics, Milliken's tree theorem in combinatorics is a partition theorem generalizing Ramsey's theorem to infinite trees, objects with more structure than sets.
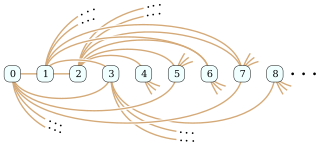
In the mathematical field of graph theory, the Rado graph, Erdős–Rényi graph, or random graph is a countably infinite graph that can be constructed by choosing independently at random for each pair of its vertices whether to connect the vertices by an edge. The names of this graph honor Richard Rado, Paul Erdős, and Alfréd Rényi, mathematicians who studied it in the early 1960s; it appears even earlier in the work of Wilhelm Ackermann. The Rado graph can also be constructed non-randomly, by symmetrizing the membership relation of the hereditarily finite sets, by applying the BIT predicate to the binary representations of the natural numbers, or as an infinite Paley graph that has edges connecting pairs of prime numbers congruent to 1 mod 4 that are quadratic residues modulo each other.
In mathematics, the Vitali covering lemma is a combinatorial and geometric result commonly used in measure theory of Euclidean spaces. This lemma is an intermediate step, of independent interest, in the proof of the Vitali covering theorem. The covering theorem is credited to the Italian mathematician Giuseppe Vitali. The theorem states that it is possible to cover, up to a Lebesgue-negligible set, a given subset E of Rd by a disjoint family extracted from a Vitali covering of E.
In symbolic dynamics and related branches of mathematics, a shift space or subshift is a set of infinite words that represent the evolution of a discrete system. In fact, shift spaces and symbolic dynamical systems are often considered synonyms. The most widely studied shift spaces are the subshifts of finite type and the sofic shifts.
In mathematics, infinitary combinatorics, or combinatorial set theory, is an extension of ideas in combinatorics to infinite sets. Some of the things studied include continuous graphs and trees, extensions of Ramsey's theorem, and Martin's axiom. Recent developments concern combinatorics of the continuum and combinatorics on successors of singular cardinals.
In the mathematical theory of infinite graphs, the Erdős–Dushnik–Miller theorem is a form of Ramsey's theorem stating that every infinite graph contains either a countably infinite independent set, or a clique with the same cardinality as the whole graph.
Folkman's theorem is a theorem in mathematics, and more particularly in arithmetic combinatorics and Ramsey theory. According to this theorem, whenever the natural numbers are partitioned into finitely many subsets, there exist arbitrarily large sets of numbers all of whose sums belong to the same subset of the partition. The theorem had been discovered and proved independently by several mathematicians, before it was named "Folkman's theorem", as a memorial to Jon Folkman, by Graham, Rothschild, and Spencer.
In mathematics, a polyadic space is a topological space that is the image under a continuous function of a topological power of an Alexandroff one-point compactification of a discrete space.
In mathematics, structural Ramsey theory is a categorical generalisation of Ramsey theory, rooted in the idea that many important results of Ramsey theory have "similar" logical structures. The key observation is noting that these Ramsey-type theorems can be expressed as the assertion that a certain category has the Ramsey property.
In mathematics, the hypergraph regularity method is a powerful tool in extremal graph theory that refers to the combined application of the hypergraph regularity lemma and the associated counting lemma. It is a generalization of the graph regularity method, which refers to the use of Szemerédi's regularity and counting lemmas.








































