
In mathematics, the gamma function is one commonly used extension of the factorial function to complex numbers. The gamma function is defined for all complex numbers except the non-positive integers. For any positive integer n,

Euler's constant is a mathematical constant usually denoted by the lowercase Greek letter gamma.

In mathematics, Stirling's approximation is an approximation for factorials. It is a good approximation, leading to accurate results even for small values of . It is named after James Stirling, though a related but less precise result was first stated by Abraham de Moivre.
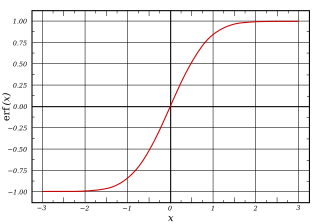
In mathematics, the error function, often denoted by erf, is a complex function of a complex variable defined as:

In mathematics, the n-th harmonic number is the sum of the reciprocals of the first n natural numbers:
In the physical sciences, the Airy functionAi(x) is a special function named after the British astronomer George Biddell Airy (1801–1892). The function Ai(x) and the related function Bi(x), are linearly independent solutions to the differential equation
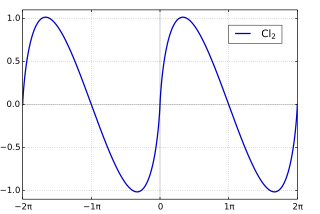
In mathematics, the Clausen function, introduced by Thomas Clausen (1832), is a transcendental, special function of a single variable. It can variously be expressed in the form of a definite integral, a trigonometric series, and various other forms. It is intimately connected with the polylogarithm, inverse tangent integral, polygamma function, Riemann zeta function, Dirichlet eta function, and Dirichlet beta function.

In mathematics, the digamma function is defined as the logarithmic derivative of the gamma function:

In mathematics, theta functions are special functions of several complex variables. They are important in many areas, including the theories of Abelian varieties and moduli spaces, and of quadratic forms. They have also been applied to soliton theory. When generalized to a Grassmann algebra, they also appear in quantum field theory.
In the mathematical field of complex analysis, contour integration is a method of evaluating certain integrals along paths in the complex plane.

In probability theory, a distribution is said to be stable if a linear combination of two independent random variables with this distribution has the same distribution, up to location and scale parameters. A random variable is said to be stable if its distribution is stable. The stable distribution family is also sometimes referred to as the Lévy alpha-stable distribution, after Paul Lévy, the first mathematician to have studied it.

In mathematics, the Stieltjes constants are the numbers that occur in the Laurent series expansion of the Riemann zeta function:

In mathematics, the Barnes G-functionG(z) is a function that is an extension of superfactorials to the complex numbers. It is related to the gamma function, the K-function and the Glaisher–Kinkelin constant, and was named after mathematician Ernest William Barnes. It can be written in terms of the double gamma function.
In mathematics, particularly q-analog theory, the Ramanujan theta function generalizes the form of the Jacobi theta functions, while capturing their general properties. In particular, the Jacobi triple product takes on a particularly elegant form when written in terms of the Ramanujan theta. The function is named after Srinivasa Ramanujan.
The gamma function is an important special function in mathematics. Its particular values can be expressed in closed form for integer and half-integer arguments, but no simple expressions are known for the values at rational points in general. Other fractional arguments can be approximated through efficient infinite products, infinite series, and recurrence relations.
In mathematics, Maass forms or Maass wave forms are studied in the theory of automorphic forms. Maass forms are complex-valued smooth functions of the upper half plane, which transform in a similar way under the operation of a discrete subgroup of as modular forms. They are Eigenforms of the hyperbolic Laplace Operator defined on and satisfy certain growth conditions at the cusps of a fundamental domain of . In contrast to the modular forms the Maass forms need not be holomorphic. They were studied first by Hans Maass in 1949.
A product distribution is a probability distribution constructed as the distribution of the product of random variables having two other known distributions. Given two statistically independent random variables X and Y, the distribution of the random variable Z that is formed as the product
In mathematics, the p-adic gamma function Γp is a function of a p-adic variable analogous to the gamma function. It was first explicitly defined by Morita (1975), though Boyarsky (1980) pointed out that Dwork (1964) implicitly used the same function. Diamond (1977) defined a p-adic analog Gp of log Γ. Overholtzer (1952) had previously given a definition of a different p-adic analogue of the gamma function, but his function does not have satisfactory properties and is not used much.

In mathematics, Ramanujan's master theorem is a technique that provides an analytic expression for the Mellin transform of an analytic function.















































