Rock mass rating

Last updated July 01, 2024

The rock mass rating (RMR) is a geomechanical classification system for rocks, developed by Z. T. Bieniawski between 1972 and 1973.^[1] Since then it has undergone multiple modifications out of which, RMR89^[1] is commonly used. Recently RMR14^[2] has been proposed to improve the RMR performance by incorporating new experiences from tunnel practices. Continuous functions and a software "QuickRMR" for RMR89 and RMR14 have also been proposed by Kundu.^[3] RMR combines the most significant geologic parameters of influence and represents them with one overall comprehensive index of rock mass quality, which is used for the design and construction of excavations in rock, such as tunnels, mines, slopes, and foundations.

Definition

The following six parameters are used to classify a rock mass using the RMR system

Uniaxial compressive strength of rock material
Rock quality designation (RQD)
Spacing of discontinuities
Condition of discontinuities.
Groundwater conditions
Orientation of discontinuities

Each of the six parameters is assigned a value corresponding to the characteristics of the rock. These values are derived from field surveys and laboratory tests. The sum of the six parameters is the "RMR value", which lies between 0 and 100.

Classification table

Below is the classification table for the RMR system.

RMR	Rock quality
0 - 20	Very Poor
21 - 40	Poor
41 - 60	Fair
61 - 80	Good
81 - 100	Very good

Procedures

The details for calculating RMR are given by Edumine^[4] and provide a series of tables for RMR determination while the latest charts for the same purpose are given in the references and further reading. In particular, the charts enclosed here for the RMR parameters intact rock strength and the combined parameters RQD and discontinuity spacing (represented by the number of discontinuities per meter), show the advantage of using the charts for better accuracy, rather than relying on the tables which show the average ratings for the ranges of each RMR parameter. The lump ratings for each parameter makes it difficult for less experienced personnel to determine the RMR accurately. There are also subjective parameters like roughness and weathering which may pose difficulty in rating assignment. Kundu et al. have proposed continuous functions for each parameter of RMR89 and RMR14 including roughness and weathering.^[3] They have also developed a software "QuickRMR" based on the continuous functions to calculate RMR with quantitative inputs.

User Interface of QuickRMR-89 QuickRMR-89.jpg — User Interface of QuickRMR-89

Applications

Rock Mass Rating RMR has found wide applications in various types of engineering projects such as tunnels, slopes, foundations, and mines. It is also adaptable for knowledge-based expert systems. Engineers informally classify rock structure into two general classifications: continuous homogenous isotropic linear elastic (what most geotechnical engineers would like to see) and discontinuous inhomogenous anisotropic non-elastic (what most in-situ rock masses actually are). A rock mass rating system provides a method of incorporating some of the complex mechanics of actual rocks into engineering design.

Moreover, the system was the first to enable estimation of rock mass properties, such as the modulus of deformation, in addition to providing tunnel support guidelines and the stand-up time of underground excavations.^[5]

Recently, after over 40 years of use, renewed attention was paid to the RMR System because of its applications to the assessment of rock mass excavability (RME) and, especially, its direct correlation with the specific energy of excavation (SEE) for TBMs used effectively to detect changes in tunneling conditions, in real time, thus serving as a warning of adverse conditions as construction proceeds.^[6]

Rock Mass Rating presents some difficulties when applied to rock slopes, since the parameter that take into account the influence of the discontinuities orientation is introduced in detail for dam foundations and tunnels but not for slopes.^[7] To address this issue, Romana^[8] defined Slope Mass Rating scheme that is based on the original Bieniawski's parameters but including a rigorous definition of the parameters considering the effect of the orientation of discontinuities.

Specific output charts for tunnel design

For convenience in tunnel design, three charts are included which are commonly used to estimate these essential rock mass properties: Stand up time, Rock mass deformability modulus Em and Rock mass strength.

In the second chart, an improved relationship for the range of RMR greater than 56 is given. This reflects the idea that, at high RMR, deformations will be dominated by intact modulus, whereas at lower RMR weathering and joint infilling will largely control deformation. This approach has the advantage that modulus values are NOT overestimated at the higher range nor underestimated or overestimated at the lower range. This is more realistic than relying on one sigmoidal equation.

A number of sigmoidal equations have been proposed that give rock mass modulus as a function of intact modulus and a rock mass rating. These equations may give a good estimate of modulus given the correct input data, however it is difficult to obtain reliable intact strength or intact modulus values from laboratory tests on samples from highly disturbed rock masses. Because of this limitation, something that is commonly done in practice is to base intact modulus values on test results done on good samples of intact rock from locations with competent rock, using either laboratory measurements of intact modulus or on an assumed ratio between intact strength and modulus for a particular rock type. This ignores the possibility that the material in zones with poor rock will often be highly weathered, and it ignores the possibility that even without weathering a zone of poor rock may represent rock which simply has a lower intact strength, and that is why it became disturbed while zones of stronger rock on the same project did not.

The tunnel support guidelines based on RMR were provided originally in the form of a table giving support recommendations for a tunnel span/diameter of 10 meters. In view of the improving technology for rock bolting, shotcrete and steel ribs, it was left to tunnel designers to modify these guidelines for other tunnel sizes, which served its purpose well. Today, after 40 years of use, it has become apparent that it would be convenient for practical tunnel designers to have charts for the selection of rock support as a function of both tunnel size and rock mass quality. This is depicted in the chart below (see Lawson 2013).

Related Research Articles

The New Austrian tunneling method (NATM), also known as the sequential excavation method (SEM) or sprayed concrete lining method (SCL), is a method of modern tunnel design and construction employing sophisticated monitoring to optimize various wall reinforcement techniques based on the type of rock encountered as tunneling progresses. This technique first gained attention in the 1960s based on the work of Ladislaus von Rabcewicz, Leopold Müller, and Franz Pacher between 1957 and 1965 in Austria. The name NATM was intended to distinguish it from earlier methods, with its economic advantage of employing inherent geological strength available in the surrounding rock mass to stabilize the tunnel wherever possible rather than reinforcing the entire tunnel.

Soil mechanics is a branch of soil physics and applied mechanics that describes the behavior of soils. It differs from fluid mechanics and solid mechanics in the sense that soils consist of a heterogeneous mixture of fluids and particles but soil may also contain organic solids and other matter. Along with rock mechanics, soil mechanics provides the theoretical basis for analysis in geotechnical engineering, a subdiscipline of civil engineering, and engineering geology, a subdiscipline of geology. Soil mechanics is used to analyze the deformations of and flow of fluids within natural and man-made structures that are supported on or made of soil, or structures that are buried in soils. Example applications are building and bridge foundations, retaining walls, dams, and buried pipeline systems. Principles of soil mechanics are also used in related disciplines such as geophysical engineering, coastal engineering, agricultural engineering, hydrology and soil physics.

Slope stability refers to the condition of inclined soil or rock slopes to withstand or undergo movement; the opposite condition is called slope instability or slope failure. The stability condition of slopes is a subject of study and research in soil mechanics, geotechnical engineering and engineering geology. Analyses are generally aimed at understanding the causes of an occurred slope failure, or the factors that can potentially trigger a slope movement, resulting in a landslide, as well as at preventing the initiation of such movement, slowing it down or arresting it through mitigation countermeasures.

Rock mass classification systems are used for various engineering design and stability analysis. These are based on empirical relations between rock mass parameters and engineering applications, such as tunnels, slopes, foundations, and excavatability. The first rock mass classification system in geotechnical engineering was proposed in 1946 for tunnels with steel set support.

Rock Structure Rating (RSR) is a quantitative method for describing quality of a rock mass and appropriate ground support, in particular, for steel-rib support, developed by Wickham, Tiedemann and Skinner.

A rockfall or rock-fall is a quantity/sheets of rock that has fallen freely from a cliff face. The term is also used for collapse of rock from roof or walls of mine or quarry workings. "A rockfall is a fragment of rock detached by sliding, toppling, or falling, that falls along a vertical or sub-vertical cliff, proceeds down slope by bouncing and flying along ballistic trajectories or by rolling on talus or debris slopes."

<span class="mw-page-title-main">Rock mechanics</span> Study of the mechanical behavior of rocks

Rock mechanics is a theoretical and applied science of the mechanical behavior of rocks and rock masses.

A universal testing machine (UTM), also known as a universal tester, universal tensile machine, materials testing machine, materials test frame, is used to test the tensile strength (pulling) and compressive strength (pushing), flexural strength, bending, shear, hardness, and torsion testing, providing valuable data for designing and ensuring the quality of materials. An earlier name for a tensile testing machine is a tensometer. The "universal" part of the name reflects that it can perform many standard tests application on materials, components, and structures.

There have been known various classifications of landslides. Broad definitions include forms of mass movement that narrower definitions exclude. For example, the McGraw-Hill Encyclopedia of Science and Technology distinguishes the following types of landslides:

Slope mass rating (SMR) is a rock mass classification scheme developed by Manuel Romana to describe the strength of an individual rock outcrop or slope. The system is founded upon the more widely used RMR scheme, which is modified with quantitative guidelines to the rate the influence of adverse joint orientations.

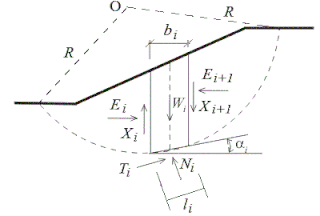
Slope stability analysis is a static or dynamic, analytical or empirical method to evaluate the stability of slopes of soil- and rock-fill dams, embankments, excavated slopes, and natural slopes in soil and rock. It is performed to assess the safe design of a human-made or natural slopes and the equilibrium conditions. Slope stability is the resistance of inclined surface to failure by sliding or collapsing. The main objectives of slope stability analysis are finding endangered areas, investigation of potential failure mechanisms, determination of the slope sensitivity to different triggering mechanisms, designing of optimal slopes with regard to safety, reliability and economics, and designing possible remedial measures, e.g. barriers and stabilization.

The Geological Strength Index (GSI) system, proposed in 1994 by Evert Hoek, is used for the estimation of the rock mass strength and the rock mass deformation modulus.

The Hoek–Brown failure criterion is an empirical stress surface that is used in rock mechanics to predict the failure of rock. The original version of the Hoek–Brown criterion was developed by Evert Hoek and E. T. Brown in 1980 for the design of underground excavations. In 1988, the criterion was extended for applicability to slope stability and surface excavation problems. An update of the criterion was presented in 2002 that included improvements in the correlation between the model parameters and the geological strength index (GSI).

A discontinuity in geotechnical engineering is a plane or surface that marks a change in physical or chemical characteristics in a soil or rock mass. A discontinuity can be, for example, a bedding, schistosity, foliation, joint, cleavage, fracture, fissure, crack, or fault plane. A division is made between mechanical and integral discontinuities. Discontinuities may occur multiple times with broadly the same mechanical characteristics in a discontinuity set, or may be a single discontinuity. A discontinuity makes a soil or rock mass anisotropic.

Within geotechnical engineering, Laubscher developed the Mining Rock Mass Rating (MRMR) system by modifying the Rock Mass Rating (RMR) system of Z. T. Bieniawski. In the MRMR system the stability and support are determined with the following equations:

The Q-system for rock mass classification is developed by Barton, Lien, and Lunde. It expresses the quality of the rock mass in the so-called Q-value, on which design are based and support recommendations for underground excavations.

The shear strength of a discontinuity in a soil or rock mass may have a strong impact on the mechanical behavior of a soil or rock mass. The shear strength of a discontinuity is often considerably lower than the shear strength of the blocks of intact material in between the discontinuities, and therefore influences, for example, tunnel, foundation, or slope engineering, but also the stability of natural slopes. Many slopes, natural and man-made, fail due to a low shear strength of discontinuities in the soil or rock mass in the slope. The deformation characteristics of a soil or rock mass are also influenced by the shear strength of the discontinuities. For example, the modulus of deformation is reduced, and the deformation becomes plastic rather than elastic. This may cause, for example, larger settlement of foundations, which is also permanent even if the load is only temporary. Furthermore, the shear strength of discontinuities influences the stress distribution in a soil or rock mass.

The sliding criterion (discontinuity) is a tool to estimate easily the shear strength properties of a discontinuity in a rock mass based on visual and tactile characterization of the discontinuity. The shear strength of a discontinuity is important in, for example, tunnel, foundation, or slope engineering, but also stability of natural slopes is often governed by the shear strength along discontinuities.

The Q-slope method for rock slope engineering and rock mass classification is developed by Barton and Bar. It expresses the quality of the rock mass for slope stability using the Q-slope value, from which long-term stable, reinforcement-free slope angles can be derived.

Geological structure measurement by LiDAR technology is a remote sensing method applied in structural geology. It enables monitoring and characterisation of rock bodies. This method's typical use is to acquire high resolution structural and deformational data for identifying geological hazards risk, such as assessing rockfall risks or studying pre-earthquake deformation signs.

References

1 2 Bieniawski, Z. T. (1989). Engineering rock mass classifications : a complete manual for engineers and geologists in mining, civil, and petroleum engineering. Wiley-Interscience. pp. 40–47. ISBN 0-471-60172-1.
↑ Celada B, Tardaguila I, Varona P, Rodriguez A, Bieniawski ZT. Innovating tunnel design by an improved experience-based RMR system. In: Proceedings of the World Tunnel Congress – Tunnels for a Better Life. vol 9. Brazil: Foz do Iguaçu; 15 May 2014: 1–9.
1 2 Kundu, J., Sarkar, K., Singh, A.K., & Singh, T., 2020. Continuous functions and a computer application for rock mass rating. Int. J. Rock Mech. Min. Sci. 129.
↑ edumine
↑ Bieniawski, Z. T. (1978). "Determining rock mass deformability". International Journal of Rock Mechanics and Mining Sciences. 15: 335–343.
↑ Bieniawski, Richard Z.T.; Celada, Benjamin; Tardaguila, Isidoro; Rodrigues, Alejandro (February 2012). "Specific energy of excavation in detecting tunnelling conditions ahead of TBMs". Tunnels & Tunneling (2): 65–68.
↑ Aksoy, C. O. (2008). "Review of rock mass rating classification: Historical developments, applications, and restrictions". Journal of Mining Science. 44 (1): 51–63. Bibcode:2008JMinS..44...51A. doi:10.1007/s10913-008-0005-2. S2CID 128817745.
↑ Romana M. (1985). New adjustment ratings for application of Bieniawski classification to slopes. Proc. Int. Symp. on the Role of Rock Mechanics: 49-53.

ASTM (1988). "Standard Guide for using the Rock Mass Rating (RMR) System (Geomechanics Classification) in Engineering Practices." American Society for Testing and Materials, Book of Standards D5878-08, v.04.09, Philadelphia, PA.