Shear band

Last updated

A shear band (or, more generally, a 'strain localization') is a narrow zone of intense shearing strain, usually of plastic nature, developing during severe deformation of ductile materials. As an example, a soil (overconsolidated silty-clay) specimen is shown in Fig. 1, after an axialsymmetric compression test. Initially the sample was cylindrical in shape and, since symmetry was tried to be preserved during the test, the cylindrical shape was maintained for a while during the test and the deformation was homogeneous, but at extreme loading two X-shaped shear bands had formed and the subsequent deformation was strongly localized (see also the sketch on the right of Fig. 1).

Contents

Fig. 1: An initially cylindrical soil sample has been deformed in a setting designed to maintain symmetry (lubricated top and bottom heads have been used). Despite the attempt of preserving symmetry, two X-shaped shear bands are clearly visible (see also the sketch on the right, where initial vertical scratches on the external surface help understanding the shear deformation). X-shaped shear bands in clay.jpg
Fig. 1: An initially cylindrical soil sample has been deformed in a setting designed to maintain symmetry (lubricated top and bottom heads have been used). Despite the attempt of preserving symmetry, two X-shaped shear bands are clearly visible (see also the sketch on the right, where initial vertical scratches on the external surface help understanding the shear deformation).

Materials in which shear bands are observed

Although not observable in brittle materials (for instance glass at room temperature), shear bands or, more generally, ‘localized deformations’ usually develop within a broad range of ductile materials (alloys, metals, granular materials, plastics, polymers, and soils) and even in quasi-brittle materials (concrete, ice, rock, and some ceramics). The relevance of the shear banding phenomena is that they precede failure, since extreme deformations occurring within shear bands lead to intense damage and fracture. Therefore, the formation of shear bands is the key to the understanding of failure in ductile materials, a research topic of great importance for the design of new materials and for the exploiting of existing materials in extreme conditions. As a consequence, localization of deformation has been the focus of an intense research activity since the middle of the 20th century.

Mathematical modeling

Shear band formation is an example of a material instability, corresponding to an abrupt loss of homogeneity of deformation occurring in a solid sample subject to a loading path compatible with continued uniform deformation. In this sense, it may be interpreted as a deformation mechanism ‘alternative’ to a trivial one and therefore a bifurcation or loss of uniqueness of a ‘perfect’ equilibrium path. The distinctive character of this bifurcation is that it may occur even in an infinite body (or under the extreme constraint of smooth contact with a rigid constraint).

Consider an infinite body made up of a nonlinear material, quasi-statically deformed in a way that stress and strain may remain homogeneous. The incremental response of this nonlinear material is assumed for simplicity linear, so that it can be expressed as a relation between a stress increment and a strain increment , through a fourth-order constitutive tensor as

where the fourth-order constitutive tensor depends on the current state, i.e. the current stress, the current strain and, possibly, other constitutive parameters (for instance, hardening variables for metals, or density for granular materials).

Conditions are sought for the emergence of a surface of discontinuity (of unit normal vector ) in the incremental stress and strain. These conditions are identified with the conditions for the occurrence of localization of deformation. In particular, incremental equilibrium requires that the incremental tractions (not the stresses!) remain continuous

(where + and - denote the two sides of the surface) and geometrical compatibility imposes a strain compatibility restriction on the form of incremental strain:

where the symbol denotes tensor product and is a vector defining the deformation discontinuity mode (orthogonal to for incompressible materials). A substitution of the incremental constitutive law (1) and of the strain compatibility ( 3 ) into the continuity of incremental tractions ( 2 ) yields the necessary condition for strain localization:

Since the second-order tensor defined for every vector as

is the so-called 'acoustic tensor', defining the condition of propagation of acceleration waves, we can conclude that the condition for strain localization coincides with the condition of singularity (propagation at null speed) of an acceleration wave. This condition represents the so-called 'loss of ellipticity' of the differential equations governing the rate equilibrium.

State-of-the-art

The state-of-the-art of the research on shear bands is that the phenomenon is well understood from the theoretical [1] [2] [3] [4] [5] [6] [7] [8] [9] and experimental [10] [11] [12] [13] point of view and available constitutive models give nice qualitative predictions, although quantitative predictions are often poor. [14] Moreover, great progresses have been made on numerical simulations, [15] [16] [17] [18] so that shear band nucleation and propagation in relatively complex situations can be traced numerically with finite element models, although still at the cost of a great computational effort. Of further interest are simulations that reveal the crystallographic orientation dependence of shear banding in single crystal and polycrystals. These simulations show that certain orientations are much more prone to undergo shear localization than others. [19]

Shear banding and crystallographic texture

Most polycrystalline metals and alloys usually deform via shear caused by dislocations, twins, and / or shear bands. This leads to pronounced plastic anisotropy at the grain scale and to preferred grain orientation distributions, i.e. crystallographic textures. Cold rolling textures of most face centered cubic metals and alloys for instance range between two types, i.e. the brass-type texture and the copper-type texture. The stacking fault energy plays an important role for the prevailing mechanisms of plastic deformation and the resultant textures. For aluminum and other fcc materials with high SFE, dislocation glide is the main mechanism during cold rolling and the {112}<111> (copper) and {123}<634> (S) texture components (copper-type textures) are developed. In contrast, in Cu–30 wt.% Zn (alpha-brass) and related metals and alloys with low SFE, mechanical twinning and shear banding occur together with dislocation glide as main deformation carriers, particularly at large plastic deformations. The resulting rolling textures are characterized by the {011}<211> (brass) and {01 1}<100> (Goss) texture components (brass-type texture). In either case non-crystallographic shear banding plays an essential role for the specific type of deformation texture evolved. [20] [21]

A perturbative approach to analyze shear band emergence

Closed-form solutions disclosing the shear band emergence can be obtained through the perturbative approach, [22] [23] consisting in the superimposition of a perturbation field upon an unperturbed deformed state. In particular, an infinite, incompressible, nonlinear elastic material, homogeneously deformed under the plane strain condition can be perturbed through superposition of concentrated forces or by the presence of cracks or rigid line inclusions.

It has been shown that, when the unperturbed state is taken close to the localization condition (4), the perturbed fields self-arrange in the form of localized fields, taking extreme values in the neighbourhood of the introduced perturbation and focussed along the shear bands directions. In particular, in the case of cracks and rigid line inclusions such shear bands emerge from the linear inclusion tips. [24]

Within the perturbative approach, an incremental model for a shear band of finite length has been introduced [25] prescribing the following conditions along its surface:

Employing this model, the following main features of shear banding have been demonstrated:

  1. similarly to fracture mechanics, a square-root singularity in the stress/deformation fields develops at the shear band tips;
  2. in presence of a shear band, the strain field is localized and strongly focussed in the direction aligned parallel to the shear band;
  3. since the energy release rate associated to the shear band growth blows up to infinity near the localization condition (4), shear bands represent preferential failure modes.

See also

Related Research Articles

Rheology is the study of the flow of matter, primarily in a fluid state, but also as "soft solids" or solids under conditions in which they respond with plastic flow rather than deforming elastically in response to an applied force. Rheology is a branch of physics, and it is the science that deals with the deformation and flow of materials, both solids and liquids.

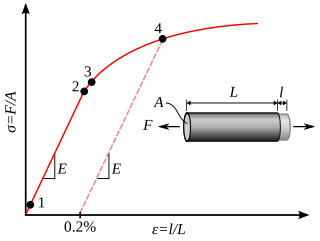
<span class="mw-page-title-main">Plasticity (physics)</span> Non-reversible deformation of a solid material in response to applied forces

In physics and materials science, plasticity is the ability of a solid material to undergo permanent deformation, a non-reversible change of shape in response to applied forces. For example, a solid piece of metal being bent or pounded into a new shape displays plasticity as permanent changes occur within the material itself. In engineering, the transition from elastic behavior to plastic behavior is known as yielding.

In physics and materials science, elasticity is the ability of a body to resist a distorting influence and to return to its original size and shape when that influence or force is removed. Solid objects will deform when adequate loads are applied to them; if the material is elastic, the object will return to its initial shape and size after removal. This is in contrast to plasticity, in which the object fails to do so and instead remains in its deformed state.

In materials science and continuum mechanics, viscoelasticity is the property of materials that exhibit both viscous and elastic characteristics when undergoing deformation. Viscous materials, like water, resist shear flow and strain linearly with time when a stress is applied. Elastic materials strain when stretched and immediately return to their original state once the stress is removed.

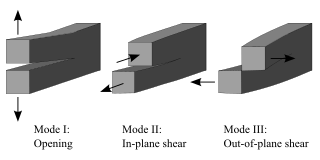
<span class="mw-page-title-main">Fracture mechanics</span> Study of propagation of cracks in materials

Fracture mechanics is the field of mechanics concerned with the study of the propagation of cracks in materials. It uses methods of analytical solid mechanics to calculate the driving force on a crack and those of experimental solid mechanics to characterize the material's resistance to fracture.

In physics and engineering, a constitutive equation or constitutive relation is a relation between two or more physical quantities that is specific to a material or substance or field, and approximates its response to external stimuli, usually as applied fields or forces. They are combined with other equations governing physical laws to solve physical problems; for example in fluid mechanics the flow of a fluid in a pipe, in solid state physics the response of a crystal to an electric field, or in structural analysis, the connection between applied stresses or loads to strains or deformations.

<span class="mw-page-title-main">Photoelasticity</span> Change in optical properties of a material due to stress

In materials science, photoelasticity describes changes in the optical properties of a material under mechanical deformation. It is a property of all dielectric media and is often used to experimentally determine the stress distribution in a material.

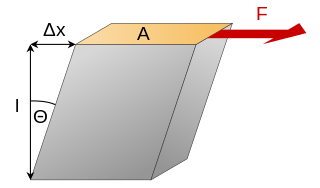
<span class="mw-page-title-main">Shear modulus</span> Ratio of shear stress to shear strain

In materials science, shear modulus or modulus of rigidity, denoted by G, or sometimes S or μ, is a measure of the elastic shear stiffness of a material and is defined as the ratio of shear stress to the shear strain:

<span class="mw-page-title-main">Lüders band</span> Bands of plastic deformation in metals

Lüders bands, is type of slip bands in metals or stretcher-strain marks which are formed due to localized bands of plastic deformation in metals experiencing tensile stresses, common to low-carbon steels and certain Al-Mg alloys. First reported by Guillaume Piobert, and later by W. Lüders, the mechanism that stimulates their appearance is known as dynamic strain aging, or the inhibition of dislocation motion by interstitial atoms, around which "atmospheres" or "zones" naturally congregate.

The J-integral represents a way to calculate the strain energy release rate, or work (energy) per unit fracture surface area, in a material. The theoretical concept of J-integral was developed in 1967 by G. P. Cherepanov and independently in 1968 by James R. Rice, who showed that an energetic contour path integral was independent of the path around a crack.

The material point method (MPM) is a numerical technique used to simulate the behavior of solids, liquids, gases, and any other continuum material. Especially, it is a robust spatial discretization method for simulating multi-phase (solid-fluid-gas) interactions. In the MPM, a continuum body is described by a number of small Lagrangian elements referred to as 'material points'. These material points are surrounded by a background mesh/grid that is used to calculate terms such as the deformation gradient. Unlike other mesh-based methods like the finite element method, finite volume method or finite difference method, the MPM is not a mesh based method and is instead categorized as a meshless/meshfree or continuum-based particle method, examples of which are smoothed particle hydrodynamics and peridynamics. Despite the presence of a background mesh, the MPM does not encounter the drawbacks of mesh-based methods which makes it a promising and powerful tool in computational mechanics.

In geology, a deformation mechanism is a process occurring at a microscopic scale that is responsible for changes in a material's internal structure, shape and volume. The process involves planar discontinuity and/or displacement of atoms from their original position within a crystal lattice structure. These small changes are preserved in various microstructures of materials such as rocks, metals and plastics, and can be studied in depth using optical or digital microscopy.

<span class="mw-page-title-main">Viscoplasticity</span> Theory in continuum mechanics

Viscoplasticity is a theory in continuum mechanics that describes the rate-dependent inelastic behavior of solids. Rate-dependence in this context means that the deformation of the material depends on the rate at which loads are applied. The inelastic behavior that is the subject of viscoplasticity is plastic deformation which means that the material undergoes unrecoverable deformations when a load level is reached. Rate-dependent plasticity is important for transient plasticity calculations. The main difference between rate-independent plastic and viscoplastic material models is that the latter exhibit not only permanent deformations after the application of loads but continue to undergo a creep flow as a function of time under the influence of the applied load.

The acoustoelastic effect is how the sound velocities of an elastic material change if subjected to an initial static stress field. This is a non-linear effect of the constitutive relation between mechanical stress and finite strain in a material of continuous mass. In classical linear elasticity theory small deformations of most elastic materials can be described by a linear relation between the applied stress and the resulting strain. This relationship is commonly known as the generalised Hooke's law. The linear elastic theory involves second order elastic constants and yields constant longitudinal and shear sound velocities in an elastic material, not affected by an applied stress. The acoustoelastic effect on the other hand include higher order expansion of the constitutive relation between the applied stress and resulting strain, which yields longitudinal and shear sound velocities dependent of the stress state of the material. In the limit of an unstressed material the sound velocities of the linear elastic theory are reproduced.

Polymer fracture is the study of the fracture surface of an already failed material to determine the method of crack formation and extension in polymers both fiber reinforced and otherwise. Failure in polymer components can occur at relatively low stress levels, far below the tensile strength because of four major reasons: long term stress or creep rupture, cyclic stresses or fatigue, the presence of structural flaws and stress-cracking agents. Formations of submicroscopic cracks in polymers under load have been studied by x ray scattering techniques and the main regularities of crack formation under different loading conditions have been analyzed. The low strength of polymers compared to theoretically predicted values are mainly due to the many microscopic imperfections found in the material. These defects namely dislocations, crystalline boundaries, amorphous interlayers and block structure can all lead to the non-uniform distribution of mechanical stress.

<span class="mw-page-title-main">Rock mass plasticity</span>

Plasticity theory for rocks is concerned with the response of rocks to loads beyond the elastic limit. Historically, conventional wisdom has it that rock is brittle and fails by fracture while plasticity is identified with ductile materials. In field scale rock masses, structural discontinuities exist in the rock indicating that failure has taken place. Since the rock has not fallen apart, contrary to expectation of brittle behavior, clearly elasticity theory is not the last word.

Drucker stability refers to a set of mathematical criteria that restrict the possible nonlinear stress-strain relations that can be satisfied by a solid material. The postulates are named after Daniel C. Drucker. A material that does not satisfy these criteria is often found to be unstable in the sense that application of a load to a material point can lead to arbitrary deformations at that material point unless an additional length or time scale is specified in the constitutive relations.

In materials science, toughening refers to the process of making a material more resistant to the propagation of cracks. When a crack propagates, the associated irreversible work in different materials classes is different. Thus, the most effective toughening mechanisms differ among different materials classes. The crack tip plasticity is important in toughening of metals and long-chain polymers. Ceramics have limited crack tip plasticity and primarily rely on different toughening mechanisms.

Crystal plasticity is a mesoscale computational technique that takes into account crystallographic anisotropy in modelling the mechanical behaviour of polycrystalline materials. The technique has typically been used to study deformation through the process of slip, however, there are some flavors of crystal plasticity that can incorporate other deformation mechanisms like twinning and phase transformations. Crystal plasticity is used to obtain the relationship between stress and strain that also captures the underlying physics at the crystal level. Hence, it can be used to predict not just the stress-strain response of a material, but also the texture evolution, micromechanical field distributions, and regions of strain localisation. The two widely used formulations of crystal plasticity are the one based on the finite element method known as Crystal Plasticity Finite Element Method (CPFEM), which is developed based on the finite strain formulation for the mechanics, and a spectral formulation which is more computationally efficient due to the fast Fourier transform, but is based on the small strain formulation for the mechanics.

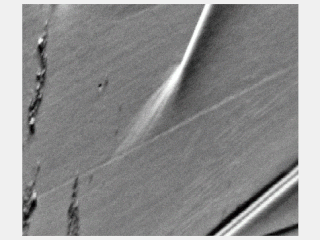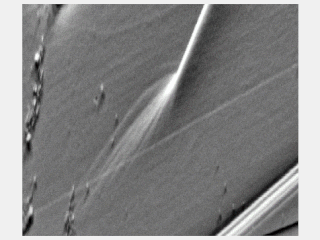
<span class="mw-page-title-main">Slip bands in metals</span> Deformation mechanism in crystallines

Slip bands or stretcher-strain marks are localized bands of plastic deformation in metals experiencing stresses. Formation of slip bands indicates a concentrated unidirectional slip on certain planes causing a stress concentration. Typically, slip bands induce surface steps and a stress concentration which can be a crack nucleation site. Slip bands extend until impinged by a boundary, and the generated stress from dislocations pile-up against that boundary will either stop or transmit the operating slip depending on its (mis)orientation.

References

  1. ↑ Bigoni, D. Nonlinear Solid Mechanics: Bifurcation Theory and Material Instability. Cambridge University Press, 2012. ISBN   9781107025417.
  2. ↑ Bigoni, Davide; Hueckel, Tomasz (1991). "Uniqueness and localization—I. Associative and non-associative elastoplasticity". International Journal of Solids and Structures. Elsevier BV. 28 (2): 197–213. doi:10.1016/0020-7683(91)90205-t. ISSN   0020-7683.
  3. ↑ Biot, M.A. (1965) Mechanics of incremental deformations. New York, Wiley.
  4. ↑ Hill, R. (1962). "Acceleration waves in solids". Journal of the Mechanics and Physics of Solids. Elsevier BV. 10 (1): 1–16. Bibcode:1962JMPSo..10....1H. doi:10.1016/0022-5096(62)90024-8. ISSN   0022-5096.
  5. ↑ Mandel, J. (1962) Ondes plastiques dans un milieu indéfini à trois dimensions. J. de Mécanique 1, 3-30.
  6. ↑ Nadai, A. (1950) Theory of flow and fracture of solids. McGraw-Hill, New York.
  7. ↑ Rice, J. R. (1977) The localization of plastic deformation. In Koiter, W.T., ed., Theoretical and Applied Mechanics. Amsterdam, North-Holland. 207-220.
  8. ↑ Rudnicki, J.W.; Rice, J.R. (1975). "Conditions for the localization of deformation in pressure-sensitive dilatant materials" (PDF). Journal of the Mechanics and Physics of Solids. Elsevier BV. 23 (6): 371–394. Bibcode:1975JMPSo..23..371R. doi:10.1016/0022-5096(75)90001-0. ISSN   0022-5096.
  9. ↑ Thomas, T.Y. (1961) Plastic flows and fracture of solids. Academic Press, New York.
  10. ↑ Desrues, J.; Lanier, J.; Stutz, P. (1985). "Localization of the deformation in tests on sand sample". Engineering Fracture Mechanics. Elsevier BV. 21 (4): 909–921. doi:10.1016/0013-7944(85)90097-9. ISSN   0013-7944.
  11. ↑ Knodel, PC; Drescher, A; Vardoulakis, I; Han, C (1990). "A Biaxial Apparatus for Testing Soils". Geotechnical Testing Journal. ASTM International. 13 (3): 226–234. doi:10.1520/gtj10161j. ISSN   0149-6115.
  12. ↑ Poirier, C.; Ammi, M.; Bideau, D.; Troadec, J. P. (1992-01-13). "Experimental study of the geometrical effects in the localization of deformation". Physical Review Letters. American Physical Society (APS). 68 (2): 216–219. Bibcode:1992PhRvL..68..216P. doi:10.1103/physrevlett.68.216. ISSN   0031-9007. PMID   10045565.
  13. ↑ Vardoulakis, I. (1983). "Rigid granular plasticity model and bifurcation in the triaxial test". Acta Mechanica. Springer Science and Business Media LLC. 49 (1–2): 57–79. doi:10.1007/bf01181755. ISSN   0001-5970. S2CID   120588998.
  14. ↑ Gajo, A., Bigoni, D. and Muir Wood, D. (2004) Multiple shear band development and related instabilities in granular materials. J. Mech. Phys. Solids 52, 2683-2724.
  15. ↑ Leroy, Y.; Ortiz, M. (1990). "Finite element analysis of transient strain localization phenomena in frictional solids". International Journal for Numerical and Analytical Methods in Geomechanics. Wiley. 14 (2): 93–124. Bibcode:1990IJNAM..14...93L. doi:10.1002/nag.1610140203. ISSN   0363-9061.
  16. ↑ Nacar, A.; Needleman, A.; Ortiz, M. (1989). "A finite element method for analyzing localization in rate dependent solids at finite strains". Computer Methods in Applied Mechanics and Engineering. Elsevier BV. 73 (3): 235–258. Bibcode:1989CMAME..73..235N. doi:10.1016/0045-7825(89)90067-4. ISSN   0045-7825.
  17. ↑ Petryk, H.; Thermann, K. (2002). "Post-critical plastic deformation in incrementally nonlinear materials". Journal of the Mechanics and Physics of Solids. Elsevier BV. 50 (5): 925–954. Bibcode:2002JMPSo..50..925P. doi:10.1016/s0022-5096(01)00131-4. ISSN   0022-5096.
  18. ↑ Loret, Benjamin; Prevost, Jean H. (1990). "Dynamic strain localization in elasto-(visco-)plastic solids, Part 1. General formulation and one-dimensional examples". Computer Methods in Applied Mechanics and Engineering. Elsevier BV. 83 (3): 247–273. Bibcode:1990CMAME..83..247L. doi:10.1016/0045-7825(90)90073-u. ISSN   0045-7825.
  19. ↑ Jia, N.; Roters, F.; Eisenlohr, P.; Kords, C.; Raabe, D. (2012). "Non-crystallographic shear banding in crystal plasticity FEM simulations: Example of texture evolution in α-brass". Acta Materialia. Elsevier BV. 60 (3): 1099–1115. Bibcode:2012AcMat..60.1099J. doi:10.1016/j.actamat.2011.10.047. ISSN   1359-6454.
  20. ↑ Jia, N.; Roters, F.; Eisenlohr, P.; Raabe, D.; Zhao, X. (2013). "Simulation of shear banding in heterophase co-deformation: Example of plane strain compressed Cu–Ag and Cu–Nb metal matrix composites". Acta Materialia. Elsevier BV. 61 (12): 4591–4606. Bibcode:2013AcMat..61.4591J. doi:10.1016/j.actamat.2013.04.029. ISSN   1359-6454.
  21. ↑ Jia, N.; Eisenlohr, P.; Roters, F.; Raabe, D.; Zhao, X. (2012). "Orientation dependence of shear banding in face-centered-cubic single crystals". Acta Materialia. Elsevier BV. 60 (8): 3415–3434. Bibcode:2012AcMat..60.3415J. doi:10.1016/j.actamat.2012.03.005. ISSN   1359-6454.
  22. ↑ Bigoni, D. and Capuani, D. (2002) Green's function for incremental nonlinear elasticity: shear bands and boundary integral formulation. Journ. Mech. Phys. Sol. 50, 471-500.
  23. ↑ Bigoni, D. and Capuani, D. (2005) Time-harmonic Green's function and boundary integral formulation for incremental nonlinear elasticity: dynamics of wave patterns and shear bands. Journ. Mech. Phys. Sol. 53, 1163-1187.
  24. ↑ Dal Corso F. and Bigoni D. (2009) The interactions between shear bands and rigid lamellar inclusions in a ductile metal matrix. Proc. R. Soc. Lond. A, 465, 143-163.
  25. ↑ Bigoni, D. and Dal Corso, F. (2008) The unrestrainable growth of a shear band in a prestressed material. Proc. R. Soc. Lond. A, 464, 2365-2390.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.