Sobolev mapping

Last updated April 28, 2024

In mathematics, a Sobolev mapping is a mapping between manifolds which has smoothness in some sense. Sobolev mappings appear naturally in manifold-constrained problems in the calculus of variations and partial differential equations, including the theory of harmonic maps.

Definition

Given Riemannian manifolds $M$ and $N$ , which is assumed by Nash's smooth embedding theorem without loss of generality to be isometrically embedded into $\mathbb {R} ^{\nu }$ as ^[1]^[2]

W^{s,p}(M,N):=\{u\in W^{s,p}(M,\mathbb {R} ^{\nu })\,\vert \,u(x)\in N{\text{ for almost every }}x\in M\}.

First-order ( $s=1$ ) Sobolev mappings can also be defined in the context of metric spaces.^[3]^[4]

Approximation

The strong approximation problem consists in determining whether smooth mappings from $M$ to $N$ are dense in $W^{s,p}(M,N)$ with respect to the norm topology. When $sp>\dim M$ , Morrey's inequality implies that Sobolev mappings are continuous and can thus be strongly approximated by smooth maps. When $sp=\dim M$ , Sobolev mappings have vanishing mean oscillation ^[5] and can thus be approximated by smooth maps.^[6]

When $sp<\dim M$ , the question of density is related to obstruction theory: $C^{\infty }(M,N)$ is dense in $W^{1,p}(M,N)$ if and only if every continuous mapping on a from a $\lfloor p\rfloor$ –dimensional triangulation of $M$ into $N$ is the restriction of a continuous map from $M$ to $N$ .^[7]^[2]

The problem of finding a sequence of weak approximation of maps in $W^{1,p}(M,N)$ is equivalent to the strong approximation when $p$ is not an integer.^[7] When $p$ is an integer, a necessary condition is that the restriction to a $\lfloor p-1\rfloor$ -dimensional triangulation of every continuous mapping from a $\lfloor p\rfloor$ –dimensional triangulation of $M$ into $N$ coincides with the restriction a continuous map from $M$ to $N$ .^[2] When $p=2$ , this condition is sufficient^[8]. For $W^{1,3}(M,\mathbb {S} ^{2})$ with $\dim M\geq 4$ , this condition is not sufficient.^[9]

Homotopy

The homotopy problem consists in describing and classifying the path-connected components of the space $W^{s,p}(M,N)$ endowed with the norm topology. When $0<s\leq 1$ and $\dim M\leq sp$ , then the path-connected components of $W^{s,p}(M,N)$ are essentially the same as the path-connected components of $C(M,N)$ : two maps in $W^{s,p}(M,N)\cap C(M,N)$ are connected by a path in $W^{s,p}(M,N)$ if and only if they are connected by a path in $C(M,N)$ , any path-connected component of $W^{s,p}(M,N)$ and any path-connected component of $C(M,N)$ intersects $W^{s,p}(M,N)\cap C(M,N)$ non trivially.^[10]^[11]^[12] When $\dim M>p$ , two maps in $W^{1,p}(M,N)$ are connected by a continuous path in $W^{1,p}(M,N)$ if and only if their restrictions to a generic $\lfloor p-1\rfloor$ -dimensional triangulation are homotopic.^[2]^{: th. 1.1}

Extension of traces

The classical trace theory states that any Sobolev map $u\in W^{1,p}(M,N)$ has a trace $Tu\in W^{1-1/p,p}(\partial M,N)$ and that when $N=\mathbb {R}$ , the trace operator is onto. The proof of the surjectivity being based on an averaging argument, the result does not readily extend to Sobolev mappings. The trace operator is known to be onto when $\pi _{1}(N)\simeq \dotsb \pi _{\lfloor p-1\rfloor }(N)\simeq \{0\}$ ^[13] or when $p\geq 3$ , $\pi _{1}(N)$ is finite and $\pi _{2}(N)\simeq \dotsb \pi _{\lfloor p-1\rfloor }(N)\simeq \{0\}$ .^[14] The surjectivity of the trace operator fails if $\pi _{\lfloor p-1\rfloor }(N)\not \simeq \{0\}$ ^[13]^[15] or if $\pi _{\ell }(N)$ is infinite for some $\ell \in \{1,\dotsc ,\lfloor p-1\rfloor \}$ .^[14]^[16]

Lifting

Given a covering map ${\displaystyle \pi$ , the lifting problem asks whether any map $u\in W^{s,p}(M,N)$ can be written as $u=\pi \circ {\tilde {u}}$ for some ${\tilde {u}}\in W^{s,p}(M,{\tilde {N}})$ , as it is the case for continuous or smooth $u$ and ${\tilde {u}}$ when $M$ is simply-connected in the classical lifting theory. If the domain $M$ is simply connected, any map $u\in W^{s,p}(M,N)$ can be written as $u=\pi \circ {\tilde {u}}$ for some ${\tilde {u}}\in W^{s,p}(M,N)$ when $sp\geq \dim M$ ,^[17]^[18] when $s\geq 1$ and $2\leq sp<\dim M$ ^[19]^[18] and when $N$ is compact, $0<s<1$ and $2\leq sp<\dim M$ .^[20] There is a topological obstruction to the lifting when $sp<2$ and an analytical obstruction when $1\leq sp<\dim M$ .^[17]^[18]

Related Research Articles

In mathematics, a diffeomorphism is an isomorphism of differentiable manifolds. It is an invertible function that maps one differentiable manifold to another such that both the function and its inverse are continuously differentiable.

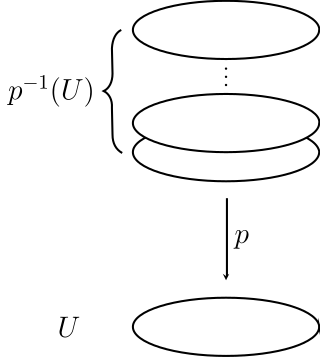
In topology, a covering or covering projection is a map between topological spaces that, intuitively, locally acts like a projection of multiple copies of a space onto itself. In particular, coverings are special types of local homeomorphisms. If $is a covering, is said to be a covering space or cover of, and is said to be the base of the covering, or simply the base . By abuse of terminology, and may sometimes be called covering spaces as well. Since coverings are local homeomorphisms, a covering space is a special kind of étale space.$

In mathematics, a vector bundle is a topological construction that makes precise the idea of a family of vector spaces parameterized by another space $: to every point of the space we associate a vector space in such a way that these vector spaces fit together to form another space of the same kind as, which is then called a vector bundle over .$

<span class="mw-page-title-main">Fiber bundle</span> Continuous surjection satisfying a local triviality condition

In mathematics, and particularly topology, a fiber bundle is a space that is locally a product space, but globally may have a different topological structure. Specifically, the similarity between a space $and a product space is defined using a continuous surjective map, that in small regions of behaves just like a projection from corresponding regions of to The map called the projection or submersion of the bundle, is regarded as part of the structure of the bundle. The space is known as the total space of the fiber bundle, as the base space, and the fiber .$

In Riemannian geometry, the sectional curvature is one of the ways to describe the curvature of Riemannian manifolds. The sectional curvature K(σ_p) depends on a two-dimensional linear subspace σ_p of the tangent space at a point p of the manifold. It can be defined geometrically as the Gaussian curvature of the surface which has the plane σ_p as a tangent plane at p, obtained from geodesics which start at p in the directions of σ_p. The sectional curvature is a real-valued function on the 2-Grassmannian bundle over the manifold.

In mathematics, a principal bundle is a mathematical object that formalizes some of the essential features of the Cartesian product $of a space with a group . In the same way as with the Cartesian product, a principal bundle is equipped with$

An action of $on, analogous to for a product space.$
A projection onto $. For a product space, this is just the projection onto the first factor, .$

In mathematics, a submersion is a differentiable map between differentiable manifolds whose differential is everywhere surjective. This is a basic concept in differential topology. The notion of a submersion is dual to the notion of an immersion.

In the mathematical field of topology, a section of a fiber bundle $is a continuous right inverse of the projection function . In other words, if is a fiber bundle over a base space, :$

<span class="mw-page-title-main">Foliation</span> In mathematics, a type of equivalence relation on an n-manifold

In mathematics, a foliation is an equivalence relation on an n-manifold, the equivalence classes being connected, injectively immersed submanifolds, all of the same dimension p, modeled on the decomposition of the real coordinate space Rⁿ into the cosets x + R^p of the standardly embedded subspace R^p. The equivalence classes are called the leaves of the foliation. If the manifold and/or the submanifolds are required to have a piecewise-linear, differentiable, or analytic structure then one defines piecewise-linear, differentiable, or analytic foliations, respectively. In the most important case of differentiable foliation of class C^r it is usually understood that r ≥ 1. The number p is called the dimension of the foliation and q = n − p is called its codimension.

In mathematics, and especially differential geometry and gauge theory, a connection is a device that defines a notion of parallel transport on the bundle; that is, a way to "connect" or identify fibers over nearby points. A principal G-connection on a principal G-bundle $over a smooth manifold is a particular type of connection which is compatible with the action of the group .$

In mathematics, a Sobolev space is a vector space of functions equipped with a norm that is a combination of L^p-norms of the function together with its derivatives up to a given order. The derivatives are understood in a suitable weak sense to make the space complete, i.e. a Banach space. Intuitively, a Sobolev space is a space of functions possessing sufficiently many derivatives for some application domain, such as partial differential equations, and equipped with a norm that measures both the size and regularity of a function.

<span class="mw-page-title-main">Holonomy</span> Concept in differential geometry

In differential geometry, the holonomy of a connection on a smooth manifold is a general geometrical consequence of the curvature of the connection measuring the extent to which parallel transport around closed loops fails to preserve the geometrical data being transported. For flat connections, the associated holonomy is a type of monodromy and is an inherently global notion. For curved connections, holonomy has nontrivial local and global features.

In mathematics, in the subfield of geometric topology, the mapping class group is an important algebraic invariant of a topological space. Briefly, the mapping class group is a certain discrete group corresponding to symmetries of the space.

In differential geometry, a field in mathematics, a Poisson manifold is a smooth manifold endowed with a Poisson structure. The notion of Poisson manifold generalises that of symplectic manifold, which in turn generalises the phase space from Hamiltonian mechanics.

In mathematics, specifically algebraic topology, an Eilenberg–MacLane space is a topological space with a single nontrivial homotopy group.

In differential geometry, in the category of differentiable manifolds, a fibered manifold is a surjective submersion

In mathematics, the Abel–Jacobi map is a construction of algebraic geometry which relates an algebraic curve to its Jacobian variety. In Riemannian geometry, it is a more general construction mapping a manifold to its Jacobi torus. The name derives from the theorem of Abel and Jacobi that two effective divisors are linearly equivalent if and only if they are indistinguishable under the Abel–Jacobi map.

In mathematics, specifically in surgery theory, the surgery obstructions define a map $from the normal invariants to the L-groups which is in the first instance a set-theoretic map with the following property when :$

In mathematics, convenient vector spaces are locally convex vector spaces satisfying a very mild completeness condition.

This is a glossary of properties and concepts in algebraic topology in mathematics.

References

↑ Mironescu, Petru (2007). "Sobolev maps on manifolds: degree, approximation, lifting" (PDF). Contemporary Mathematics. 446: 413–436. doi:10.1090/conm/446/08642. ISBN 9780821841907.
1 2 3 4 Hang, Fengbo; Lin, Fanghua (2003). "Topology of sobolev mappings, II". Acta Mathematica. 191 (1): 55–107. doi: 10.1007/BF02392696 . S2CID 121520479.
↑ Chiron, David (August 2007). "On the definitions of Sobolev and BV spaces into singular spaces and the trace problem". Communications in Contemporary Mathematics. 09 (4): 473–513. doi:10.1142/S0219199707002502.
↑ Hajłasz, Piotr (2009). "Sobolev Mappings between Manifolds and Metric Spaces". Sobolev Spaces in Mathematics I. International Mathematical Series. 8: 185–222. doi:10.1007/978-0-387-85648-3_7. ISBN 978-0-387-85647-6.
↑ Brezis, H.; Nirenberg, L. (September 1995). "Degree theory and BMO; part I: Compact manifolds without boundaries". Selecta Mathematica. 1 (2): 197–263. doi:10.1007/BF01671566. S2CID 195270732.
↑ Schoen, Richard; Uhlenbeck, Karen (1 January 1982). "A regularity theory for harmonic maps". Journal of Differential Geometry. 17 (2). doi: 10.4310/jdg/1214436923 .
1 2 Bethuel, Fabrice (1991). "The approximation problem for Sobolev maps between two manifolds". Acta Mathematica. 167: 153–206. doi: 10.1007/BF02392449 . S2CID 122996551.
↑ Pakzad, M.R.; Rivière, T. (February 2003). "Weak density of smooth maps for the Dirichlet energy between manifolds". Geometric and Functional Analysis. 13 (1): 223–257. doi:10.1007/s000390300006. S2CID 121794503.
↑ Bethuel, Fabrice (February 2020). "A counterexample to the weak density of smooth maps between manifolds in Sobolev spaces". Inventiones Mathematicae. 219 (2): 507–651. arXiv: 1401.1649 . Bibcode:2020InMat.219..507B. doi:10.1007/s00222-019-00911-3. S2CID 119627475.
↑ Brezis, Haı̈m; Li, YanYan (September 2000). "Topology and Sobolev spaces". Comptes Rendus de l'Académie des Sciences - Series I - Mathematics. 331 (5): 365–370. Bibcode:2000CRASM.331..365B. doi:10.1016/S0764-4442(00)01656-6.
↑ Brezis, Haim; Li, Yanyan (July 2001). "Topology and Sobolev Spaces". Journal of Functional Analysis. 183 (2): 321–369. doi: 10.1006/jfan.2000.3736 .
↑ Bousquet, Pierre (February 2008). "Fractional Sobolev spaces and topology". Nonlinear Analysis: Theory, Methods & Applications. 68 (4): 804–827. doi:10.1016/j.na.2006.11.038.
1 2 Hardt, Robert; Lin, Fang-Hua (September 1987). "Mappings minimizing the L^p norm of the gradient". Communications on Pure and Applied Mathematics. 40 (5): 555–588. doi:10.1002/cpa.3160400503.
1 2 Mironescu, Petru; Van Schaftingen, Jean (9 July 2021). "Trace theory for Sobolev mappings into a manifold". Annales de la Faculté des sciences de Toulouse: Mathématiques. 30 (2): 281–299. arXiv: 2001.02226 . doi:10.5802/afst.1675. S2CID 210023485.
↑ Bethuel, Fabrice; Demengel, Françoise (October 1995). "Extensions for Sobolev mappings between manifolds". Calculus of Variations and Partial Differential Equations. 3 (4): 475–491. doi:10.1007/BF01187897. S2CID 121749565.
↑ Bethuel, Fabrice (March 2014). "A new obstruction to the extension problem for Sobolev maps between manifolds". Journal of Fixed Point Theory and Applications. 15 (1): 155–183. arXiv: 1402.4614 . doi:10.1007/s11784-014-0185-0. S2CID 119614310.
1 2 Bourgain, Jean; Brezis, Haim; Mironescu, Petru (December 2000). "Lifting in Sobolev spaces". Journal d'Analyse Mathématique . 80 (1): 37–86. doi: 10.1007/BF02791533 .
1 2 3 Bethuel, Fabrice; Chiron, David (2007). "Some questions related to the lifting problem in Sobolev spaces". Contemporary Mathematics. 446: 125–152. doi:10.1090/conm/446/08628. ISBN 9780821841907.
↑ Bethuel, Fabrice; Zheng, Xiaomin (September 1988). "Density of smooth functions between two manifolds in Sobolev spaces". Journal of Functional Analysis. 80 (1): 60–75. doi: 10.1016/0022-1236(88)90065-1 .
↑ Mironescu, Petru; Van Schaftingen, Jean (7 September 2021). "Lifting in compact covering spaces for fractional Sobolev mappings". Analysis & PDE. 14 (6): 1851–1871. arXiv: 1907.01373 . doi:10.2140/apde.2021.14.1851. S2CID 195776361.