In a computer's central processing unit (CPU), the accumulator is a register in which intermediate arithmetic logic unit results are stored.
An adding machine is a class of mechanical calculator, usually specialized for bookkeeping calculations. In the United States, the earliest adding machines were usually built to read in dollars and cents. Adding machines were ubiquitous office equipment until they were phased out in favor of electronic calculators in the 1970s and by personal computers beginning in about 1985. The older adding machines were rarely seen in American office settings by the year 2000.
A one-instruction set computer (OISC), sometimes referred to as an ultimate reduced instruction set computer (URISC), is an abstract machine that uses only one instruction – obviating the need for a machine language opcode. With a judicious choice for the single instruction and given arbitrarily many resources, an OISC is capable of being a universal computer in the same manner as traditional computers that have multiple instructions. OISCs have been recommended as aids in teaching computer architecture and have been used as computational models in structural computing research. The first carbon nanotube computer is a 1-bit one-instruction set computer.
Napier's bones is a manually operated calculating device created by John Napier of Merchiston, Scotland for the calculation of products and quotients of numbers. The method was based on lattice multiplication, and also called rabdology, a word invented by Napier. Napier published his version in 1617. It was printed in Edinburgh and dedicated to his patron Alexander Seton.
Two's complement is the most common method of representing signed integers on computers, and more generally, fixed point binary values. Two's complement uses the binary digit with the greatest value as the sign to indicate whether the binary number is positive or negative; when the most significant bit is 1 the number is signed as negative and when the most significant bit is 0 the number is signed as positive. As a result, non-negative numbers are represented as themselves: 6 is 0110, zero is 0000, and -6 is 1010. Note that while the number of binary bits is fixed throughout a computation it is otherwise arbitrary.
In arithmetic, long division is a standard division algorithm suitable for dividing multi-digit Hindu-Arabic numerals that is simple enough to perform by hand. It breaks down a division problem into a series of easier steps.
In mathematics and computing, the method of complements is a technique to encode a symmetric range of positive and negative integers in a way that they can use the same algorithm for addition throughout the whole range. For a given number of places half of the possible representations of numbers encode the positive numbers, the other half represents their respective additive inverses. The pairs of mutually additive inverse numbers are called complements. Thus subtraction of any number is implemented by adding its complement. Changing the sign of any number is encoded by generating its complement, which can be done by a very simple and efficient algorithm. This method was commonly used in mechanical calculators and is still used in modern computers. The generalized concept of the radix complement is also valuable in number theory, such as in Midy's theorem.
Wilhelm Schickard was a German professor of Hebrew and astronomy who became famous in the second part of the 20th century after Franz Hammer, a biographer of Johannes Kepler, claimed that the drawings of a calculating clock, predating the public release of Pascal's calculator by twenty years, had been discovered in two unknown letters written by Schickard to Johannes Kepler in 1623 and 1624.
The Curta is a hand-held mechanical calculator designed by Curt Herzstark. It is known for its extremely compact design: a small cylinder that fits in the palm of the hand. It was affectionately known as the "pepper grinder" or "peppermill" due to its shape and means of operation; its superficial resemblance to a certain type of hand grenade also earned it the nickname "math grenade".
A mechanical calculator, or calculating machine, is a mechanical device used to perform the basic operations of arithmetic automatically, or (historically) a simulation such as an analog computer or a slide rule. Most mechanical calculators were comparable in size to small desktop computers and have been rendered obsolete by the advent of the electronic calculator and the digital computer.
Mental calculation consists of arithmetical calculations using only the human brain, with no help from any supplies or devices such as a calculator. People may use mental calculation when computing tools are not available, when it is faster than other means of calculation, or even in a competitive context. Mental calculation often involves the use of specific techniques devised for specific types of problems. People with unusually high ability to perform mental calculations are called mental calculators or lightning calculators.
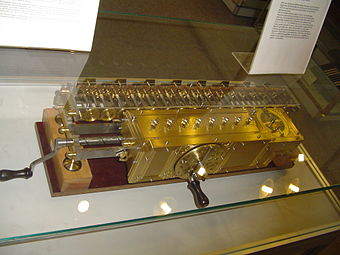
The arithmometer was the first digital mechanical calculator strong enough and reliable enough to be used daily in an office environment. This calculator could add and subtract two numbers directly and could perform long multiplications and divisions effectively by using a movable accumulator for the result.
Elementary arithmetic is a branch of mathematics involving addition, subtraction, multiplication, and division. Due to its low level of abstraction, broad range of application, and position as the foundation of all mathematics, elementary arithmetic is generally the first branch of mathematics taught in schools.
Pascal's calculator is a mechanical calculator invented by Blaise Pascal in 1642. Pascal was led to develop a calculator by the laborious arithmetical calculations required by his father's work as the supervisor of taxes in Rouen. He designed the machine to add and subtract two numbers directly and to perform multiplication and division through repeated addition or subtraction.
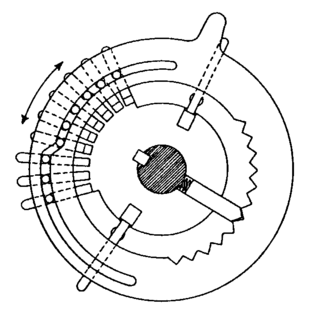
A pinwheel calculator is a class of mechanical calculator described as early as 1685, and popular in the 19th and 20th century, calculating via wheels whose number of teeth were adjustable. These wheels, also called pinwheels, could be set by using a side lever which could expose anywhere from 0 to 9 teeth, and therefore when coupled to a counter they could, at each rotation, add a number from 0 to 9 to the result. By linking these wheels with carry mechanisms a new kind of calculator engine was invented. Turn the wheels one way and one performs an addition, the other way a subtraction.
In elementary arithmetic, a standard algorithm or method is a specific method of computation which is conventionally taught for solving particular mathematical problems. These methods vary somewhat by nation and time, but generally include exchanging, regrouping, long division, and long multiplication using a standard notation, and standard formulas for average, area, and volume. Similar methods also exist for procedures such as square root and even more sophisticated functions, but have fallen out of the general mathematics curriculum in favor of calculators. As to standard algorithms in elementary mathematics, Fischer et al. (2019) state that advanced students use standard algorithms more effectively than peers who use these algorithms unreasoningly. That said, standard algorithms, such as addition, subtraction, as well as those mentioned above, represent central components of elementary math.
Kochanski multiplication is an algorithm that allows modular arithmetic to be performed efficiently when the modulus is large. This has particular application in number theory and in cryptography: for example, in the RSA cryptosystem and Diffie–Hellman key exchange.
A Leibniz wheel or stepped drum is a cylinder with a set of teeth of incremental lengths which, when coupled to a counting wheel, can be used in the calculating engine of a class of mechanical calculators. Invented by Leibniz in 1673, it was used for three centuries until the advent of the electronic calculator in the mid-1970s.
The Millionaire was the first commercially successful mechanical calculator that could perform a direct multiplication. It was in production from 1893 to 1935 with a total of about five thousand machines manufactured.
The Arithmaurel was a mechanical calculator that had a very intuitive user interface, especially for multiplying and dividing numbers because the result was displayed as soon as the operands were entered. It was first patented in France by Timoleon Maurel, in 1842. It received a gold medal at the French national show in Paris in 1849. Its complexity and the fragility of its design prevented it from being manufactured.
