The Symmetries of Things

Last updated

The Symmetries of Things is a book on mathematical symmetry and the symmetries of geometric objects, aimed at audiences of multiple levels. It was written over the course of many years by John Horton Conway, Heidi Burgiel, and Chaim Goodman-Strauss, [1] and published in 2008 by A K Peters. Its critical reception was mixed, with some reviewers praising it for its accessible and thorough approach to its material and for its many inspiring illustrations, [2] [3] [4] [5] and others complaining about its inconsistent level of difficulty, [6] overuse of neologisms, failure to adequately cite prior work, and technical errors. [7]

Contents

Topics

The Symmetries of Things has three major sections, subdivided into 26 chapters. [8] The first of the sections discusses the symmetries of geometric objects. It includes both the symmetries of finite objects in two and three dimensions, and two-dimensional infinite structures such as frieze patterns and tessellations, [2] and develops a new notation for these symmetries based on work of Alexander Murray MacBeath that, as proven by the authors using a simplified form of the Riemann–Hurwitz formula, covers all possibilities. [9] Other topics include Euler's polyhedral formula and the classification of two-dimensional surfaces. [8] It is heavily illustrated with both artworks and objects depicting these symmetries, such as the art of M. C. Escher [2] and Bathsheba Grossman, [3] [4] as well as new illustrations created by the authors using custom software. [2]

The second section of the book considers symmetries more abstractly and combinatorially, considering both the color-preserving symmetries of colored objects, the symmetries of topological spaces described in terms of orbifolds, and abstract forms of symmetry described by group theory and presentations of groups. This section culminates with a classification of all of the finite groups with up to 2009 elements. [2]

The third section of the book provides a classification of the three-dimensional space groups [2] and examples of honeycombs such as the Weaire–Phelan structure. [3] It also considers the symmetries of less familiar geometries: higher dimensional spaces, non-Euclidean spaces, [2] and three-dimensional flat manifolds. [9] Hyperbolic groups are used to provide a new explanation of the problem of hearing the shape of a drum. [8] [9] It includes the first published classification of four-dimensional convex uniform polytopes announced by Conway and Richard K. Guy in 1965, and a discussion of William Thurston's geometrization conjecture, proved by Grigori Perelman shortly before the publication of the book, according to which all three-dimensional manifolds can be realized as symmetric spaces. [2] One omission lamented by Jaron Lanier is the set of regular projective polytopes such as the 11-cell. [4]

Audience and reception

Reviewer Darren Glass writes that different parts of the book are aimed at different audiences, resulting in "a wonderful book which can be appreciated on many levels" [2] and providing an unusual level of depth for a popular mathematics book. [5] Its first section, on symmetries of low-dimensional Euclidean spaces, is suitable for a general audience. The second part involves some understanding of group theory, as would be expected of undergraduate mathematics students, and some additional familiarity with abstract algebra towards its end. And the third part, more technical, is primarily aimed at researchers in these topics, [2] although much still remains accessible at the undergraduate level. [9] It also has exercises making it useful as a textbook, and its heavy use of color illustration would make it suitable as a coffee table book. [2] However, reviewer Robert Moyer finds fault with its choice to include material at significantly different levels of difficulty, writing that for most of its audience, too much of the book will be unreadable. [6]

Much of the material in the book is either new, or previously known only through technical publications aimed at specialists, [1] [6] [8] and much of the previously-known material that it presents is described in new notation and nomenclature. [1] [8] Although there are many other books on symmetry, [2] reviewer N. G. Macleod writes that this one "may well become the definitive guide in this area for many years". [3] Jaron Lanier calls it "a plaything, an inexhaustible exercise in brain expansion for the reader, a work of art and a bold statement of what the culture of math can be like", and "a masterpiece". [4]

Despite these positive reviews, Branko Grünbaum, himself an authority on geometric symmetry, is much less enthusiastic, writing that the book has "some serious shortcomings". These include the unnecessary use of "cute" neologisms for concepts that already have well-established terminology, an inadequate treatment of MacBeath's and Andreas Dress's contributions to the book's notation, sloppy reasoning in some arguments, inaccurate claims of novelty and failure to credit previous work in the classification of colored plane patterns, missing cases in this classification, likely errors in other of the more technical parts, poor copyediting, and a lack of clear definitions that ends up leaving out such central notions as the symmetries of a circle without providing any explanation of why they were omitted. [7]

Related Research Articles

<span class="mw-page-title-main">Cube</span> Solid object with six equal square faces

In geometry, a cube is a three-dimensional solid object bounded by six square faces. It has twelve edges and eight vertices. It can be represented as a rectangular cuboid with six square faces, or a parallelepiped with equal edges. It is an example of many type of solids: Platonic solid, regular polyhedron, parallelohedron, zonohedron, and plesiohedron. The dual polyhedron of a cube is the regular octahedron.

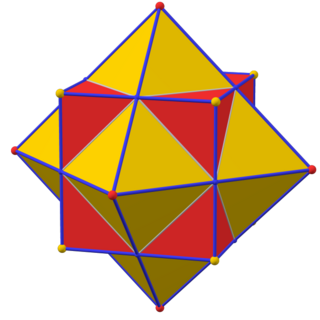
<span class="mw-page-title-main">Dual polyhedron</span> Polyhedron associated with another by swapping vertices for faces

In geometry, every polyhedron is associated with a second dual structure, where the vertices of one correspond to the faces of the other, and the edges between pairs of vertices of one correspond to the edges between pairs of faces of the other. Such dual figures remain combinatorial or abstract polyhedra, but not all can also be constructed as geometric polyhedra. Starting with any given polyhedron, the dual of its dual is the original polyhedron.

<span class="mw-page-title-main">Polyhedron</span> 3D shape with flat faces, straight edges and sharp corners

In geometry, a polyhedron is a three-dimensional shape with flat polygonal faces, straight edges and sharp corners or vertices.

In elementary geometry, a polytope is a geometric object with flat sides (faces). Polytopes are the generalization of three-dimensional polyhedra to any number of dimensions. Polytopes may exist in any general number of dimensions n as an n-dimensional polytope or n-polytope. For example, a two-dimensional polygon is a 2-polytope and a three-dimensional polyhedron is a 3-polytope. In this context, "flat sides" means that the sides of a (k + 1)-polytope consist of k-polytopes that may have (k – 1)-polytopes in common.

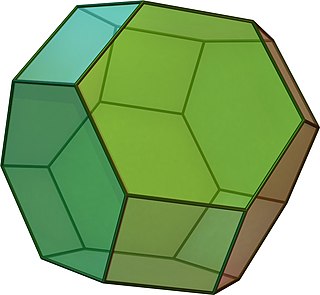
<span class="mw-page-title-main">Truncated octahedron</span> Archimedean solid

In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces, 36 edges, and 24 vertices. Since each of its faces has point symmetry the truncated octahedron is a 6-zonohedron. It is also the Goldberg polyhedron GIV(1,1), containing square and hexagonal faces. Like the cube, it can tessellate 3-dimensional space, as a permutohedron.

<span class="mw-page-title-main">Star polygon</span> Regular non-convex polygon

In geometry, a star polygon is a type of non-convex polygon. Regular star polygons have been studied in depth; while star polygons in general appear not to have been formally defined, certain notable ones can arise through truncation operations on regular simple or star polygons.

<span class="mw-page-title-main">Tessellation</span> Tiling of a plane in mathematics

A tessellation or tiling is the covering of a surface, often a plane, using one or more geometric shapes, called tiles, with no overlaps and no gaps. In mathematics, tessellation can be generalized to higher dimensions and a variety of geometries.

<span class="mw-page-title-main">Regular polytope</span> Polytope with highest degree of symmetry

In mathematics, a regular polytope is a polytope whose symmetry group acts transitively on its flags, thus giving it the highest degree of symmetry. In particular, all its elements or j-faces — cells, faces and so on — are also transitive on the symmetries of the polytope, and are themselves regular polytopes of dimension j≤ n.

In geometry, a polytope or a tiling is isogonal or vertex-transitive if all its vertices are equivalent under the symmetries of the figure. This implies that each vertex is surrounded by the same kinds of face in the same or reverse order, and with the same angles between corresponding faces.

<span class="mw-page-title-main">11-cell</span> Abstract regular 4-polytope

In mathematics, the 11-cell is a self-dual abstract regular 4-polytope. Its 11 cells are hemi-icosahedral. It has 11 vertices, 55 edges and 55 faces. It has Schläfli type {3,5,3}, with 3 hemi-icosahedra around each edge.

<span class="mw-page-title-main">Point group</span> Group of geometric symmetries with at least one fixed point

In geometry, a point group is a mathematical group of symmetry operations (isometries in a Euclidean space) that have a fixed point in common. The coordinate origin of the Euclidean space is conventionally taken to be a fixed point, and every point group in dimension d is then a subgroup of the orthogonal group O(d). Point groups are used to describe the symmetries of geometric figures and physical objects such as molecules.

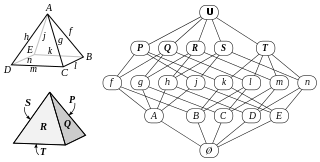
<span class="mw-page-title-main">Abstract polytope</span> Poset representing certain properties of a polytope

In mathematics, an abstract polytope is an algebraic partially ordered set which captures the dyadic property of a traditional polytope without specifying purely geometric properties such as points and lines.

<span class="mw-page-title-main">Quarter cubic honeycomb</span>

The quarter cubic honeycomb, quarter cubic cellulation or bitruncated alternated cubic honeycomb is a space-filling tessellation in Euclidean 3-space. It is composed of tetrahedra and truncated tetrahedra in a ratio of 1:1. It is called "quarter-cubic" because its symmetry unit – the minimal block from which the pattern is developed by reflections – is four times that of the cubic honeycomb.

<i>Regular Polytopes</i> (book) Geometry book

Regular Polytopes is a geometry book on regular polytopes written by Harold Scott MacDonald Coxeter. It was originally published by Methuen in 1947 and by Pitman Publishing in 1948, with a second edition published by Macmillan in 1963 and a third edition by Dover Publications in 1973. The Basic Library List Committee of the Mathematical Association of America has recommended that it be included in undergraduate mathematics libraries.

<span class="mw-page-title-main">Architectonic and catoptric tessellation</span> Uniform Euclidean 3D tessellations and their duals

In geometry, John Horton Conway defines architectonic and catoptric tessellations as the uniform tessellations of Euclidean 3-space with prime space groups and their duals, as three-dimensional analogue of the Platonic, Archimedean, and Catalan tiling of the plane. The singular vertex figure of an architectonic tessellation is the dual of the cell of the corresponding catoptric tessellation, and vice versa. The cubille is the only Platonic (regular) tessellation of 3-space, and is self-dual. There are other uniform honeycombs constructed as gyrations or prismatic stacks which are excluded from these categories.

<span class="mw-page-title-main">Regular skew apeirohedron</span> Infinite regular skew polyhedron

In geometry, a regular skew apeirohedron is an infinite regular skew polyhedron. They have either skew regular faces or skew regular vertex figures.

Convex Polytopes is a graduate-level mathematics textbook about convex polytopes, higher-dimensional generalizations of three-dimensional convex polyhedra. It was written by Branko Grünbaum, with contributions from Victor Klee, Micha Perles, and G. C. Shephard, and published in 1967 by John Wiley & Sons. It went out of print in 1970. A second edition, prepared with the assistance of Volker Kaibel, Victor Klee, and Günter M. Ziegler, was published by Springer-Verlag in 2003, as volume 221 of their book series Graduate Texts in Mathematics.

Mathematical Models is a book on the construction of physical models of mathematical objects for educational purposes. It was written by Martyn Cundy and A. P. Rollett, and published by the Clarendon Press in 1951, with a second edition in 1961. Tarquin Publications published a third edition in 1981.

Regular Figures is a book on polyhedra and symmetric patterns, by Hungarian geometer László Fejes Tóth. It was published in 1964 by Pergamon in London and Macmillan in New York.

References

  1. 1 2 3 Wilson, Phil (December 2008), "Review of The Symmetries of Things" (PDF), Plus Magazine, Millennium Mathematics Project, University of Cambridge
  2. 1 2 3 4 5 6 7 8 9 10 11 12 Glass, Darren (July 2008), "Review of The Symmetries of Things", MAA Reviews
  3. 1 2 3 4 Macleod, N. G. (March 2010), "Review of The Symmetries of Things", Mathematics in School, 39 (2): 43–44, JSTOR   20696997
  4. 1 2 3 4 Lanier, Jaron (January–February 2009), "From planar patterns to polytopes (review of The Symmetries of Things)", American Scientist , 97 (1): 73–75, JSTOR   27859276
  5. 1 2 Eaton, Charles (March 2012), The Mathematical Gazette , 96 (535): 188–190, doi:10.1017/S002555720000437X, JSTOR   23249557 {{citation}}: CS1 maint: untitled periodical (link)
  6. 1 2 3 Moyer, Robert (May 2009), "Review of The Symmetries of Things", The Mathematics Teacher , 102 (9): 716–717, JSTOR   20876494
  7. 1 2 Grünbaum, Branko (June–July 2009), "Review of The Symmetries of Things", The American Mathematical Monthly , 116 (6): 555–562, doi:10.4169/193009709X470470, JSTOR   40391162
  8. 1 2 3 4 5 Chirteş, Florentina, "Review of The Symmetries of Things", zbMATH, Zbl   1173.00001
  9. 1 2 3 4 Conder, Marston (2009), "Review of The Symmetries of Things", MathSciNet, MR   2410150
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.