Related Research Articles
Fractional calculus is a branch of mathematical analysis that studies the several different possibilities of defining real number powers or complex number powers of the differentiation operator
In fractional calculus, an area of mathematical analysis, the differintegral is a combined differentiation/integration operator. Applied to a function ƒ, the q-differintegral of f, here denoted by
In mathematics, time-scale calculus is a unification of the theory of difference equations with that of differential equations, unifying integral and differential calculus with the calculus of finite differences, offering a formalism for studying hybrid systems. It has applications in any field that requires simultaneous modelling of discrete and continuous data. It gives a new definition of a derivative such that if one differentiates a function defined on the real numbers then the definition is equivalent to standard differentiation, but if one uses a function defined on the integers then it is equivalent to the forward difference operator.
Functional integration is a collection of results in mathematics and physics where the domain of an integral is no longer a region of space, but a space of functions. Functional integrals arise in probability, in the study of partial differential equations, and in the path integral approach to the quantum mechanics of particles and fields.
The Chern–Simons theory is a 3-dimensional topological quantum field theory of Schwarz type developed by Edward Witten. It was discovered first by mathematical physicist Albert Schwarz. It is named after mathematicians Shiing-Shen Chern and James Harris Simons, who introduced the Chern–Simons 3-form. In the Chern–Simons theory, the action is proportional to the integral of the Chern–Simons 3-form.
In general relativity, Regge calculus is a formalism for producing simplicial approximations of spacetimes that are solutions to the Einstein field equation. The calculus was introduced by the Italian theoretician Tullio Regge in 1961.
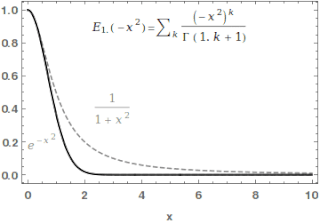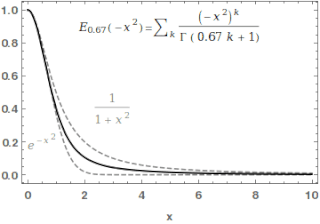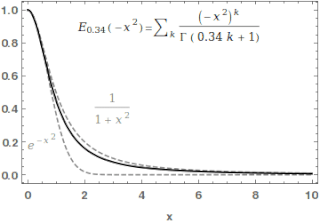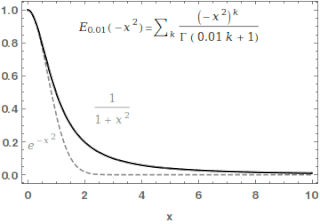
In mathematics, the Mittag-Leffler function is a special function, a complex function which depends on two complex parameters and . It may be defined by the following series when the real part of is strictly positive:
Finite element exterior calculus (FEEC) is a mathematical framework that formulates finite element methods using chain complexes. Its main application has been a comprehensive theory for finite element methods in computational electromagnetism, computational solid and fluid mechanics. FEEC was developed in the early 2000s by Douglas N. Arnold, Richard S. Falk and Ragnar Winther, among others. Finite element exterior calculus is sometimes called as an example of a compatible discretization technique, and bears similarities with discrete exterior calculus, although they are distinct theories.

Moshe Zakai was a Distinguished Professor at the Technion, Israel in electrical engineering, member of the Israel Academy of Sciences and Humanities and Rothschild Prize winner.

Jürg Martin Fröhlich is a Swiss mathematician and theoretical physicist. He is best known for introducing rigorous techniques for the analysis of statistical mechanics models, in particular continuous symmetry breaking, and for pioneering the study of topological phases of matter using low-energy effective field theories.
In applied mathematics and mathematical analysis, the fractal derivative or Hausdorff derivative is a non-Newtonian generalization of the derivative dealing with the measurement of fractals, defined in fractal geometry. Fractal derivatives were created for the study of anomalous diffusion, by which traditional approaches fail to factor in the fractal nature of the media. A fractal measure t is scaled according to tα. Such a derivative is local, in contrast to the similarly applied fractional derivative. Fractal calculus is formulated as a generalization of standard calculus.
In the fields of dynamical systems and control theory, a fractional-order system is a dynamical system that can be modeled by a fractional differential equation containing derivatives of non-integer order. Such systems are said to have fractional dynamics. Derivatives and integrals of fractional orders are used to describe objects that can be characterized by power-law nonlocality, power-law long-range dependence or fractal properties. Fractional-order systems are useful in studying the anomalous behavior of dynamical systems in physics, electrochemistry, biology, viscoelasticity and chaotic systems.
The Mittag-Leffler distributions are two families of probability distributions on the half-line . They are parametrized by a real or . Both are defined with the Mittag-Leffler function, named after Gösta Mittag-Leffler.

Alexander Nikolaevich Varchenko is a Soviet and Russian mathematician working in geometry, topology, combinatorics and mathematical physics.
In mathematics, Katugampola fractional operators are integral operators that generalize the Riemann–Liouville and the Hadamard fractional operators into a unique form. The Katugampola fractional integral generalizes both the Riemann–Liouville fractional integral and the Hadamard fractional integral into a single form and It is also closely related to the Erdelyi–Kober operator that generalizes the Riemann–Liouville fractional integral. Katugampola fractional derivative has been defined using the Katugampola fractional integral and as with any other fractional differential operator, it also extends the possibility of taking real number powers or complex number powers of the integral and differential operators.
Anry Nersessian is an Armenian mathematician, specializing in differential and integral equations, functional and numerical analysis. He is known for fractional derivatives introduced by the joint work with Mkhitar Djrbashian. Separately, M. Caputo considered the same modification of Riemann–Liouville integral, which is why the fractional derivative is known as Caputo, Djrbashian-Caputo or Caputo-Djrbashian derivative.

Daniele Mortari is Professor of Aerospace Engineering at Texas A&M University and Chief Scientist for Space for Texas A&M ASTRO Center. Mortari is known for inventing the Flower Constellations and the k-vector range searching technique and the Theory of Functional Connections.
Alexander "Sandy" Munro Davie is a Scottish mathematician and was the chess champion of Scotland in 1964, 1966, and 1969.
Virginia S. Kiryakova is a Bulgarian mathematician known for her work on the fractional calculus, on special functions in fractional calculus including the Mittag-Leffler functions, and on the history of calculus. She is a professor in the Institute of Mathematics and Informatics of the Bulgarian Academy of Sciences.
Prabhakar function is a certain special function in mathematics introduced by the Indian mathematician Tilak Raj Prabhakar in a paper published in 1971. The function is a three-parameter generalization of the well known two-parameter Mittag-Leffler function in mathematics. The function was originally introduced to solve certain classes of integral equations. Later the function was found to have applications in the theory of fractional calculus and also in certain areas of physics.
References
- ↑ T. R. Prabhakar (1971). "A singular integral equation with a generalized Mittag–Leffler function in the kernel". Yokohama Math. Journal. 19 (1): 7–15.
- ↑ Andrea Giusti, Ivano Colombaro, Roberto Garra (2020). "A practical guide to Prabhakar fractional calculus". Fractional Calculus and Applied Analysis. 23 (1): 9–54. arXiv: 2002.10978 . doi: 10.1515/fca-2020-0002 . Retrieved 26 December 2023.
{{cite journal}}: CS1 maint: multiple names: authors list (link) - ↑ Francesco Mainardi and Roberto Garrappa (July 2015). "On complete monotonicity of the Prabhakar function and non-Debye relaxation in dielectrics". Journal of Computational Physics. 293: 70–80. arXiv: 1610.01763 . Bibcode:2015JCoPh.293...70M. doi:10.1016/j.jcp.2014.08.006. S2CID 29142283 . Retrieved 26 December 2023.
- ↑ "Untimely demise of Dr. Tilak Raj Prabhakar". Ganita Bharati. 4 (1): i–ii. 1982.
- ↑ Roberto Garra and Roberto Garrappa (March 2018). "The Prabhakar or three parameter Mittag–Leffler function: theory and application". Communications in Nonlinear Science and Numerical Simulation. 56: 314–329. arXiv: 1708.07298 . Bibcode:2018CNSNS..56..314G. doi:10.1016/j.cnsns.2017.08.018.