Veblen–Young theorem

Last updated April 08, 2019

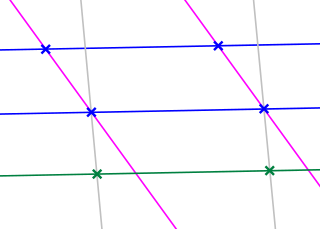
In mathematics, the Veblen–Young theorem, proved by OswaldVeblen and John Wesley Young ( 1908 , 1910 , 1917 ), states that a projective space of dimension at least 3 can be constructed as the projective space associated to a vector space over a division ring.

Oswald Veblen was an American mathematician, geometer and topologist, whose work found application in atomic physics and the theory of relativity. He proved the Jordan curve theorem in 1905; while this was long considered the first rigorous proof, many now also consider Camille Jordan's original proof rigorous.

John Wesley Young was an American mathematician who, with Oswald Veblen, introduced the axioms of projective geometry, coauthored a 2-volume work on them, and proved the Veblen–Young theorem.

Projective space space of 1-dimensional linear subspaces (lines passing through the origin) in a vector space

In mathematics, a projective space can be thought of as the set of lines through the origin of a vector space V. The cases when V = R² and V = R³ are the real projective line and the real projective plane, respectively, where R denotes the field of real numbers, R² denotes ordered pairs of real numbers, and R³ denotes ordered triplets of real numbers.

Non-Desarguesian planes give examples of 2-dimensional projective spaces that do not arise from vector spaces over division rings, showing that the restriction to dimension at least 3 is necessary.

In mathematics, a non-Desarguesian plane, named after Girard Desargues, is a projective plane that does not satisfy Desargues' theorem, or in other words a plane that is not a Desarguesian plane. The theorem of Desargues is valid in all projective spaces of dimension not 2, that is, all the classical projective geometries over a field, but David Hilbert found that some projective planes do not satisfy it. Understanding of these examples is not complete, in the current state of knowledge.

Jacques Tits generalized the Veblen–Young theorem to Tits buildings, showing that those of rank at least 3 arise from algebraic groups.

Jacques Tits is a Belgium-born French mathematician who works on group theory and incidence geometry. He introduced Tits buildings, the Tits alternative, the Tits group, and the Tits metric.

In algebraic geometry, an algebraic group is a group that is an algebraic variety, such that the multiplication and inversion operations are given by regular maps on the variety.

Johnvon Neumann ( 1998 ) generalized the Veblen–Young theorem to continuous geometry, showing that a complemented modular lattice of order at least 4 is isomorphic to the principal right ideals of a von Neumann regular ring.

John von Neumann mathematician and physicist

John von Neumann was a Hungarian-American mathematician, physicist, computer scientist, and polymath. Von Neumann was generally regarded as the foremost mathematician of his time and said to be "the last representative of the great mathematicians"; a genius who was comfortable integrating both pure and applied sciences.

In mathematics, continuous geometry is an analogue of complex projective geometry introduced by von Neumann, where instead of the dimension of a subspace being in a discrete set 0, 1, ..., n, it can be an element of the unit interval [0,1]. Von Neumann was motivated by his discovery of von Neumann algebras with a dimension function taking a continuous range of dimensions, and the first example of a continuous geometry other than projective space was the projections of the hyperfinite type II factor.

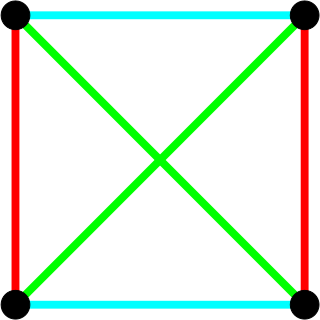
In the mathematical discipline of order theory, a complemented lattice is a bounded lattice, in which every element a has a complement, i.e. an element b satisfying a ∨ b = 1 and a ∧ b = 0. Complements need not be unique.

Statement

A projective spaceS can be defined abstractly as a set P (the set of points), together with a set L of subsets of P (the set of lines), satisfying these axioms :

Each two distinct points p and q are in exactly one line.
Veblen's axiom: If a, b, c, d are distinct points and the lines through ab and cd meet, then so do the lines through ac and bd.
Any line has at least 3 points on it.

The Veblen–Young theorem states that if the dimension of a projective space is at least 3 (meaning that there are two non-intersecting lines) then the projective space is isomorphic with the projective space of lines in a vector space over some division ring K.

In abstract algebra, a division ring, also called a skew field, is a ring in which division is possible. Specifically, it is a nonzero ring in which every nonzero element $a$ has a multiplicative inverse, i.e., an element $x$ with $a \cdot x = x \cdot a = 1.$ Stated differently, a ring is a division ring if and only if the group of units equals the set of all nonzero elements. A division ring is a type of noncommutative ring under the looser definition where noncommutative ring refers to rings which are not necessarily commutative.

Related Research Articles

Projective plane Geometric concept of a 2D space with a "point at infinity" adjoined

In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines that do not intersect. A projective plane can be thought of as an ordinary plane equipped with additional "points at infinity" where parallel lines intersect. Thus any two distinct lines in a projective plane intersect in one and only one point.

Projective geometry is a topic in mathematics. It is the study of geometric properties that are invariant with respect to projective transformations. This means that, compared to elementary geometry, projective geometry has a different setting, projective space, and a selective set of basic geometric concepts. The basic intuitions are that projective space has more points than Euclidean space, for a given dimension, and that geometric transformations are permitted that transform the extra points to Euclidean points, and vice versa.

In mathematics, affine geometry is what remains of Euclidean geometry when not using the metric notions of distance and angle.

A finite geometry is any geometric system that has only a finite number of points. The familiar Euclidean geometry is not finite, because a Euclidean line contains infinitely many points. A geometry based on the graphics displayed on a computer screen, where the pixels are considered to be the points, would be a finite geometry. While there are many systems that could be called finite geometries, attention is mostly paid to the finite projective and affine spaces because of their regularity and simplicity. Other significant types of finite geometry are finite Möbius or inversive planes and Laguerre planes, which are examples of a general type called Benz planes, and their higher-dimensional analogs such as higher finite inversive geometries.

In geometry, a striking feature of projective planes is the symmetry of the roles played by points and lines in the definitions and theorems, and (plane) duality is the formalization of this concept. There are two approaches to the subject of duality, one through language and the other a more functional approach through special mappings. These are completely equivalent and either treatment has as its starting point the axiomatic version of the geometries under consideration. In the functional approach there is a map between related geometries that is called a duality. Such a map can be constructed in many ways. The concept of plane duality readily extends to space duality and beyond that to duality in any finite-dimensional projective geometry.

In mathematics, a duality, generally speaking, translates concepts, theorems or mathematical structures into other concepts, theorems or structures, in a one-to-one fashion, often by means of an involution operation: if the dual of A is B, then the dual of B is A. Such involutions sometimes have fixed points, so that the dual of A is A itself. For example, Desargues' theorem is self-dual in this sense under the standard duality in projective geometry.

In geometry, an affine plane is a system of points and lines that satisfy the following axioms:

In mathematics, incidence geometry is the study of incidence structures. A geometric structure such as the Euclidean plane is a complicated object that involves concepts such as length, angles, continuity, betweenness, and incidence. An incidence structure is what is obtained when all other concepts are removed and all that remains is the data about which points lie on which lines. Even with this severe limitation, theorems can be proved and interesting facts emerge concerning this structure. Such fundamental results remain valid when additional concepts are added to form a richer geometry. It sometimes happens that authors blur the distinction between a study and the objects of that study, so it is not surprising to find that some authors refer to incidence structures as incidence geometries.

Karl Georg Christian von Staudt was a German mathematician who used synthetic geometry to provide a foundation for arithmetic.

In mathematics, the lines of a 3-dimensional projective space, S, can be viewed as points of a 5-dimensional projective space, T. In that 5-space, the points that represent each line in S lie on a hyperbolic quadric, Q known as the Klein quadric.

In projective geometry, a homography is an isomorphism of projective spaces, induced by an isomorphism of the vector spaces from which the projective spaces derive. It is a bijection that maps lines to lines, and thus a collineation. In general, some collineations are not homographies, but the fundamental theorem of projective geometry asserts that is not so in the case of real projective spaces of dimension at least two. Synonyms include projectivity, projective transformation, and projective collineation.

In mathematics, in the field of geometry, a polar space of rank n, or projective indexn − 1, consists of a set P, conventionally called the set of points, together with certain subsets of P, called subspaces, that satisfy these axioms:

In projective geometry, a collineation is a one-to-one and onto map from one projective space to another, or from a projective space to itself, such that the images of collinear points are themselves collinear. A collineation is thus an isomorphism between projective spaces, or an automorphism from a projective space to itself. Some authors restrict the definition of collineation to the case where it is an automorphism. The set of all collineations of a space to itself form a group, called the collineation group.

In mathematics, a space is a set with some added structure.

In geometry, an affine plane is a two-dimensional affine space.

References

Cameron, Peter J. (1992), Projective and polar spaces, QMW Maths Notes, 13, London: Queen Mary and Westfield College School of Mathematical Sciences, ISBN 978-0-902480-12-4, MR 1153019
Veblen, Oswald; Young, John Wesley (1908), "A Set of Assumptions for Projective Geometry", American Journal of Mathematics , 30 (4): 347–380, doi:10.2307/2369956, ISSN 0002-9327, MR 1506049
Veblen, Oswald; Young, John Wesley (1910), Projective geometry Volume I, Ginn and Co., Boston, ISBN 978-1-4181-8285-4, MR 0179666
Veblen, Oswald; Young, John Wesley (1917), Projective geometry Volume II, Ginn and Co., Boston, ISBN 978-1-60386-062-8, MR 0179667
von Neumann, John (1998) [1960], Continuous geometry, Princeton Landmarks in Mathematics, Princeton University Press, ISBN 978-0-691-05893-1, MR 0120174

International Standard Book Number Unique numeric book identifier

The International Standard Book Number (ISBN) is a numeric commercial book identifier which is intended to be unique. Publishers purchase ISBNs from an affiliate of the International ISBN Agency.

Mathematical Reviews is a journal published by the American Mathematical Society (AMS) that contains brief synopses, and in some cases evaluations, of many articles in mathematics, statistics, and theoretical computer science. The AMS also publishes an associated online bibliographic database called MathSciNet which contains an electronic version of Mathematical Reviews and additionally contains citation information for over 3.5 million items as of 2018.

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.