Circular mil

circular mil
circular mil
General information
Unit of	Area
Symbol	cmil
Derivation	1 cmil = ⁠π/4⁠(0.001 in)2
Conversions
1 cmil in ...	... is equal to ...
SI units	506.7075 μm2
FPS units	7.853982×10−7 in2

Last updated October 28, 2024

A circular mil is a unit of area, equal to the area of a circle with a diameter of one mil (one thousandth of an inch or 0.0254 mm). It is equal to $π$ /4 square mils or approximately 5.067×10⁻⁴ mm². It is a unit intended for referring to the area of a wire with a circular cross section. As the definition of the unit contains $π$ , it is easy to calculate area values in circular mils when the diameter in mils is known.

In Canada and the United States, the Canadian Electrical Code (CEC) and the National Electrical Code (NEC), respectively, use the circular mil to define wire sizes larger than 0000 AWG. In many NEC publications and uses, large wires may be expressed in thousands of circular mils, which is abbreviated in two different ways: kcmil^[1] or MCM.^[2] For example, one common wire size used in the NEC has a conductor diameter of 0.5 inches, or 500 mils, and thus a cross-section of $500^{2}=250{,}000$ circular mils, written as 250 kcmil or 250 MCM, which is the first size larger than 0000 AWG used within the NEC.

1000 circular mil equals approximately 0.5067 mm², so for many purposes, a ratio of 2 MCM ≈ 1 mm² can be used with negligible (1.3%) error.

Equivalence to other units of area

As a unit of area, the circular mil can be converted to other units such as square inches or square millimetres.

1 circular mil is approximately equal to:

0.7854 square mils (1 square mil is about 1.273 circular mils)
7.854 × 10⁻⁷ square inches (1 square inch is about 1.273 million circular mils)
5.067 × 10⁻¹⁰ square metres
5.067 × 10⁻⁴ square millimetres
506.7 μm²

1000 circular mils = 1 MCM or 1 kcmil, and is (approximately) equal to:

0.5067 mm², so 2 kcmil ≈ 1 mm² (a 1.3% error)

Therefore, for practical purposes such as wire choice, 2 kcmil ≈ 1 mm² is a reasonable rule of thumb for many applications.

Square mils

In square mils, the area of a circle with a diameter of 1 mil is:

$A=\pi r^{2}=\pi \left({\frac {d}{2}}\right)^{2}={\frac {\pi d^{2}}{4}}={\rm {{\frac {\pi \times (1~mil)^{2}}{4}}={\frac {\pi }{4}}~mil^{2}\approx 0.7854~mil^{2}.}}$

By definition, this area is also equal to 1 circular mil, so

${\rm {1~cmil={\frac {\pi }{4}}~mil^{2}.}}$

The conversion factor from square mils to circular mils is therefore 4/ $π$ cmil per square mil:

${\rm {{\frac {4}{\pi }}{\frac {cmil}{mil^{2}}}.}}$

The formula for the area of an arbitrary circle in circular mils can be derived by applying this conversion factor to the standard formula for the area of a circle (which gives its result in square mils).

${\begin{aligned}\{A\}_{{\textrm {mil}}^{2}}&=\pi r^{2}=\pi \left({\frac {d}{2}}\right)^{2}={\frac {\pi d^{2}}{4}}&&({\text{Area in square mils}})\\[2ex]\{A\}_{\textrm {cmil}}&=\{A\}_{{\textrm {mil}}^{2}}\times {\frac {4}{\pi }}&&({\text{Convert to cmil}})\\[2ex]\{A\}_{\textrm {cmil}}&={\frac {\pi d^{2}}{4}}\times {\frac {4}{\pi }}&&({\text{Substitute area in square mils with its definition}})\\[2ex]\{A\}_{\textrm {cmil}}&=d^{2}.\end{aligned}}$

Square inches

To equate circular mils with square inches rather than square mils, the definition of a mil in inches can be substituted:

{\begin{aligned}{\rm {1~cmil}}&={\rm {{\frac {\pi }{4}}~mil^{2}={\frac {\pi }{4}}~(0.001~in)^{2}}}\\[2ex]&={\rm {{\frac {\pi }{4{,}000{,}000}}~in^{2}\approx 7.854\times 10^{-7}~in^{2}}}\end{aligned}}

Square millimetres

Likewise, since 1 inch is defined as exactly 25.4 mm, 1 mil is equal to exactly 0.0254 mm, so a similar conversion is possible from circular mils to square millimetres:

{\begin{aligned}{\rm {1~cmil}}&={\rm {{\frac {\pi }{4}}~mil^{2}={\frac {\pi }{4}}~(0.0254~mm)^{2}={\frac {\pi \times 0.000\,645\,16}{4}}~mm^{2}}}\\[2ex]&={\rm {1.6129\pi \times 10^{-4}~mm^{2}\approx 5.067\times 10^{-4}~mm^{2}}}\end{aligned}}

Example calculations

A 0000 AWG solid wire is defined to have a diameter of exactly 0.46 inches (11.68 mm). The cross-sectional area of this wire is:

Formula 1: circular mil

Note: 1 inch = 1000 mils

{\begin{aligned}d&={\rm {0.46~inches=460~mil}}\\A&=d^{2}~{\rm {cmil/mil^{2}=(460~mil)^{2}~cmil/mil^{2}=211{,}600~cmil.}}\end{aligned}}

(This is the same result as the AWG circular mil formula shown below for $n = -3$ )

Formula 2: square mil

{\begin{aligned}d&={\rm {0.46~inches=460~mils}}\\r&={d \over 2}={\rm {230~mils}}\\A&=\pi r^{2}={\rm {\pi \times (230~mil)^{2}=52{,}900\pi ~mil^{2}\approx 166{,}190.25~mil^{2}}}\end{aligned}}

Formula 3: square inch

{\begin{aligned}d&={\rm {0.46~inches}}\\r&={d \over 2}={\rm {0.23~inches}}\\A&=\pi r^{2}={\rm {\pi \times (0.23~in)^{2}=0.0529\pi \approx 0.16619~in^{2}}}\end{aligned}}

Calculating diameter from area

When large diameter wire sizes are specified in kcmil, such as the widely used 250 kcmil and 350 kcmil wires, the diameter of the wire can be calculated from the area without using $π$ :

We first convert from kcmil to circular mil

{\begin{aligned}A&={\rm {250~kcmil=250{,}000~{\text{cmil}}}}\\d&={\sqrt {A~\mathrm {mil^{2}/cmil} }}\\d&={\rm {{\sqrt {(250{,}000~cmil)~mil^{2}/cmil}}=500~mil=0.500~inches}}\end{aligned}}

Thus, this wire would have a diameter of a half inch or 12.7 mm.

Metric equivalent

Some tables give conversions to circular millimetres (cmm).^[3]^[4] The area in cmm is defined as the square of the wire diameter in mm. However, this unit is rarely used in practice. One of the few examples is in a patent for a bariatric weight loss device.^[5]

{\rm {1~cmm=\left({\frac {1000}{25.4}}\right)^{2}~cmil\approx 1{,}550~cmil}}

AWG circular mil formula

The formula to calculate the area in circular mil for any given AWG (American Wire Gauge) size is as follows. $A_{n}$ represents the area of number $n$ AWG.

A_{n}=\left(5\times 92^{(36-n)/39}\right)^{2}

For example, a number 12 gauge wire would use $n=12$ :

\left(5\times 92^{(36-12)/39}\right)^{2}=6530~{\textrm {cmil}}

Sizes with multiple zeros are successively larger than 0 AWG and can be denoted using "number of zeros/0"; for example "4/0" for 0000 AWG. For an $m$ /0 AWG wire, use

n=-(m-1)=1-m

in the above formula.

For example, 0000 AWG (4/0 AWG), would use $n=-3$ ; and the calculated result would be 211,600 circular mils.

Standard sizes

Standard sizes are from 250 to 400 in increments of 50 kcmil, 400 to 1000 in increments of 100 kcmil, and from 1000 to 2000 in increments of 250 kcmil.^[6]

The diameter in the table below is that of a solid rod with the given conductor area in circular mils. Stranded wire is larger in diameter to allow for gaps between the strands, depending on the number and size of strands.

Standard kcmil wire sizes
& solid copper equivalents
Area		Diameter		NEC copper wire ampacity with 60/75/90 °C insulation (A)^[7]
(kcmil, MCM)	(mm²)	(in)	(mm)
250	126.7	0.500	12.70	215	255	290
300	152.0	0.548	13.91	240	285	320
350	177.3	0.592	15.03	260	310	350
400	202.7	0.632	16.06	280	335	380
500	253.4	0.707	17.96	320	380	430
600	304.0	0.775	19.67	355	420	475
700	354.7	0.837	21.25	385	460	520
750	380.0	0.866	22.00	400	475	535
800	405.4	0.894	22.72	410	490	555
900	456.0	0.949	24.10	435	520	585
1000	506.7	1.000	25.40	455	545	615
1250	633.4	1.118	28.40	495	590	665
1500	760.1	1.225	31.11	520	625	705
1750	886.7	1.323	33.60	545	650	735
2000	1013.4	1.414	35.92	560	665	750

Note: For smaller wires, consult American wire gauge § Tables of AWG wire sizes.

Related Research Articles

Bessel functions, first defined by the mathematician Daniel Bernoulli and then generalized by Friedrich Bessel, are canonical solutions $y (x)$ of Bessel's differential equation $for an arbitrary complex number, which represents the order of the Bessel function. Although and produce the same differential equation, it is conventional to define different Bessel functions for these two values in such a way that the Bessel functions are mostly smooth functions of .$

The Stefan–Boltzmann law, also known as Stefan's law, describes the intensity of the thermal radiation emitted by matter in terms of that matter's temperature. It is named for Josef Stefan, who empirically derived the relationship, and Ludwig Boltzmann who derived the law theoretically.

<span class="mw-page-title-main">Fourier series</span> Decomposition of periodic functions into sums of simpler sinusoidal forms

A Fourier series is an expansion of a periodic function into a sum of trigonometric functions. The Fourier series is an example of a trigonometric series, but not all trigonometric series are Fourier series. By expressing a function as a sum of sines and cosines, many problems involving the function become easier to analyze because trigonometric functions are well understood. For example, Fourier series were first used by Joseph Fourier to find solutions to the heat equation. This application is possible because the derivatives of trigonometric functions fall into simple patterns. Fourier series cannot be used to approximate arbitrary functions, because most functions have infinitely many terms in their Fourier series, and the series do not always converge. Well-behaved functions, for example smooth functions, have Fourier series that converge to the original function. The coefficients of the Fourier series are determined by integrals of the function multiplied by trigonometric functions, described in Common forms of the Fourier series below.

In geometry, a solid angle is a measure of the amount of the field of view from some particular point that a given object covers. That is, it is a measure of how large the object appears to an observer looking from that point. The point from which the object is viewed is called the apex of the solid angle, and the object is said to subtend its solid angle at that point.

<span class="mw-page-title-main">Effusion</span> Process of a gas escaping through a small hole

In physics and chemistry, effusion is the process in which a gas escapes from a container through a hole of diameter considerably smaller than the mean free path of the molecules. Such a hole is often described as a pinhole and the escape of the gas is due to the pressure difference between the container and the exterior.

American Wire Gauge (AWG) is a logarithmic stepped standardized wire gauge system used since 1857, predominantly in North America, for the diameters of round, solid, nonferrous, electrically conducting wire. Dimensions of the wires are given in ASTM standard B 258. The cross-sectional area of each gauge is an important factor for determining its current-carrying capacity.

<span class="mw-page-title-main">Debye model</span> Method in physics

In thermodynamics and solid-state physics, the Debye model is a method developed by Peter Debye in 1912 to estimate phonon contribution to the specific heat in a solid. It treats the vibrations of the atomic lattice (heat) as phonons in a box in contrast to the Einstein photoelectron model, which treats the solid as many individual, non-interacting quantum harmonic oscillators. The Debye model correctly predicts the low-temperature dependence of the heat capacity of solids, which is proportional to $- the Debye T 3 law . Similarly to the Einstein photoelectron model, it recovers the Dulong-Petit law at high temperatures. Due to simplifying assumptions, its accuracy suffers at intermediate temperatures.$

The Basel problem is a problem in mathematical analysis with relevance to number theory, concerning an infinite sum of inverse squares. It was first posed by Pietro Mengoli in 1650 and solved by Leonhard Euler in 1734, and read on 5 December 1735 in The Saint Petersburg Academy of Sciences. Since the problem had withstood the attacks of the leading mathematicians of the day, Euler's solution brought him immediate fame when he was twenty-eight. Euler generalised the problem considerably, and his ideas were taken up more than a century later by Bernhard Riemann in his seminal 1859 paper "On the Number of Primes Less Than a Given Magnitude", in which he defined his zeta function and proved its basic properties. The problem is named after Basel, hometown of Euler as well as of the Bernoulli family who unsuccessfully attacked the problem.

In probability theory, the Landau distribution is a probability distribution named after Lev Landau. Because of the distribution's "fat" tail, the moments of the distribution, such as mean or variance, are undefined. The distribution is a particular case of stable distribution.

In mathematics, the discrete-time Fourier transform (DTFT) is a form of Fourier analysis that is applicable to a sequence of discrete values.

The Compton wavelength is a quantum mechanical property of a particle, defined as the wavelength of a photon whose energy is the same as the rest energy of that particle. It was introduced by Arthur Compton in 1923 in his explanation of the scattering of photons by electrons.

In orbital mechanics, mean motion is the angular speed required for a body to complete one orbit, assuming constant speed in a circular orbit which completes in the same time as the variable speed, elliptical orbit of the actual body. The concept applies equally well to a small body revolving about a large, massive primary body or to two relatively same-sized bodies revolving about a common center of mass. While nominally a mean, and theoretically so in the case of two-body motion, in practice the mean motion is not typically an average over time for the orbits of real bodies, which only approximate the two-body assumption. It is rather the instantaneous value which satisfies the above conditions as calculated from the current gravitational and geometric circumstances of the body's constantly-changing, perturbed orbit.

In geometry, the area enclosed by a circle of radius $r$ is $π r 2$ . Here the Greek letter $π$ represents the constant ratio of the circumference of any circle to its diameter, approximately equal to 3.14159.

Microstrip is a type of electrical transmission line which can be fabricated with any technology where a conductor is separated from a ground plane by a dielectric layer known as "substrate". Microstrip lines are used to convey microwave-frequency signals.

In mathematics, Machin-like formulas are a popular technique for computing $π$ to a large number of digits. They are generalizations of John Machin's formula from 1706:

For most numbered asteroids, almost nothing is known apart from a few physical parameters and orbital elements. Some physical characteristics can only be estimated. The physical data is determined by making certain standard assumptions.

A circular arc is the arc of a circle between a pair of distinct points. If the two points are not directly opposite each other, one of these arcs, the minor arc, subtends an angle at the center of the circle that is less than $π$ radians ; and the other arc, the major arc, subtends an angle greater than $π$ radians. The arc of a circle is defined as the part or segment of the circumference of a circle. A straight line that connects the two ends of the arc is known as a chord of a circle. If the length of an arc is exactly half of the circle, it is known as a semicircular arc.

<span class="mw-page-title-main">Sectional density</span> Gun ballistic calculation

Sectional density is the ratio of an object's mass to its cross sectional area with respect to a given axis. It conveys how well an object's mass is distributed to overcome resistance along that axis.

<span class="mw-page-title-main">Wrapped exponential distribution</span> Probability distribution

In probability theory and directional statistics, a wrapped exponential distribution is a wrapped probability distribution that results from the "wrapping" of the exponential distribution around the unit circle.

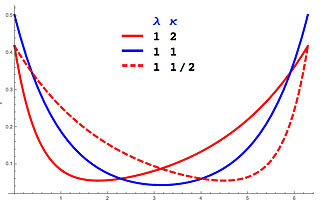
<span class="mw-page-title-main">Wrapped asymmetric Laplace distribution</span>

In probability theory and directional statistics, a wrapped asymmetric Laplace distribution is a wrapped probability distribution that results from the "wrapping" of the asymmetric Laplace distribution around the unit circle. For the symmetric case (asymmetry parameter κ = 1), the distribution becomes a wrapped Laplace distribution. The distribution of the ratio of two circular variates (Z) from two different wrapped exponential distributions will have a wrapped asymmetric Laplace distribution. These distributions find application in stochastic modelling of financial data.

References

↑ "Popular Acronyms" Archived 2011-09-03 at the Wayback Machine . NEMA
↑ "Energy Acronyms", California Energy Commission
↑ Charles Hoare, The A.B.C. of Slide Rule Practice, p. 52, London: Aston & Mander, 1872 OCLC 605063273
↑ Edwin James Houston, A Dictionary of Electrical Words, Terms and Phrases, p. 135, New York: W. J. Johnston, 1889 OCLC 1069614872
↑ Greg A. Lloyd, Bariatric Magnetic Apparatus and Method of Manufacturing Thereof, US patent US 8481076 , 9 July 2013.
↑ NFPA 70-2011 National Electrical Code 2011 Edition Archived 2008-10-15 at the Wayback Machine . Table 310.15(B)(17) page 70-155, Allowable Ampacities of Single-Insulated Conductors Rated Up to and Including 2000 Volts in Free Air, Based on Ambient Air Temperature of 30°C (86°F).
↑ NFPA 70 National Electrical Code 2008 Edition Archived 2008-10-15 at the Wayback Machine . Table 310.16 page 70-148, Allowable ampacities of insulated conductors rated 0 through 2000 volts, 60°C through 90°C, not more than three current-carrying conductors in raceway, cable, or earth (directly buried) based on ambient temperature of 30°C. Extracts from NFPA 70 do not represent the full position of NFPA and the original complete Code must be consulted. In particular, the maximum permissible overcurrent protection devices may set a lower limit.

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] "Popular Acronyms" Archived 2011-09-03 at the Wayback Machine . NEMA

[2] "Energy Acronyms", California Energy Commission

[3] Charles Hoare, The A.B.C. of Slide Rule Practice, p. 52, London: Aston & Mander, 1872 OCLC 605063273

[4] Edwin James Houston, A Dictionary of Electrical Words, Terms and Phrases, p. 135, New York: W. J. Johnston, 1889 OCLC 1069614872

[5] Greg A. Lloyd, Bariatric Magnetic Apparatus and Method of Manufacturing Thereof, US patent US 8481076 , 9 July 2013.

[6] NFPA 70-2011 National Electrical Code 2011 Edition Archived 2008-10-15 at the Wayback Machine . Table 310.15(B)(17) page 70-155, Allowable Ampacities of Single-Insulated Conductors Rated Up to and Including 2000 Volts in Free Air, Based on Ambient Air Temperature of 30°C (86°F).

[7] NFPA 70 National Electrical Code 2008 Edition Archived 2008-10-15 at the Wayback Machine . Table 310.16 page 70-148, Allowable ampacities of insulated conductors rated 0 through 2000 volts, 60°C through 90°C, not more than three current-carrying conductors in raceway, cable, or earth (directly buried) based on ambient temperature of 30°C. Extracts from NFPA 70 do not represent the full position of NFPA and the original complete Code must be consulted. In particular, the maximum permissible overcurrent protection devices may set a lower limit.

[1]

[2]

[3]

[4]

[5]

[6]

[7]