
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or plane area refers to the area of a shape or planar lamina, while surface area refers to the area of an open surface or the boundary of a three-dimensional object. Area can be understood as the amount of material with a given thickness that would be necessary to fashion a model of the shape, or the amount of paint necessary to cover the surface with a single coat. It is the two-dimensional analogue of the length of a curve or the volume of a solid.

In geometry, the circumference is the perimeter of a circle or ellipse. That is, the circumference would be the arc length of the circle, as if it were opened up and straightened out to a line segment. More generally, the perimeter is the curve length around any closed figure. Circumference may also refer to the circle itself, that is, the locus corresponding to the edge of a disk. The circumference of a sphere is the circumference, or length, of any one of its great circles.

In mathematics, Hausdorff dimension is a measure of roughness, or more specifically, fractal dimension, that was first introduced in 1918 by mathematician Felix Hausdorff. For instance, the Hausdorff dimension of a single point is zero, of a line segment is 1, of a square is 2, and of a cube is 3. That is, for sets of points that define a smooth shape or a shape that has a small number of corners—the shapes of traditional geometry and science—the Hausdorff dimension is an integer agreeing with the usual sense of dimension, also known as the topological dimension. However, formulas have also been developed that allow calculation of the dimension of other less simple objects, where, solely on the basis of their properties of scaling and self-similarity, one is led to the conclusion that particular objects—including fractals—have non-integer Hausdorff dimensions. Because of the significant technical advances made by Abram Samoilovitch Besicovitch allowing computation of dimensions for highly irregular or "rough" sets, this dimension is also commonly referred to as the Hausdorff–Besicovitch dimension.
A perimeter is a closed path that encompasses, surrounds, or outlines either a two dimensional shape or a one-dimensional length. The perimeter of a circle or an ellipse is called its circumference.

In geometry, the convex hull or convex envelope or convex closure of a shape is the smallest convex set that contains it. The convex hull may be defined either as the intersection of all convex sets containing a given subset of a Euclidean space, or equivalently as the set of all convex combinations of points in the subset. For a bounded subset of the plane, the convex hull may be visualized as the shape enclosed by a rubber band stretched around the subset.
In mathematics, the isoperimetric inequality is a geometric inequality involving the perimeter of a set and its volume. In -dimensional space the inequality lower bounds the surface area or perimeter of a set by its volume ,

In geometry, Barbier's theorem states that every curve of constant width has perimeter π times its width, regardless of its precise shape. This theorem was first published by Joseph-Émile Barbier in 1860.

In geometry, a convex polygon is a polygon that is the boundary of a convex set. This means that the line segment between two points of the polygon is contained in the union of the interior and the boundary of the polygon. In particular, it is a simple polygon. Equivalently, a polygon is convex if every line that does not contain any edge intersects the polygon in at most two points.

In geometry, the mean width is a measure of the "size" of a body; see Hadwiger's theorem for more about the available measures of bodies. In dimensions, one has to consider -dimensional hyperplanes perpendicular to a given direction in , where is the n-sphere . The "width" of a body in a given direction is the distance between the closest pair of such planes, such that the body is entirely in between the two hyper planes. The mean width is the average of this "width" over all in .
In mathematics, in the theory of several complex variables and complex manifolds, a Stein manifold is a complex submanifold of the vector space of n complex dimensions. They were introduced by and named after Karl Stein (1951). A Stein space is similar to a Stein manifold but is allowed to have singularities. Stein spaces are the analogues of affine varieties or affine schemes in algebraic geometry.
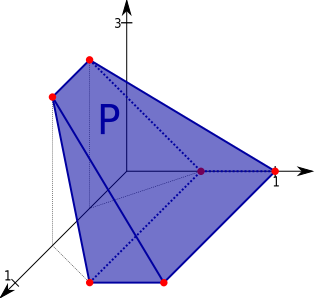
A convex polytope is a special case of a polytope, having the additional property that it is also a convex set contained in the -dimensional Euclidean space . Most texts use the term "polytope" for a bounded convex polytope, and the word "polyhedron" for the more general, possibly unbounded object. Others allow polytopes to be unbounded. The terms "bounded/unbounded convex polytope" will be used below whenever the boundedness is critical to the discussed issue. Yet other texts identify a convex polytope with its boundary.

Sphericity is a measure of how closely the shape of an object resembles that of a perfect sphere. For example, the sphericity of the balls inside a ball bearing determines the quality of the bearing, such as the load it can bear or the speed at which it can turn without failing. Sphericity is a specific example of a compactness measure of a shape. Defined by Wadell in 1935, the sphericity, , of a particle is the ratio of the surface area of a sphere with the same volume as the given particle to the surface area of the particle:

In the mathematical theory of functional analysis, the Krein–Milman theorem is a proposition about compact convex sets in locally convex topological vector spaces (TVSs).
In mathematics, the Crofton formula, named after Morgan Crofton (1826–1915), is a classic result of integral geometry relating the length of a curve to the expected number of times a "random" line intersects it.
Roundness is the measure of how closely the shape of an object approaches that of a mathematically perfect circle. Roundness applies in two dimensions, such as the cross sectional circles along a cylindrical object such as a shaft or a cylindrical roller for a bearing. In geometric dimensioning and tolerancing, control of a cylinder can also include its fidelity to the longitudinal axis, yielding cylindricity. The analogue of roundness in three dimensions is sphericity.
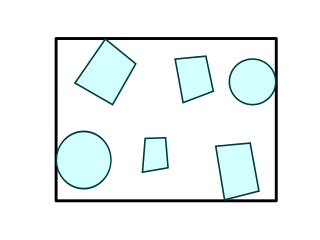
In geometry, the minimum or smallest bounding or enclosing box for a point set S in N dimensions is the box with the smallest measure within which all the points lie. When other kinds of measure are used, the minimum box is usually called accordingly, e.g., "minimum-perimeter bounding box".

An oloid is a three-dimensional curved geometric object that was discovered by Paul Schatz in 1929. It is the convex hull of a skeletal frame made by placing two linked congruent circles in perpendicular planes, so that the center of each circle lies on the edge of the other circle. The distance between the circle centers equals the radius of the circles. One third of each circle's perimeter lies inside the convex hull, so the same shape may be also formed as the convex hull of the two remaining circular arcs each spanning an angle of 4π/3.

In geometry, circle packing is the study of the arrangement of circles on a given surface such that no overlapping occurs and so that no circle can be enlarged without creating an overlap. The associated packing density, η, of an arrangement is the proportion of the surface covered by the circles. Generalisations can be made to higher dimensions – this is called sphere packing, which usually deals only with identical spheres.
Shape factors are dimensionless quantities used in image analysis and microscopy that numerically describe the shape of a particle, independent of its size. Shape factors are calculated from measured dimensions, such as diameter, chord lengths, area, perimeter, centroid, moments, etc. The dimensions of the particles are usually measured from two-dimensional cross-sections or projections, as in a microscope field, but shape factors also apply to three-dimensional objects. The particles could be the grains in a metallurgical or ceramic microstructure, or the microorganisms in a culture, for example. The dimensionless quantities often represent the degree of deviation from an ideal shape, such as a circle, sphere or equilateral polyhedron. Shape factors are often normalized, that is, the value ranges from zero to one. A shape factor equal to one usually represents an ideal case or maximum symmetry, such as a circle, sphere, square or cube.

In discrete geometry, an opaque set is a system of curves or other set in the plane that blocks all lines of sight across a polygon, circle, or other shape. Opaque sets have also been called barriers, beam detectors, opaque covers, or opaque forests. Opaque sets were introduced by Stefan Mazurkiewicz in 1916, and the problem of minimizing their total length was posed by Frederick Bagemihl in 1959.















