Related Research Articles

In mathematics and computer science, graph theory is the study of graphs, which are mathematical structures used to model pairwise relations between objects. A graph in this context is made up of vertices which are connected by edges. A distinction is made between undirected graphs, where edges link two vertices symmetrically, and directed graphs, where edges link two vertices asymmetrically. Graphs are one of the principal objects of study in discrete mathematics.

In graph theory, a tree is an undirected graph in which any two vertices are connected by exactly one path, or equivalently a connected acyclic undirected graph. A forest is an undirected graph in which any two vertices are connected by at most one path, or equivalently an acyclic undirected graph, or equivalently a disjoint union of trees.

In the mathematical field of graph theory, a bipartite graph is a graph whose vertices can be divided into two disjoint and independent sets and , that is, every edge connects a vertex in to one in . Vertex sets and are usually called the parts of the graph. Equivalently, a bipartite graph is a graph that does not contain any odd-length cycles.

Fan-Rong King Chung Graham, known professionally as Fan Chung, is a Taiwanese-born American mathematician who works mainly in the areas of spectral graph theory, extremal graph theory and random graphs, in particular in generalizing the Erdős–Rényi model for graphs with general degree distribution.

In discrete mathematics, and more specifically in graph theory, a vertex or node is the fundamental unit of which graphs are formed: an undirected graph consists of a set of vertices and a set of edges, while a directed graph consists of a set of vertices and a set of arcs. In a diagram of a graph, a vertex is usually represented by a circle with a label, and an edge is represented by a line or arrow extending from one vertex to another.

In graph theory, the degree of a vertex of a graph is the number of edges that are incident to the vertex; in a multigraph, a loop contributes 2 to a vertex's degree, for the two ends of the edge. The degree of a vertex is denoted or . The maximum degree of a graph is denoted by , and is the maximum of 's vertices' degrees. The minimum degree of a graph is denoted by , and is the minimum of 's vertices' degrees. In the multigraph shown on the right, the maximum degree is 5 and the minimum degree is 0.
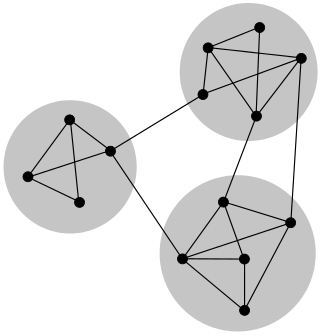
In mathematics and computer science, connectivity is one of the basic concepts of graph theory: it asks for the minimum number of elements that need to be removed to separate the remaining nodes into two or more isolated subgraphs. It is closely related to the theory of network flow problems. The connectivity of a graph is an important measure of its resilience as a network.

In mathematics, and more specifically in graph theory, a directed graph is a graph that is made up of a set of vertices connected by directed edges, often called arcs.
Ellis Lane Johnson is the Professor Emeritus and the Coca-Cola Chaired Professor in the H. Milton Stewart School of Industrial and Systems Engineering at Georgia Institute of Technology in Atlanta, Georgia.

Vincent Daniel Blondel is a Belgian professor of applied mathematics and former rector of the University of Louvain (UCLouvain) and a visiting professor at the Massachusetts Institute of Technology (MIT). Blondel's research lies in the area of mathematical control theory and theoretical computer science. He is mostly known for his contributions in computational complexity in control, multi-agent coordination and complex networks.
Daniela Kühn is a German mathematician and the Mason Professor in Mathematics at the University of Birmingham in Birmingham, England. She is known for her research in combinatorics, and particularly in extremal combinatorics and graph theory.

Bruce Alan ReedFRSC is a Canadian mathematician and computer scientist, a former Canada Research Chair in Graph Theory at McGill University. His research is primarily in graph theory. He is a distinguished research fellow of the Institute of Mathematics in the Academia Sinica, Taiwan, and an adjunct professor at the University of Victoria in Canada.
Wen-Ch'ing (Winnie) Li is a Taiwanese-American mathematician and a Distinguished Professor of Mathematics at Pennsylvania State University. She is a number theorist, with research focusing on the theory of automorphic forms and applications of number theory to coding theory and spectral graph theory. In particular, she has applied her research results in automorphic forms and number theory to construct efficient communication networks called Ramanujan graphs and Ramanujan complexes.
Arthur Marmaduke Hobbs was an American mathematician specializing in graph theory. He spent his teaching career at Texas A&M University.
Leslie Hogben is an American mathematician specializing in graph theory and linear algebra, and known for her mentorship of graduate students in mathematics. She is a professor emerita of mathematics at Iowa State University, where she held the Dio Lewis Holl Chair in Applied Mathematics 2012-2020; she was also professor of electrical and computer engineering at Iowa State, associate dean for graduate studies and faculty development of the College of Liberal Arts and Sciences at Iowa State (2019-2024), and associate director for diversity at the American Institute of Mathematics.
Christina Magdalena (Kieka) Mynhardt is a South African born Canadian mathematician known for her work on dominating sets in graph theory, including domination versions of the eight queens puzzle. She is a professor of mathematics and statistics at the University of Victoria in Canada.
Pósa's theorem, in graph theory, is a sufficient condition for the existence of a Hamiltonian cycle based on the degrees of the vertices in an undirected graph. It implies two other degree-based sufficient conditions, Dirac's theorem on Hamiltonian cycles and Ore's theorem. Unlike those conditions, it can be applied to graphs with a small number of low-degree vertices. It is named after Lajos Pósa, a protégé of Paul Erdős born in 1947, who discovered this theorem in 1962.
Mirka Miller was a Czech-Australian mathematician and computer scientist interested in graph theory and data security. She was a professor of electrical engineering and computer science at the University of Newcastle.
Debra Lynn Boutin is an American mathematician, the Samuel F. Pratt Professor of Mathematics at Hamilton College, where she chairs the mathematics department. Her research involves the symmetries of graphs and distinguishing colorings of graphs.
References
- 1 2 3 4 5 "Daniela Ferrero", Lathisms, 2017, retrieved 2024-10-23
- 1 2 3 4 Cobb, Timia (December 15, 2021), Daniela Ferrero honored as an Association for Women in Mathematics class of 2022 fellow, Texas State University, retrieved 2024-10-23
- ↑ Hicks, Illya V.; Brimkov, Boris (2024), "The many face(t)s of zero forcing", Notices of the American Mathematical Society, 71 (2): 167–173, doi:10.1090/noti2874, MR 4693614
- ↑ Daniela Ferrero at the Mathematics Genealogy Project
- ↑ Curriculum vitae, Texas State University, retrieved 2024-10-23