Conductor or conduction may refer to:
In graph theory, a planar graph is a graph that can be embedded in the plane, i.e., it can be drawn on the plane in such a way that its edges intersect only at their endpoints. In other words, it can be drawn in such a way that no edges cross each other. Such a drawing is called a plane graph or planar embedding of the graph. A plane graph can be defined as a planar graph with a mapping from every node to a point on a plane, and from every edge to a plane curve on that plane, such that the extreme points of each curve are the points mapped from its end nodes, and all curves are disjoint except on their extreme points.

In mathematics, a semigroup is an algebraic structure consisting of a set together with an associative internal binary operation on it.
Edge or EDGE may refer to:

In mathematics, genus has a few different, but closely related, meanings. Intuitively, the genus is the number of "holes" of a surface. A sphere has genus 0, while a torus has genus 1.
The term regular can mean normal or in accordance with rules. It may refer to:

In mathematics, a knot is an embedding of the circle S1 into three-dimensional Euclidean space, R3. Often two knots are considered equivalent if they are ambient isotopic, that is, if there exists a continuous deformation of R3 which takes one knot to the other.
Subdivision may refer to:
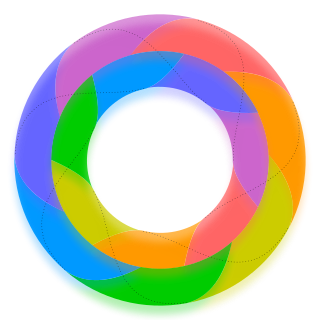
In graph theory, the Heawood conjecture or Ringel–Youngs theorem gives a lower bound for the number of colors that are necessary for graph coloring on a surface of a given genus. For surfaces of genus 0, 1, 2, 3, 4, 5, 6, 7, ..., the required number of colors is 4, 7, 8, 9, 10, 11, 12, 12, .... OEIS: A000934, the chromatic number or Heawood number.
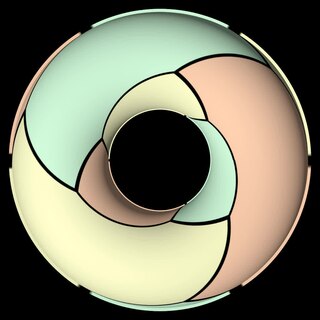
In mathematics, topological graph theory is a branch of graph theory. It studies the embedding of graphs in surfaces, spatial embeddings of graphs, and graphs as topological spaces. It also studies immersions of graphs.

In the mathematical field of graph theory, a toroidal graph is a graph that can be embedded on a torus. In other words, the graph's vertices can be placed on a torus such that no edges cross.
209 is the natural number following 208 and preceding 210.

In topological graph theory, an embedding of a graph on a surface is a representation of on in which points of are associated with vertices and simple arcs are associated with edges in such a way that:
In mathematics, a cancellative semigroup is a semigroup having the cancellation property. In intuitive terms, the cancellation property asserts that from an equality of the form a·b = a·c, where · is a binary operation, one can cancel the element a and deduce the equality b = c. In this case the element being cancelled out is appearing as the left factors of a·b and a·c and hence it is a case of the left cancellation property. The right cancellation property can be defined analogously. Prototypical examples of cancellative semigroups are the positive integers under addition or multiplication. Cancellative semigroups are considered to be very close to being groups because cancellability is one of the necessary conditions for a semigroup to be embeddable in a group. Moreover, every finite cancellative semigroup is a group. One of the main problems associated with the study of cancellative semigroups is to determine the necessary and sufficient conditions for embedding a cancellative semigroup in a group.
In mathematics, a numerical semigroup is a special kind of a semigroup. Its underlying set is the set of all nonnegative integers except a finite number and the binary operation is the operation of addition of integers. Also, the integer 0 must be an element of the semigroup. For example, while the set {0, 2, 3, 4, 5, 6, ...} is a numerical semigroup, the set {0, 1, 3, 5, 6, ...} is not because 1 is in the set and 1 + 1 = 2 is not in the set. Numerical semigroups are commutative monoids and are also known as numerical monoids.

Michael Grain Crandall is an American mathematician, specializing in differential equations.

John Cameron Urschel is a Canadian-American mathematician, former professional American football guard and center and chess player. He played college football at Penn State and was drafted by the Baltimore Ravens in the fifth round of the 2014 NFL Draft. Urschel played his entire NFL career with Baltimore before announcing his retirement on July 27, 2017, at 26 years old.

In graph theory, a Xuong tree is a spanning tree of a given graph with the property that, in the remaining graph , the number of connected components with an odd number of edges is as small as possible. They are named after Nguyen Huy Xuong, who used them to characterize the cellular embeddings of a given graph having the largest possible genus.
Zdeněk Hedrlín was a Czech mathematician, specializing in universal algebra and combinatorial theory, both in pure and applied mathematics.









