In geometry, an icosidodecahedron or pentagonal gyrobirotunda is a polyhedron with twenty (icosi) triangular faces and twelve (dodeca) pentagonal faces. An icosidodecahedron has 30 identical vertices, with two triangles and two pentagons meeting at each, and 60 identical edges, each separating a triangle from a pentagon. As such, it is one of the Archimedean solids and more particularly, a quasiregular polyhedron.
In geometry, a polyhedral compound is a figure that is composed of several polyhedra sharing a common centre. They are the three-dimensional analogs of polygonal compounds such as the hexagram.

In geometry, the rhombicosidodecahedron is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed of two or more types of regular polygon faces.

In geometry, a truncated icosidodecahedron, rhombitruncated icosidodecahedron, great rhombicosidodecahedron, omnitruncated dodecahedron or omnitruncated icosahedron is an Archimedean solid, one of thirteen convex, isogonal, non-prismatic solids constructed by two or more types of regular polygon faces.
In geometry, a uniform polyhedron has regular polygons as faces and is vertex-transitive—there is an isometry mapping any vertex onto any other. It follows that all vertices are congruent. Uniform polyhedra may be regular, quasi-regular, or semi-regular. The faces and vertices don't need to be convex, so many of the uniform polyhedra are also star polyhedra.

In geometry, the great dirhombicosidodecahedron (or great snub disicosidisdodecahedron) is a nonconvex uniform polyhedron, indexed last as U75. It has 124 faces (40 triangles, 60 squares, and 24 pentagrams), 240 edges, and 60 vertices.

In geometry, the small stellated dodecahedron is a Kepler-Poinsot polyhedron, named by Arthur Cayley, and with Schläfli symbol {5⁄2,5}. It is one of four nonconvex regular polyhedra. It is composed of 12 pentagrammic faces, with five pentagrams meeting at each vertex.

In geometry, the great stellated dodecahedron is a Kepler–Poinsot polyhedron, with Schläfli symbol {5⁄2,3}. It is one of four nonconvex regular polyhedra.

In geometry, the great icosidodecahedron is a nonconvex uniform polyhedron, indexed as U54. It has 32 faces (20 triangles and 12 pentagrams), 60 edges, and 30 vertices. It is given a Schläfli symbol r{3,5⁄2}. It is the rectification of the great stellated dodecahedron and the great icosahedron. It was discovered independently by Hess (1878), Badoureau (1881) and Pitsch (1882).

In geometry, the nonconvex great rhombicosidodecahedron is a nonconvex uniform polyhedron, indexed as U67. It has 62 faces (20 triangles, 30 squares and 12 pentagrams), 120 edges, and 60 vertices. It is also called the quasirhombicosidodecahedron. It is given a Schläfli symbol rr{5⁄3,3}. Its vertex figure is a crossed quadrilateral.

In geometry, a truncation is an operation in any dimension that cuts polytope vertices, creating a new facet in place of each vertex. The term originates from Kepler's names for the Archimedean solids.

In geometry, an alternation or partial truncation, is an operation on a polygon, polyhedron, tiling, or higher dimensional polytope that removes alternate vertices.

The compound of five cubes is one of the five regular polyhedral compounds. It was first described by Edmund Hess in 1876.

In geometry, a uniform star polyhedron is a self-intersecting uniform polyhedron. They are also sometimes called nonconvex polyhedra to imply self-intersecting. Each polyhedron can contain either star polygon faces, star polygon vertex figures, or both.
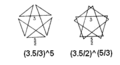
In geometry, the small complex icosidodecahedron is a degenerate uniform star polyhedron. Its edges are doubled, making it degenerate. The star has 32 faces, 60 (doubled) edges and 12 vertices and 4 sharing faces. The faces in it are considered as two overlapping edges as topological polyhedron.
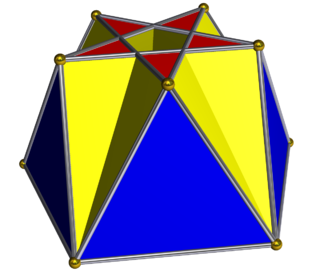
In geometry, the pentagrammic cuploid or pentagrammmic semicupola is the simplest of the infinite family of cuploids. It can be obtained as a slice of the small complex rhombicosidodecahedron. As in all cupolae, the base polygon has twice as many edges and vertices as the top; but in this case the base polygon is a degenerate {10/2} decagram, as the top is a {5/2} pentagram. Hence, the degenerate base is withdrawn and the triangles are connected to the squares instead.

In geometry, the crossed pentagonal cuploid or crossed pentagonal semicupola is one member of the infinite family of cuploids. It can be obtained as a slice of the great complex rhombicosidodecahedron. As in all cupolae, the base polygon has twice as many edges and vertices as the top; but in this case the base polygon is a degenerate {10/4} decagram, as the top is a {5/4} pentagon. Hence, the degenerate base is withdrawn and the triangles are connected to the squares instead.

The expanded icosidodecahedron is a polyhedron, constructed as an expanded icosidodecahedron. It has 122 faces: 20 triangles, 60 squares, 12 pentagons, and 30 rhombs. The 120 vertices exist at two sets of 60, with a slightly different distance from its center.