Hall plane

Last updated

In mathematics, a Hall plane is a non-Desarguesian projective plane constructed by Marshall Hall Jr. (1943). [1] There are examples of order p2n for every prime p and every positive integer n provided p2n> 4. [2]

Contents

Algebraic construction via Hall systems

The original construction of Hall planes was based on the Hall quasifield (also called a Hall system), H of order p2n for p a prime. The creation of the plane from the quasifield follows the standard construction (see quasifield for details).

To build a Hall quasifield, start with a Galois field, F = GF(pn) for p a prime and a quadratic irreducible polynomial f(x) = x2 − rx − s over F. Extend H = F × F, a two-dimensional vector space over F, to a quasifield by defining a multiplication on the vectors by (a, b) ∘ (c, d) = (ac − bd−1f(c), ad − bc + br) when d ≠ 0 and (a, b) ∘ (c, 0) = (ac, bc) otherwise.

Writing the elements of H in terms of a basis ⟨1, λ⟩, that is, identifying (x, y) with x + λy as x and y vary over F, we can identify the elements of F as the ordered pairs (x, 0), i.e. x + λ0. The properties of the defined multiplication which turn the right vector space H into a quasifield are:

  1. every element α of H not in F satisfies the quadratic equation f(α) = 0;
  2. F is in the kernel of H (meaning that (α + β)c = αc + βc, and (αβ)c = α(βc) for all α, β in H and all c in F); and
  3. every element of F commutes (multiplicatively) with all the elements of H. [3]

Derivation

Another construction that produces Hall planes is obtained by applying derivation to Desarguesian planes.

A process, due to T. G. Ostrom, which replaces certain sets of lines in a projective plane by alternate sets in such a way that the new structure is still a projective plane is called derivation. We give the details of this process. [4] Start with a projective plane π of order n2 and designate one line ℓ as its line at infinity. Let A be the affine plane π∖ℓ. A set D of n + 1 points of ℓ is called a derivation set if for every pair of distinct points X and Y of A which determine a line meeting ℓ in a point of D, there is a Baer subplane containing X, Y and D (we say that such Baer subplanes belong to D.) Define a new affine plane D(A) as follows: The points of D(A) are the points of A. The lines of D(A) are the lines of π which do not meet ℓ at a point of D (restricted to A) and the Baer subplanes that belong to D (restricted to A). The set D(A) is an affine plane of order n2 and it, or its projective completion, is called a derived plane. [5]

Properties

  1. Hall planes are translation planes.
  2. All finite Hall planes of the same order are isomorphic.
  3. Hall planes are not self-dual.
  4. All finite Hall planes contain subplanes of order 2 (Fano subplanes).
  5. All finite Hall planes contain subplanes of order different from 2.
  6. Hall planes are André planes.

Hall plane of order 9

Hall plane of order 9
Order9
Lenz–Barlotti class IVa.3
Automorphisms28 × 35 × 5
Point orbit lengths10, 81
Line orbit lengths1, 90
Properties Translation plane

The Hall plane of order 9 is the smallest Hall plane, and one of the three smallest examples of a finite non-Desarguesian projective plane, along with its dual and the Hughes plane of order 9. [6]

Construction

While usually constructed in the same way as other Hall planes, the Hall plane of order 9 was actually found earlier by Oswald Veblen and Joseph Wedderburn in 1907. [7] There are four quasifields of order nine which can be used to construct the Hall plane of order nine. Three of these are Hall systems generated by the irreducible polynomials f(x) = x2 + 1, g(x) = x2 − x − 1 or h(x) = x2 + x − 1. [8] The first of these produces an associative quasifield, [9] that is, a near-field, and it was in this context that the plane was discovered by Veblen and Wedderburn. This plane is often referred to as the nearfield plane of order nine.

Properties

Automorphism Group

The Hall plane of order 9 is the unique projective plane, finite or infinite, which has Lenz–Barlotti class IVa.3. [10] Its automorphism group acts on its (necessarily unique) translation line imprimitively, having 5 pairs of points that the group preserves set-wise; the automorphism group acts as S5 on these 5 pairs. [11]

Unitals

The Hall plane of order 9 admits four inequivalent embedded unitals. [12] Two of these unitals arise from Buekenhout's [13] constructions: one is parabolic, meeting the translation line in a single point, while the other is hyperbolic, meeting the translation line in 4 points. The latter of these two unitals was shown by Grüning [14] to also be embeddable in the dual Hall plane. Another of the unitals arises from the construction of Barlotti and Lunardon. [15] The fourth has an automorphism group of order 8 isomorphic to the quaternions, and is not part of any known infinite family.

Notes

  1. ↑ Hall (1943)
  2. ↑ Although the constructions will provide a projective plane of order 4, the unique such plane is Desarguesian and is generally not considered to be a Hall plane.
  3. ↑ Hughes & Piper (1973 , p. 183)
  4. ↑ Hughes & Piper (1973 , pp. 202–218, Chapter X. Derivation)
  5. ↑ Hughes & Piper (1973 , p. 203, Theorem 10.2)
  6. ↑ Moorhouse, G. Eric (2017), Projective Planes of Small Order explicitly lists the incidence structure of these planes.
  7. ↑ Veblen, Oswald; Wedderburn, Joseph H.M. (1907), "Non-Desarguesian and non-Pascalian geometries" (PDF), Transactions of the American Mathematical Society , 8 (3): 379–388, doi: 10.2307/1988781 , JSTOR   1988781
  8. ↑ Stevenson, Frederick W. (1972), Projective Planes, San Francisco: W.H. Freeman and Company, pp. 333–334, ISBN   0-7167-0443-9
  9. ↑ D. Hughes and F. Piper (1973). Projective Planes. Springer-Verlag. p. 186. ISBN   0-387-90044-6.
  10. ↑ Dembowski, Peter (1968). Finite Geometries : Reprint of the 1968 Edition. Berlin, Heidelberg: Springer Berlin Heidelberg. p. 126. ISBN   978-3-642-62012-6. OCLC   851794158.
  11. ↑ André, Johannes (1955-12-01). "Projektive Ebenen über Fastkörpern". Mathematische Zeitschrift (in German). 62 (1): 137–160. doi:10.1007/BF01180628. ISSN   1432-1823. S2CID   122641224.
  12. ↑ Penttila, Tim; Royle, Gordon F. (1995-11-01). "Sets of type (m, n) in the affine and projective planes of order nine". Designs, Codes and Cryptography. 6 (3): 229–245. doi:10.1007/BF01388477. ISSN   1573-7586. S2CID   43638589.
  13. ↑ Buekenhout, F. (July 1976). "Existence of unitals in finite translation planes of order q2 with a kernel of order q". Geometriae Dedicata. 5 (2). doi:10.1007/BF00145956. ISSN   0046-5755. S2CID   123037502.
  14. ↑ Grüning, Klaus (1987-06-01). "A class of unitals of order q which can be embedded in two different planes of order q2". Journal of Geometry. 29 (1): 61–77. doi:10.1007/BF01234988. ISSN   1420-8997. S2CID   117872040.
  15. ↑ Barlotti, A.; Lunardon, G. (1979). "Una classe di unitals nei Δ-piani". Rivisita di Matematica della Università di Parma. 4: 781–785.

Related Research Articles

<span class="mw-page-title-main">Projective plane</span> Geometric concept of a 2D space with a "point at infinity" adjoined

In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect at a single point, but there are some pairs of lines that do not intersect. A projective plane can be thought of as an ordinary plane equipped with additional "points at infinity" where parallel lines intersect. Thus any two distinct lines in a projective plane intersect at exactly one point.

<span class="mw-page-title-main">Projective space</span> Completion of the usual space with "points at infinity"

In mathematics, the concept of a projective space originated from the visual effect of perspective, where parallel lines seem to meet at infinity. A projective space may thus be viewed as the extension of a Euclidean space, or, more generally, an affine space with points at infinity, in such a way that there is one point at infinity of each direction of parallel lines.

In mathematics, projective geometry is the study of geometric properties that are invariant with respect to projective transformations. This means that, compared to elementary Euclidean geometry, projective geometry has a different setting, projective space, and a selective set of basic geometric concepts. The basic intuitions are that projective space has more points than Euclidean space, for a given dimension, and that geometric transformations are permitted that transform the extra points to Euclidean points, and vice versa.

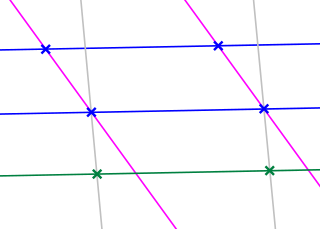
<span class="mw-page-title-main">Affine geometry</span> Euclidean geometry without distance and angles

In mathematics, affine geometry is what remains of Euclidean geometry when ignoring the metric notions of distance and angle.

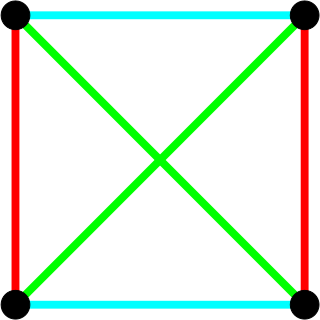
<span class="mw-page-title-main">Finite geometry</span> Geometric system with a finite number of points

A finite geometry is any geometric system that has only a finite number of points. The familiar Euclidean geometry is not finite, because a Euclidean line contains infinitely many points. A geometry based on the graphics displayed on a computer screen, where the pixels are considered to be the points, would be a finite geometry. While there are many systems that could be called finite geometries, attention is mostly paid to the finite projective and affine spaces because of their regularity and simplicity. Other significant types of finite geometry are finite Möbius or inversive planes and Laguerre planes, which are examples of a general type called Benz planes, and their higher-dimensional analogs such as higher finite inversive geometries.

<span class="mw-page-title-main">Desargues's theorem</span> Two triangles are in perspective axially if and only if they are in perspective centrally

In projective geometry, Desargues's theorem, named after Girard Desargues, states:

In geometry, an affine plane is a system of points and lines that satisfy the following axioms:

In mathematics, incidence geometry is the study of incidence structures. A geometric structure such as the Euclidean plane is a complicated object that involves concepts such as length, angles, continuity, betweenness, and incidence. An incidence structure is what is obtained when all other concepts are removed and all that remains is the data about which points lie on which lines. Even with this severe limitation, theorems can be proved and interesting facts emerge concerning this structure. Such fundamental results remain valid when additional concepts are added to form a richer geometry. It sometimes happens that authors blur the distinction between a study and the objects of that study, so it is not surprising to find that some authors refer to incidence structures as incidence geometries.

In mathematics, a translation plane is a projective plane which admits a certain group of symmetries. Along with the Hughes planes and the Figueroa planes, translation planes are among the most well-studied of the known non-Desarguesian planes, and the vast majority of known non-Desarguesian planes are either translation planes, or can be obtained from a translation plane via successive iterations of dualization and/or derivation.

<span class="mw-page-title-main">Joseph Wedderburn</span> Scottish mathematician

Joseph Henry Maclagan Wedderburn FRSE FRS was a Scottish mathematician, who taught at Princeton University for most of his career. A significant algebraist, he proved that a finite division algebra is a field, and part of the Artin–Wedderburn theorem on simple algebras. He also worked on group theory and matrix algebra.

In mathematics, an algebraic structure consisting of a non-empty set and a ternary mapping may be called a ternary system. A planar ternary ring (PTR) or ternary field is special type of ternary system used by Marshall Hall to construct projective planes by means of coordinates. A planar ternary ring is not a ring in the traditional sense, but any field gives a planar ternary ring where the operation is defined by . Thus, we can think of a planar ternary ring as a generalization of a field where the ternary operation takes the place of both addition and multiplication.

In mathematics, a quasifield is an algebraic structure where and are binary operations on , much like a division ring, but with some weaker conditions. All division rings, and thus all fields, are quasifields.

In mathematics, a near-field is an algebraic structure similar to a division ring, except that it has only one of the two distributive laws. Alternatively, a near-field is a near-ring in which there is a multiplicative identity and every non-zero element has a multiplicative inverse.

<span class="mw-page-title-main">Oval (projective plane)</span> Circle-like pointset in a geometric plane

In projective geometry an oval is a point set in a plane that is defined by incidence properties. The standard examples are the nondegenerate conics. However, a conic is only defined in a pappian plane, whereas an oval may exist in any type of projective plane. In the literature, there are many criteria which imply that an oval is a conic, but there are many examples, both infinite and finite, of ovals in pappian planes which are not conics.

In geometry, a Moufang plane, named for Ruth Moufang, is a type of projective plane, more specifically a special type of translation plane. A translation plane is a projective plane that has a translation line, that is, a line with the property that the group of automorphisms that fixes every point of the line acts transitively on the points of the plane not on the line. A translation plane is Moufang if every line of the plane is a translation line.

In mathematics, a non-Desarguesian plane is a projective plane that does not satisfy Desargues' theorem, or in other words a plane that is not a Desarguesian plane. The theorem of Desargues is true in all projective spaces of dimension not 2; in other words, the only projective spaces of dimension not equal to 2 are the classical projective geometries over a field. However, David Hilbert found that some projective planes do not satisfy it. The current state of knowledge of these examples is not complete.

In mathematics, a Hughes plane is one of the non-Desarguesian projective planes found by Hughes (1957). There are examples of order p2n for every odd prime p and every positive integer n.

In geometry, specifically projective geometry, a blocking set is a set of points in a projective plane that every line intersects and that does not contain an entire line. The concept can be generalized in several ways. Instead of talking about points and lines, one could deal with n-dimensional subspaces and m-dimensional subspaces, or even more generally, objects of type 1 and objects of type 2 when some concept of intersection makes sense for these objects. A second way to generalize would be to move into more abstract settings than projective geometry. One can define a blocking set of a hypergraph as a set that meets all edges of the hypergraph.

In geometry, a unital is a set of n3 + 1 points arranged into subsets of size n + 1 so that every pair of distinct points of the set are contained in exactly one subset. This is equivalent to saying that a unital is a 2-(n3 + 1, n + 1, 1) block design. Some unitals may be embedded in a projective plane of order n2 (the subsets of the design become sets of collinear points in the projective plane). In this case of embedded unitals, every line of the plane intersects the unital in either 1 or n + 1 points. In the Desarguesian planes, PG(2,q2), the classical examples of unitals are given by nondegenerate Hermitian curves. There are also many non-classical examples. The first and the only known unital with non prime power parameters, n=6, was constructed by Bhaskar Bagchi and Sunanda Bagchi. It is still unknown if this unital can be embedded in a projective plane of order 36, if such a plane exists.

Topological geometry deals with incidence structures consisting of a point set and a family of subsets of called lines or circles etc. such that both and carry a topology and all geometric operations like joining points by a line or intersecting lines are continuous. As in the case of topological groups, many deeper results require the point space to be (locally) compact and connected. This generalizes the observation that the line joining two distinct points in the Euclidean plane depends continuously on the pair of points and the intersection point of two lines is a continuous function of these lines.

References

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.