In mathematics,analytic geometry,also known as coordinate geometry or Cartesian geometry,is the study of geometry using a coordinate system. This contrasts with synthetic geometry.

Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces,otherwise known as smooth manifolds. It uses the techniques of differential calculus,integral calculus,linear algebra and multilinear algebra. The field has its origins in the study of spherical geometry as far back as antiquity. It also relates to astronomy,the geodesy of the Earth,and later the study of hyperbolic geometry by Lobachevsky. The simplest examples of smooth spaces are the plane and space curves and surfaces in the three-dimensional Euclidean space,and the study of these shapes formed the basis for development of modern differential geometry during the 18th and 19th centuries.

In mathematics,a hyperbola is a type of smooth curve lying in a plane,defined by its geometric properties or by equations for which it is the solution set. A hyperbola has two pieces,called connected components or branches,that are mirror images of each other and resemble two infinite bows. The hyperbola is one of the three kinds of conic section,formed by the intersection of a plane and a double cone. If the plane intersects both halves of the double cone but does not pass through the apex of the cones,then the conic is a hyperbola.

In geometry,Descartes' theorem states that for every four kissing,or mutually tangent,circles,the radii of the circles satisfy a certain quadratic equation. By solving this equation,one can construct a fourth circle tangent to three given,mutually tangent circles. The theorem is named after RenéDescartes,who stated it in 1643.

William Mark Goldman is a professor of mathematics at the University of Maryland,College Park. He received a B.A. in mathematics from Princeton University in 1977,and a Ph.D. in mathematics from the University of California,Berkeley in 1980.
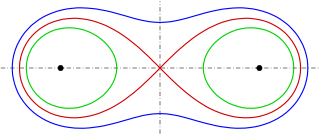
In geometry,a Cassini oval is a quartic plane curve defined as the locus of points in the plane such that the product of the distances to two fixed points (foci) is constant. This may be contrasted with an ellipse,for which the sum of the distances is constant,rather than the product. Cassini ovals are the special case of polynomial lemniscates when the polynomial used has degree 2.
In mathematics,a translation plane is a projective plane which admits a certain group of symmetries. Along with the Hughes planes and the Figueroa planes,translation planes are among the most well-studied of the known non-Desarguesian planes,and the vast majority of known non-Desarguesian planes are either translation planes,or can be obtained from a translation plane via successive iterations of dualization and/or derivation.

In geometry,a pencil is a family of geometric objects with a common property,for example the set of lines that pass through a given point in a plane,or the set of circles that pass through two given points in a plane.

In projective geometry an oval is a point set in a plane that is defined by incidence properties. The standard examples are the nondegenerate conics. However,a conic is only defined in a pappian plane,whereas an oval may exist in any type of projective plane. In the literature,there are many criteria which imply that an oval is a conic,but there are many examples,both infinite and finite,of ovals in pappian planes which are not conics.

In hyperbolic geometry,a hypercycle,hypercircle or equidistant curve is a curve whose points have the same orthogonal distance from a given straight line.
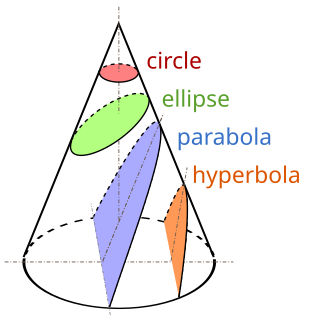
A conic section,conic or a quadratic curve is a curve obtained from a cone's surface intersecting a plane. The three types of conic section are the hyperbola,the parabola,and the ellipse;the circle is a special case of the ellipse,though it was sometimes called as a fourth type. The ancient Greek mathematicians studied conic sections,culminating around 200 BC with Apollonius of Perga's systematic work on their properties.

In geometry,a pole and polar are respectively a point and a line that have a unique reciprocal relationship with respect to a given conic section.
In mathematics,a non-Desarguesian plane is a projective plane that does not satisfy Desargues' theorem,or in other words a plane that is not a Desarguesian plane. The theorem of Desargues is true in all projective spaces of dimension not 2;in other words,the only projective spaces of dimension not equal to 2 are the classical projective geometries over a field. However,David Hilbert found that some projective planes do not satisfy it. The current state of knowledge of these examples is not complete.

In geometry,the unit hyperbola is the set of points (x,y) in the Cartesian plane that satisfy the implicit equation In the study of indefinite orthogonal groups,the unit hyperbola forms the basis for an alternative radial length

In mathematics,a Cayley–Klein metric is a metric on the complement of a fixed quadric in a projective space which is defined using a cross-ratio. The construction originated with Arthur Cayley's essay "On the theory of distance" where he calls the quadric the absolute. The construction was developed in further detail by Felix Klein in papers in 1871 and 1873,and subsequent books and papers. The Cayley–Klein metrics are a unifying idea in geometry since the method is used to provide metrics in hyperbolic geometry,elliptic geometry,and Euclidean geometry. The field of non-Euclidean geometry rests largely on the footing provided by Cayley–Klein metrics.
In geometry,a unital is a set of n3 + 1 points arranged into subsets of size n + 1 so that every pair of distinct points of the set are contained in exactly one subset. This is equivalent to saying that a unital is a 2-(n3 + 1,n + 1,1) block design. Some unitals may be embedded in a projective plane of order n2 (the subsets of the design become sets of collinear points in the projective plane). In this case of embedded unitals,every line of the plane intersects the unital in either 1 or n + 1 points. In the Desarguesian planes,PG(2,q2),the classical examples of unitals are given by nondegenerate Hermitian curves. There are also many non-classical examples. The first and the only known unital with non prime power parameters,n=6,was constructed by Bhaskar Bagchi and Sunanda Bagchi. It is still unknown if this unital can be embedded in a projective plane of order 36,if such a plane exists.

The Steiner conic or more precisely Steiner's generation of a conic,named after the Swiss mathematician Jakob Steiner,is an alternative method to define a non-degenerate projective conic section in a projective plane over a field.
Ascher Otto Wagner was an Austrian and British mathematician,specializing in the theory of finite groups and finite projective planes. He is known for the Dembowski–Wagner theorem.
A frequently studied problem in finite geometry is to identify ways in which an object can be covered by other simpler objects such as points,lines,and planes. In projective geometry,a specific instance of this problem that has numerous applications is determining whether,and how,a projective space can be covered by pairwise disjoint subspaces which have the same dimension;such a partition is called a spread. Specifically,a spread of a projective space ,where is an integer and a division ring,is a set of -dimensional subspaces,for some such that every point of the space lies in exactly one of the elements of the spread.

In mathematics,a spherical conic or sphero-conic is a curve on the sphere,the intersection of the sphere with a concentric elliptic cone. It is the spherical analog of a conic section in the plane,and as in the planar case,a spherical conic can be defined as the locus of points the sum or difference of whose great-circle distances to two foci is constant. By taking the antipodal point to one focus,every spherical ellipse is also a spherical hyperbola,and vice versa. As a space curve,a spherical conic is a quartic,though its orthogonal projections in three principal axes are planar conics. Like planar conics,spherical conics also satisfy a "reflection property":the great-circle arcs from the two foci to any point on the conic have the tangent and normal to the conic at that point as their angle bisectors.














