Related Research Articles

Hipparchus was a Greek astronomer, geographer, and mathematician. He is considered the founder of trigonometry, but is most famous for his incidental discovery of the precession of the equinoxes. Hipparchus was born in Nicaea, Bithynia, and probably died on the island of Rhodes, Greece. He is known to have been a working astronomer between 162 and 127 BC.
Kidinnu was a Chaldean astronomer and mathematician. Strabo of Amaseia called him Kidenas, Pliny the Elder called him Cidenas, and Vettius Valens called him Kidynas.
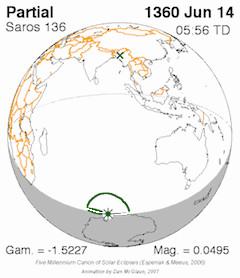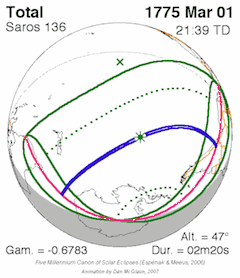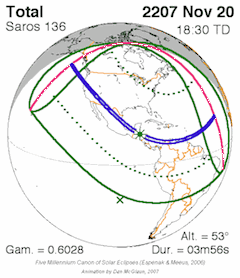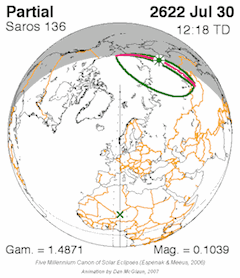
Eclipses may occur repeatedly, separated by certain intervals of time: these intervals are called eclipse cycles. The series of eclipses separated by a repeat of one of these intervals is called an eclipse series.
The saros is a period of exactly 223 synodic months, approximately 6585.3211 days, or 18 years, 10, 11, or 12 days, and 8 hours, that can be used to predict eclipses of the Sun and Moon. One saros period after an eclipse, the Sun, Earth, and Moon return to approximately the same relative geometry, a near straight line, and a nearly identical eclipse will occur, in what is referred to as an eclipse cycle. A sar is one half of a saros.

The Antikythera mechanism is an Ancient Greek hand-powered orrery, described as the oldest known example of an analogue computer used to predict astronomical positions and eclipses decades in advance. It could also be used to track the four-year cycle of athletic games which was similar to an Olympiad, the cycle of the ancient Olympic Games.
Nabû-nāṣir was the king of Babylon from 747 to 734 BC. He deposed a foreign Chaldean usurper named Nabu-shuma-ishkun, bringing native rule back to Babylon after twenty-three years of Chaldean rule. His reign saw the beginning of a new era characterized by the systematic maintenance of chronologically precise historical records. Both the Babylonian Chronicle and the Ptolemaic Canon begin with his accession to the throne. He was contemporary with the Assyrian kings Aššur-nirarī V and Tiglath-Pileser III, the latter under whom he became a vassal, and the Elamite kings Humban-Tahrah I and Humban-Nikaš I.
The inex is an eclipse cycle of 10,571.95 days. The cycle was first described in modern times by Crommelin in 1901, but was named by George van den Bergh who studied it in detail half a century later. It has been suggested that the cycle was known to Hipparchos. One inex after an eclipse of a particular saros series there will be an eclipse in the next saros series, unless the latter saros series has come to an end.

A lunar node is either of the two orbital nodes of the Moon, that is, the two points at which the orbit of the Moon intersects the ecliptic. The ascending node is where the Moon moves into the northern ecliptic hemisphere, while the descending node is where the Moon enters the southern ecliptic hemisphere.
Lunar theory attempts to account for the motions of the Moon. There are many small variations in the Moon's motion, and many attempts have been made to account for them. After centuries of being problematic, lunar motion can now be modeled to a very high degree of accuracy.
Oenopides of Chios was an ancient Greek geometer and astronomer, who lived around 450 BCE.

Babylonian astronomy was the study or recording of celestial objects during the early history of Mesopotamia.

The theory of tides is the application of continuum mechanics to interpret and predict the tidal deformations of planetary and satellite bodies and their atmospheres and oceans under the gravitational loading of another astronomical body or bodies.

A total lunar eclipse will take place on June 17, 2076. The moon will pass through the center of the Earth's shadow. While the visual effect of a total eclipse is variable, the Moon may be stained a deep orange or red color at maximum eclipse. With a gamma value of only -0.0452 and an umbral eclipse magnitude of 1.7943, this is the second greatest eclipse in Saros series 131 as well as the largest and darkest lunar eclipse between June 26, 2029 and June 28, 2094. Overall, it will be the third largest and darkest lunar eclipse of the 21st century. While it will have similar values to the lunar eclipse of July 16, 2000, totality will not last over 106 minutes due to the moon's relatively large apparent size as seen from Earth and greater speed in its elliptical orbit. Totality's expected to last 100 minutes 34 seconds from 9:11:39 to 10:52:15 with the greatest point at 10:01:57 UTC.
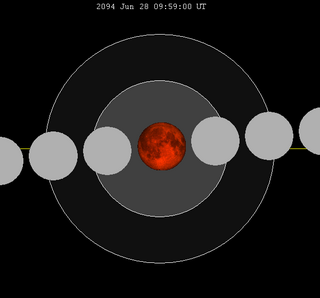
A total lunar eclipse will take place on June 28, 2094. The moon will pass through the center of the Earth's shadow. While the visual effect of a total eclipse is variable, the Moon may be stained a deep orange or red color at maximum eclipse. With a gamma value of only 0.0288 and an umbral eclipse magnitude of 1.8234, this is the greatest eclipse in Saros series 131 as well as the second largest and darkest lunar eclipse of the 21st century.
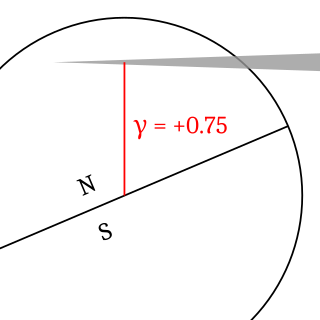
Gamma of an eclipse describes how centrally the shadow of the Moon or Earth strikes the other body. This distance, measured at the moment when the axis of the shadow cone passes closest to the center of the Earth or Moon, is stated as a fraction of the equatorial radius of the Earth or Moon.

An eclipse season is the period, roughly every six months, when eclipses occur. Eclipse seasons are the result of the axial parallelism of the Moon's orbital plane, just as Earth's weather seasons are the result of the axial parallelism of Earth's tilted axis as it orbits around the Sun. During the season, the "lunar nodes" – the line where the Moon's orbital plane intersects with the Earth's orbital plane – aligns with the Sun and Earth, such that a solar eclipse is formed during the new moon phase and a lunar eclipse is formed during the full moon phase.

The Babylonian astronomical diaries are a collection of Babylonian cuneiform texts that contain systematic records of astronomical observations and political events as well as predictions, based on astronomical observations. They also include other information such as commodity prices for particular dates and weather reports.

In lunar calendars, a lunar month is the time between two successive syzygies of the same type: new moons or full moons. The precise definition varies, especially for the beginning of the month.

Dodecatemoria are subdivisions of the twelve signs of the Zodiac into a further twelve parts each. These can be said to form a "micro-zodiac" of 144 dodecatemoria, each corresponding to 2.5° of the ecliptic. In an alternate usage, the dodecamorion refers to a point on the ecliptic reached by the addition of twelve times a given number of degrees within a sign, either to the original degree, or to the beginning of the sign.

A total eclipse of the Moon occurs on Wednesday June 9, 2123, with maximum eclipse at 05:06 UT. A dramatic total eclipse lasting 106 minutes and 6 seconds will plunge the full Moon into deep darkness, as it passes right through the centre of the Earth's umbral shadow. While the visual effect of a total eclipse is variable, the Moon may be stained a deep orange or red colour at maximum eclipse. This will be a great spectacle for everyone who sees it. The partial eclipse will last for 3 hours and 56 minutes in total. The penumbral eclipse lasts for 6 hours and 14 minutes. Maximum eclipse is at 05:06:28 UT. This will be the longest Total Lunar Eclipse since 16 July 2000, and the longest one until 12 May 2264 and 27 July 3107, though the eclipse on June 19, 2141 will be nearly identical in all aspects. This will also be the longest of the 22nd century and the second longest of the 3rd millennium. The eclipse on June 19, 2141 will be the second longest of the 22nd century and the third longest of the third millennium.
References
- 1 2 3 4 5 Curriculum vitae , retrieved 2018-11-06
- 1 2 3 Steele, John; Ossendrijver, Mathieu (2017), "In honor of Lis Brack-Bernsen", Studies on the Ancient Exact Sciences in Honour of Lis Brack-Bernsen, Berlin Studies of the Ancient World, vol. 44, Edition Topoi, pp. 263–269, doi:10.17171/3-44-14
- 1 2 3 Studies on the Ancient Exact Sciences in Honour of Lis Brack-Bernsen, Edition Topoi, 2017
- ↑ Brack-Bernsen, Lis (2010), "Methods for understanding and reconstructing Babylonian predicting rule", Writings of Early Scholars in the Ancient Near East, Egypt, Rome, and Greece: 277–297, doi:10.1515/9783110229936.4.277, ISBN 978-3-11-022992-9
- ↑ Sachs, Abraham (1948). "A classification of the Babylonian astronomical tablets of the Seleucid period". Journal of Cuneiform Studies. 2 (4): 271–290. doi:10.2307/3515929. JSTOR 3515929. S2CID 164038422.
- 1 2 Huber, Peter J.; Steele, John M. (2007). "Babylonian lunar six tablets". Sciamvs. 8: 3–36.
- 1 2 Shnider, Steven (2017). "Britton's Theory of the Creation of Column Φ". Archive for History of Exact Sciences. 71 (3): 279–318. doi:10.1007/s00407-017-0189-4. S2CID 125603853.
- ↑ Steele, John M. (2000). "Babylonian lunar theory reconsidered". Isis. 91 (1): 125–126. doi:10.1086/384630. S2CID 146183565.
- ↑ Brack-Bernsen, Lis (2020). "Babylonian astronomy: a new understanding of column Φ". Archive for History of Exact Sciences. 74 (6): 605–640. doi:10.1007/s00407-020-00254-z. S2CID 225502955.
- ↑ "Prof. Dr. Lis Brack-Bernsen", Mitgliederverzeichnis, Academy of Sciences Leopoldina , retrieved 2018-11-06