Low thrust relative orbital transfer

Last updated February 28, 2023

In orbital mechanics, low-thrust relative transfer is an orbital maneuver in which a chaser spacecraft covers a specific relative distance relative to the target spacecraft using continuous low-thrust system with specific impulse of the order of 4000-8000s.^[1] This is in contrast to conventional impulsive transfers in the orbit which uses thermal rocket engines to develop impulse of the order of 300-400s. Such type of transfer uses low-thrust propulsion systems such as electrically powered spacecraft propulsion and solar sail.

Low-thrust relative transfer uses the orbital relative motion equations which are the non-linear set of equations that describes the motion of the chaser spacecraft relative to the target in terms of displacements along the respective axis of the accelerated frame of reference fixed on the target spacecraft. In 1960, W. H. Clohessy and R. S. Wiltshire published the Clohessy-Wiltshire equations,^[2] which presents a rather simplified model of orbital relative motion, in which the target is in a circular orbit, and the chaser spacecraft is in an elliptical or circular orbit. Since, the quantity of available thrust is limited, the transfer is occasionally posed as an optimal control problem subjected to the required objective and constraints.

Explanation

Relative motion in the orbit means the motion of a spacecraft orbiting a planet relative to the other spacecraft orbiting the same planet. There can be one primary spacecraft known as the target and the other spacecraft with the task of performing the required maneuver relative to the target. Based on the mission requirement, the various relative orbital transfers can be rendezvous and docking operations, and maintaining station relative to the target. Unlike using a thrust-impulse to instantaneously change the velocity of the spacecraft, in non-impulsive transfer, there is a continuous application of thrust, so that, the spacecraft changes its direction gradually. Non-impulsive transfers relies on the low-thrust propulsion for the operation. Some of the mentionable low-thrust propulsion methods are, ionic propulsion, Hall-effect thruster and solar-sail systems. The electrostatic ion thruster uses high-voltage electrodes to accelerate ions with electrostatic forces, and achieve a specific impulse within the range of 4000-8000s.

Mathematical Models

The continuous low-thrust relative transfer can be described in mathematical form by adding components of specific thrust which will act as control input in the equations of motion model for relative orbital transfer. Although a number of linearized models have been developed since 1960s which gives simplified set of equations, one popular model was developed by W. H. Clohessy and R. S. Wiltshire, and is modified to account for continuous motion and can be written as:

${\ddot {x}}=3n^{2}x+2n{\dot {y}}+u_{x}$

${\ddot {y}}=-2n{\dot {x}}+u_{y}$

${\ddot {z}}=-n^{2}z+u_{z}$

where:

$x$ , $y$ and $z$ are the relative distance component of the chaser in the target fixed frame of reference
$u_{x},u_{y}$ and $u_{z}$ are the specific thrust in the form of control input along $x$ , $y$ and $z$ -axis of the target fixed frame of reference
$n$ is the orbital frequency of the target orbit

Optimal relative transfers

Since, in continuous low-thrust transfers the thrust availability is limited, such type of transfers are usually subjected to certain performance index and final state constraints, posing the transfer as an optimal control problem with defined boundary conditions.^[3] For the transfer to have optimal control input expenditure, the problem can be written as:

$J={\frac {1}{2}}\int _{t_{0}}^{t_{f}}({\vec {u}}^{T}\cdot R\cdot {\vec {u}})dt$

subjected to dynamics of the relative transfer:

${\dot {\vec {x}}}=A{\vec {x}}+B{\vec {u}}$

and boundary conditions:

${\vec {x}}(t_{0})={\vec {x}}_{0}$

${\vec {x}}(t_{f})={\vec {x}}_{f}$

where:

${\vec {x}}$ is the state-vector defined as ${\vec {x}}={\begin{bmatrix}x&{\dot {x}}&y&{\dot {y}}&z&{\dot {z}}\end{bmatrix}}^{T}$
${\vec {u}}$ is the control input vector defined as ${\vec {u}}={\begin{bmatrix}u_{x}&u_{y}&u_{z}\end{bmatrix}}^{T}$
$R$ is the weight matrix
$A$ is the state matrix obtained from the Clohessy-Wiltshire equations, such that, $A={\begin{bmatrix}0&1&0&0&0&0\\3n^{2}&0&0&2n&0&0\\0&0&0&1&0&0\\0&-2n&0&0&0&0\\0&0&0&0&0&1\\0&0&0&0&-n^{2}&0\\\end{bmatrix}}$
$B$ is the input matrix, such that, $B={\begin{bmatrix}0&0&0\\1&0&0\\0&0&0\\0&1&0\\0&0&0\\0&0&1\\\end{bmatrix}}$
$t_{0}$ is the time of start of transfer
$t_{f}$ is the time of end of transfer
${\vec {x}}_{0}$ is the initial value of the state vector
${\vec {x}}_{f}$ is the final value of the state vector

Sometimes, it is also useful to subject the system to control constraints because in case of continuous low-thrust transfer, there are always bounds on the availability of thrust. Hence, if the maximum quantity of thrust available is $u_{max}$ , then, an additional inequality constraint can be imposed on the optimal control problem posed above as:

$||{\vec {u}}(t)||\leq u_{max}$

Additionally, if the relative transfer is occurring such that the chaser and the target spacecraft are very close to each other, the collision-avoidance constraints can also be employed in the optimal control problem in the form of a minimum relative distance, $r_{min}$ as:

$||{\vec {x}}(t)||\geq r_{min}$

and because of obvious reasons, the final value of state-vector cannot be less than $r_{min}$ .

Related Research Articles

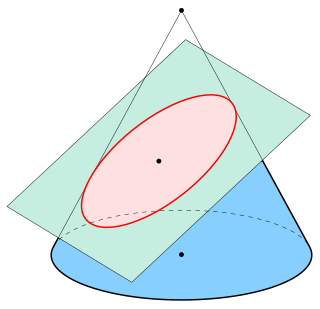
<span class="mw-page-title-main">Ellipse</span> Plane curve: conic section

In mathematics, an ellipse is a plane curve surrounding two focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. It generalizes a circle, which is the special type of ellipse in which the two focal points are the same. The elongation of an ellipse is measured by its eccentricity $, a number ranging from to .$

In physics, the Lorentz transformations are a six-parameter family of linear transformations from a coordinate frame in spacetime to another frame that moves at a constant velocity relative to the former. The respective inverse transformation is then parameterized by the negative of this velocity. The transformations are named after the Dutch physicist Hendrik Lorentz.

A magnetic sail is a proposed method of spacecraft propulsion that uses a static magnetic field to deflect a plasma wind of charged particles radiated by the Sun or a Star thereby transferring momentum to accelerate or decelerate a spacecraft. Most approaches require little to no propellant and thus are a form of Field propulsion. A magnetic sail could also thrust against a planetary ionosphere or magnetosphere. Important use cases are: a modest force from the solar wind sustainable for a long period of time; deceleration in the interstellar medium and the plasma wind of a destination Star following interstellar travel at relativistic speeds achieved by some other means; and efficient deceleration in a planetary ionosphere. Plasma characteristics for the Solar wind, a planetary ionosphere and the interstellar medium and the specifics of the magnetic sail design determine achievable performance; such as, thrust, required power and mass.

Kinematics is a subfield of physics, developed in classical mechanics, that describes the motion of points, bodies (objects), and systems of bodies without considering the forces that cause them to move. Kinematics, as a field of study, is often referred to as the "geometry of motion" and is occasionally seen as a branch of mathematics. A kinematics problem begins by describing the geometry of the system and declaring the initial conditions of any known values of position, velocity and/or acceleration of points within the system. Then, using arguments from geometry, the position, velocity and acceleration of any unknown parts of the system can be determined. The study of how forces act on bodies falls within kinetics, not kinematics. For further details, see analytical dynamics.

Orbital elements are the parameters required to uniquely identify a specific orbit. In celestial mechanics these elements are considered in two-body systems using a Kepler orbit. There are many different ways to mathematically describe the same orbit, but certain schemes, each consisting of a set of six parameters, are commonly used in astronomy and orbital mechanics.

In astronautics, the Hohmann transfer orbit is an orbital maneuver used to transfer a spacecraft between two orbits of different altitudes around a central body. Examples would be used for travel between low Earth orbit and the Moon, or another solar planet or asteroid. In the idealized case, the initial and target orbits are both circular and coplanar. The maneuver is accomplished by placing the craft into an elliptical transfer orbit that is tangential to both the initial and target orbits. The maneuver uses two impulsive engine burns: the first establishes the transfer orbit, and the second adjusts the orbit to match the target.

In continuum mechanics, vorticity is a pseudovector field that describes the local spinning motion of a continuum near some point, as would be seen by an observer located at that point and traveling along with the flow. It is an important quantity in the dynamical theory of fluids and provides a convenient framework for understanding a variety of complex flow phenomena, such as the formation and motion of vortex rings.

<span class="mw-page-title-main">Orbital mechanics</span> Field of classical mechanics concerned with the motion of spacecraft

Orbital mechanics or astrodynamics is the application of ballistics and celestial mechanics to the practical problems concerning the motion of rockets and other spacecraft. The motion of these objects is usually calculated from Newton's laws of motion and the law of universal gravitation. Orbital mechanics is a core discipline within space-mission design and control.

In the mathematical field of differential geometry, a metric tensor is an additional structure on a manifold $M$ that allows defining distances and angles, just as the inner product on a Euclidean space allows defining distances and angles there. More precisely, a metric tensor at a point $p$ of $M$ is a bilinear form defined on the tangent space at $p$ , and a metric tensor on $M$ consists of a metric tensor at each point $p$ of $M$ that varies smoothly with $p$ .

<span class="mw-page-title-main">Trajectory</span> Path of a moving object

A trajectory or flight path is the path that an object with mass in motion follows through space as a function of time. In classical mechanics, a trajectory is defined by Hamiltonian mechanics via canonical coordinates; hence, a complete trajectory is defined by position and momentum, simultaneously.

In physics and mathematics, the Lorentz group is the group of all Lorentz transformations of Minkowski spacetime, the classical and quantum setting for all (non-gravitational) physical phenomena. The Lorentz group is named for the Dutch physicist Hendrik Lorentz.

In spaceflight, an orbital maneuver is the use of propulsion systems to change the orbit of a spacecraft. For spacecraft far from Earth an orbital maneuver is called a deep-space maneuver (DSM).

A space rendezvous is a set of orbital maneuvers during which two spacecraft, one of which is often a space station, arrive at the same orbit and approach to a very close distance. Rendezvous requires a precise match of the orbital velocities and position vectors of the two spacecraft, allowing them to remain at a constant distance through orbital station-keeping. Rendezvous may or may not be followed by docking or berthing, procedures which bring the spacecraft into physical contact and create a link between them.

Spacecraft flight dynamics is the application of mechanical dynamics to model how the external forces acting on a space vehicle or spacecraft determine its flight path. These forces are primarily of three types: propulsive force provided by the vehicle's engines; gravitational force exerted by the Earth and other celestial bodies; and aerodynamic lift and drag.

Vector autoregression (VAR) is a statistical model used to capture the relationship between multiple quantities as they change over time. VAR is a type of stochastic process model. VAR models generalize the single-variable (univariate) autoregressive model by allowing for multivariate time series. VAR models are often used in economics and the natural sciences.

The perifocal coordinate (PQW) system is a frame of reference for an orbit. The frame is centered at the focus of the orbit, i.e. the celestial body about which the orbit is centered. The unit vectors $and lie in the plane of the orbit. is directed towards the periapsis of the orbit and has a true anomaly of 90 degrees past the periapsis. The third unit vector is the angular momentum vector and is directed orthogonal to the orbital plane such that:$

Orbit determination is the estimation of orbits of objects such as moons, planets, and spacecraft. One major application is to allow tracking newly observed asteroids and verify that they have not been previously discovered. The basic methods were discovered in the 17th century and have been continuously refined.

In mathematics, in the field of control theory, a Sylvester equation is a matrix equation of the form:

There are many ways to derive the Lorentz transformations utilizing a variety of physical principles, ranging from Maxwell's equations to Einstein's postulates of special relativity, and mathematical tools, spanning from elementary algebra and hyperbolic functions, to linear algebra and group theory.

The Clohessy–Wiltshire equations describe a simplified model of orbital relative motion, in which the target is in a circular orbit, and the chaser spacecraft is in an elliptical or circular orbit. This model gives a first-order approximation of the chaser's motion in a target-centered coordinate system. It is very useful in planning rendezvous of the chaser with the target.

References

↑ Grodzovskiy, G. L.; Ivanov, Yu. N.; Tokarev, V.V. (1964). The mechanics of low-thrust space flight. United States: NASA-TTF.
↑ Clohessy, W.H.; Wiltshire, R.S. (1960). "Terminal Guidance System for Satellite Rendezvous". Journal of the Aerospace Sciences. 27 (9): 653–658. doi:10.2514/8.8704 – via Aerospace Research Central.
↑ Kumar, Yajur (2016-05-25). Optimal Low Thrust Transfer for Relative Orbital Motion. doi:10.13140/rg.2.2.16899.91689.

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Grodzovskiy, G. L.; Ivanov, Yu. N.; Tokarev, V.V. (1964). The mechanics of low-thrust space flight. United States: NASA-TTF.

[2] Clohessy, W.H.; Wiltshire, R.S. (1960). "Terminal Guidance System for Satellite Rendezvous". Journal of the Aerospace Sciences. 27 (9): 653–658. doi:10.2514/8.8704 – via Aerospace Research Central.

[3] Kumar, Yajur (2016-05-25). Optimal Low Thrust Transfer for Relative Orbital Motion. doi:10.13140/rg.2.2.16899.91689.

[1]

[2]

[3]