Casting process simulation is a computational technique used in industry and metallurgy to model and analyze the metal-casting process. This technology allows engineers to predict and visualize the flow of molten metal, crystallization patterns, and potential defects in the casting before the start of the actual production process. By simulating the casting process, manufacturers can optimize mold design, reduce material consumption, and improve the quality of the final product.
The theoretical foundations of heat conduction, critically important for casting simulation, were established by Jean-Baptiste Joseph Fourier at the École polytechnique in Paris. His thesis "Analytical Theory of Heat," [1] awarded in 1822, laid the groundwork for all subsequent calculations of heat conduction and heat transfer in solid materials. Additionally, French physicist and engineer Claude-Louis Navier and Irish mathematician and physicist George Gabriel Stokes provided the foundations of fluid dynamics, which led to the development of the Navier-Stokes equations. [2] [3] Adolph Fick , working in the 19th century at the University of Zurich, developed the fundamental equations describing diffusion, published in 1855. [4]
The beginning of simulation in casting started in the 1950s when V. Pashkis used analog computers to predict the movement of the crystallization front. [5] The first use of digital computers to solve problems related to casting was carried out by Dr K. Fursund in 1962, who considered the penetration of steel into a sand mold. [6] A pioneering work by J. G. Hentzel and J. Keverian in 1965 was the two-dimensional simulation of the crystallization of steel castings, using a program developed by General Electric to simulate heat transfer. [7] In 1968, Ole Vestby was the first to use the finite difference method to program a 2D model that evaluated the temperature distribution during welding. [8]
The 1980s marked a significant increase in research and development activities around the topic of casting process simulation with contributions from various international groups, including J. T. Berry and R. D. Pielke in the United States, E. Niyama in Japan, W. Kurz in Lausanne, and F. Durand in Grenoble. An especially important role in advancing this field was played by Professor P. R. Sahm at the Aachen Foundry Institute. Key milestones of this period were the introduction of the "criterion function" by Hansen and Berry [9] in 1980, the Niyama criterion function [10] for the representation of central porosities in 1982, and the proposal of a criterion function for the detection of hot cracks in steel castings by E. Fehlner and P. N. Hansen in 1984. [11] In the late 1980s, the first capabilities for simulating mold filling were developed.
The 1990s focused on the simulation of stresses and strains in castings with significant contributions from Hattel and Hansen in 1990. This decade also saw efforts to predict microstructures and mechanical properties with the pioneering work of I. Svensson and M. Wessen in Sweden. [12] [13]
The production of casting is one of the most complex and multifaceted processes in metallurgy, requiring careful control and an understanding of a multitude of physical and chemical phenomena. To effectively manage this process and ensure the high quality of the final products, it is essential to have a deep understanding of the interaction of the various casting parameters. In this context, the mathematical modeling of casting acts as a critically important scientific tool, allowing for detailed analysis and optimization of the casting process based on mathematical principles. [14] [15] [16]
Mathematical modeling of casting is a complex process that involves the formulation and solution of mathematical equations that describe physical phenomena such as thermal conductivity, fluid dynamics, phase transformations, among others. To solve these equations, various numerical analysis methods are applied, among which the finite element method (FEM), [17] finite difference method (FDM), and finite volume method (FVM) hold a special place. Each of these methods has its particular characteristics and is applied depending on the specific modeling tasks and the requirements for precision and efficiency in the calculations.
Finite difference method (FDM): This method is based on differential equations of heat and mass transfer, which are approximated using finite difference relationships. [18] The advantage of the FDM is its simplicity and the ability to simplify the solution of multidimensional problems. However, the method has limitations in modeling the boundaries of complex areas and performs poorly for castings with thin walls. [19]
The finite element method and Finite volume method (FVM): [20] Both methods are based on integral equations of heat and mass transfer. They provide a good approximation of the boundaries and allow the use of elements with different discretizations. The main drawbacks are the need for a finite element generator, the complexity of the equations, and large requirements for memory and time resources.
Modifications of the FVM: These methods attempt to combine the simplicity of the FDM with a good approximation of the boundaries of the FEM. They have the potential to improve the approximation of boundaries between different materials and phases.[ citation needed ] [21]
The analysis of different methods of mathematical modeling of casting processes shows that the finite element method is one of the most reliable and optimal approaches for casting simulation. [22] Despite higher computational resource requirements and complexity in implementation compared to the finite difference method and finite volume method, the FEM provides high accuracy in modeling boundaries, complex geometries, and temperature fields, which is critically important for predicting defects in castings and optimizing casting processes. [23] [24]
Computer-aided engineering (CAE) systems for casting processes have long been used by foundries around the world as a "virtual foundry workshop," where it is possible to perform and verify any idea that arises in the minds of designers and technologists. [25] The global market for CAE for casting processes can already be considered established. [26]
Within the structure of the company for the development of the technology of a new casting, a computer-aided design department for casting processes is created, responsible for operating CAE systems for casting processes. Calculations are carried out by specialists of the department according to their job instructions, and interaction with other departments is regulated by technological design instructions. [27]
The process begins with the delivery of the 3D model and drawing of the part to the foundry technologists, who coordinate the casting configuration with the mechanical workshop and determine the margins. [28] Then, the technology is developed in the CAE department and transferred to the foundry workshop for experimental castings. The results are monitored, and if necessary, the castings are examined in the central laboratory of the factory. If defects are detected, an adjustment of the model parameters and the technological process is made in the CAE department, after which the technology is tested again in the workshop.[ citation needed ] [29]
This cycle is repeated until suitable castings are obtained, after which the technology is considered developed and implemented in mass production. [30] [31]
In the modern foundry industry, software for the simulation of casting processes is widely used. Among the multitude of software solutions available, it is worth mentioning the most prominent and widely used products: Procast, MAGMASOFT, and PoligonSoft.
ProCAST: a casting process modeling system using the finite element method, which provides the joint solution of temperature, hydrodynamics, and deformation problems, along with unique metallurgical capabilities, for all casting processes and casting alloys. In addition to the main aspects of casting production – filling, crystallization, and porosity prediction, ProCAST is capable of predicting the occurrence of deformations and residual stresses in the casting and can be used to analyze processes such as core making, centrifugal casting, lost wax casting, continuous casting. [32]
PoligonSoft: a casting process modeling system using the finite element method. Applicable for modeling almost any casting technology and any casting alloy. For a long time, PoligonSoft was the only casting process modeling system in the world that included a special model for calculating microporosity. [33] [34] To date, this model can be considered the most stable, and the results obtained with its help can satisfy the most demanding users. In many respects, PoligonSoft can be considered the Russian equivalent of the ProCAST system. [35]
MAGMASOFT: a casting process modeling system using the finite difference method. It allows analyzing thermal processes, mold filling, crystallization, and predicting defects in castings. [36] The program includes modules for different casting technologies and helps optimize casting parameters to improve product quality. MAGMASOFT is an effective tool for increasing the productivity and quality of casting production. [37]
The simulation of the casting process reflects the user's knowledge, who decides whether the filling system has led to an acceptable result. Optimization suggestions must come from the operator. The main problem is that all processes occur simultaneously and are interconnected: changes in one parameter affect many quality characteristics of the casting. [38]
Autonomous optimization, which began in the late 1980s, uses the simulation tool as a virtual testing ground, changing filling conditions and process parameters to find the optimal solution. [39] This allows evaluating numerous process parameters and their impact on process stability.
It is important to remember that only what can be modeled can be optimized. Optimization does not replace process knowledge or experience. [40] The simulation user must know the objectives and quality criteria necessary to achieve those objectives and formulate specific questions to the program to obtain quantitative solutions. [41]
A discrete element method (DEM), also called a distinct element method, is any of a family of numerical methods for computing the motion and effect of a large number of small particles. Though DEM is very closely related to molecular dynamics, the method is generally distinguished by its inclusion of rotational degrees-of-freedom as well as stateful contact, particle deformation and often complicated geometries. With advances in computing power and numerical algorithms for nearest neighbor sorting, it has become possible to numerically simulate millions of particles on a single processor. Today DEM is becoming widely accepted as an effective method of addressing engineering problems in granular and discontinuous materials, especially in granular flows, powder mechanics, ice and rock mechanics. DEM has been extended into the Extended Discrete Element Method taking heat transfer, chemical reaction and coupling to CFD and FEM into account.
Computational fluid dynamics (CFD) is a branch of fluid mechanics that uses numerical analysis and data structures to analyze and solve problems that involve fluid flows. Computers are used to perform the calculations required to simulate the free-stream flow of the fluid, and the interaction of the fluid with surfaces defined by boundary conditions. With high-speed supercomputers, better solutions can be achieved, and are often required to solve the largest and most complex problems. Ongoing research yields software that improves the accuracy and speed of complex simulation scenarios such as transonic or turbulent flows. Initial validation of such software is typically performed using experimental apparatus such as wind tunnels. In addition, previously performed analytical or empirical analysis of a particular problem can be used for comparison. A final validation is often performed using full-scale testing, such as flight tests.
Computer-aided engineering (CAE) is the general usage of technology to aid in tasks related to engineering analysis. Any use of technology to solve or assist engineering issues falls under this umbrella.
Molding sand, also known as foundry sand, is a sand that when moistened and compressed or oiled or heated tends to pack well and hold its shape. It is used in the process of sand casting for preparing the mold cavity.
A foundry is a factory that produces metal castings. Metals are cast into shapes by melting them into a liquid, pouring the metal into a mold, and removing the mold material after the metal has solidified as it cools. The most common metals processed are aluminum and cast iron. However, other metals, such as bronze, brass, steel, magnesium, and zinc, are also used to produce castings in foundries. In this process, parts of desired shapes and sizes can be formed.
Micromechanics is the analysis of heterogeneous materials including of composite, and anisotropic and orthotropic materials on the level of the individual constituents that constitute them and their interactions.
Soft-body dynamics is a field of computer graphics that focuses on visually realistic physical simulations of the motion and properties of deformable objects. The applications are mostly in video games and films. Unlike in simulation of rigid bodies, the shape of soft bodies can change, meaning that the relative distance of two points on the object is not fixed. While the relative distances of points are not fixed, the body is expected to retain its shape to some degree. The scope of soft body dynamics is quite broad, including simulation of soft organic materials such as muscle, fat, hair and vegetation, as well as other deformable materials such as clothing and fabric. Generally, these methods only provide visually plausible emulations rather than accurate scientific/engineering simulations, though there is some crossover with scientific methods, particularly in the case of finite element simulations. Several physics engines currently provide software for soft-body simulation.
Integrated Computational Materials Engineering (ICME) is an approach to design products, the materials that comprise them, and their associated materials processing methods by linking materials models at multiple length scales. Key words are "Integrated", involving integrating models at multiple length scales, and "Engineering", signifying industrial utility. The focus is on the materials, i.e. understanding how processes produce material structures, how those structures give rise to material properties, and how to select materials for a given application. The key links are process-structures-properties-performance. The National Academies report describes the need for using multiscale materials modeling to capture the process-structures-properties-performance of a material.
Directional solidification(DS) and progressive solidification are types of solidification within castings. Directional solidification is solidification that occurs from farthest end of the casting and works its way towards the sprue. Progressive solidification, also known as parallel solidification, is solidification that starts at the walls of the casting and progresses perpendicularly from that surface.
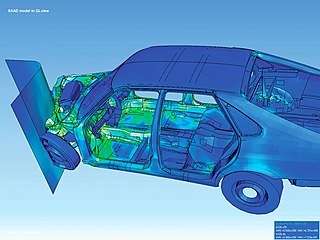
The finite element method (FEM) is a popular method for numerically solving differential equations arising in engineering and mathematical modeling. Typical problem areas of interest include the traditional fields of structural analysis, heat transfer, fluid flow, mass transport, and electromagnetic potential. Computers are usually used to perform the calculations required. With high-speed supercomputers, better solutions can be achieved, and are often required to solve the largest and most complex problems.
RF microwave CAE CAD is computer-aided design (CAD) using computer technology to aid in the design, modeling, and simulation of an RF or microwave product. It is a visual and symbol-based method of communication whose conventions are particular to RF/microwave engineering.
modeFRONTIER is a software solution for simulation process automation and design exploration developed by ESTECO, an Italian engineering software house. modeFRONTIER leverages advanced optimization algorithms and methodologies and enables engineers to find optimal solutions for complex engineering problems. Its workflow-based environment and multi-objective optimization algorithms are used for streamlining the engineering design process and obtaining improved results.
Casting is a manufacturing process in which a liquid material is usually poured into a mold, which contains a hollow cavity of the desired shape, and then allowed to solidify. The solidified part is also known as a casting, which is ejected or broken out of the mold to complete the process. Casting materials are usually metals or various time setting materials that cure after mixing two or more components together; examples are epoxy, concrete, plaster and clay. Casting is most often used for making complex shapes that would be otherwise difficult or uneconomical to make by other methods. Heavy equipment like machine tool beds, ships' propellers, etc. can be cast easily in the required size, rather than fabricating by joining several small pieces. Casting is a 7,000-year-old process. The oldest surviving casting is a copper frog from 3200 BC.
VisualFEA is a finite element analysis software program for Microsoft Windows and Mac OS X. It is developed and distributed by Intuition Software, Inc. of South Korea, and used chiefly for structural and geotechnical analysis. Its strongest point is its intuitive, user-friendly design based on graphical pre- and postprocessing capabilities. It has educational features for teaching and learning structural mechanics, and finite element analysis through graphical simulation. It is widely used in college-level courses related to structural mechanics and finite element methods.
Simcenter Amesim is a commercial simulation software for the modeling and analysis of multi-domain systems. It is part of systems engineering domain and falls into the mechatronic engineering field.
Predictive engineering analytics (PEA) is a development approach for the manufacturing industry that helps with the design of complex products. It concerns the introduction of new software tools, the integration between those, and a refinement of simulation and testing processes to improve collaboration between analysis teams that handle different applications. This is combined with intelligent reporting and data analytics. The objective is to let simulation drive the design, to predict product behavior rather than to react on issues which may arise, and to install a process that lets design continue after product delivery.
Optimization Systems Associates (OSA) was founded by John Bandler in 1983. OSA produced the first commercial implementation of space mapping optimization to enhance the speed and accuracy of engineering design. OSA’s primary thrust was in computer-aided design (CAD) and simulation and optimization of radio-frequency and microwave circuits and systems. Its products included developments of Bandler's space mapping concept and methodology, which facilitates effective modeling and design optimization of computationally intensive engineering systems.
FEATool Multiphysics is a physics, finite element analysis (FEA), and partial differential equation (PDE) simulation toolbox. FEATool Multiphysics features the ability to model fully coupled heat transfer, fluid dynamics, chemical engineering, structural mechanics, fluid-structure interaction (FSI), electromagnetics, as well as user-defined and custom PDE problems in 1D, 2D (axisymmetry), or 3D, all within a graphical user interface (GUI) or optionally as script files. FEATool has been employed and used in academic research, teaching, and industrial engineering simulation contexts.
Titanium foams exhibit high specific strength, high energy absorption, excellent corrosion resistance and biocompatibility. These materials are ideally suited for applications within the aerospace industry. An inherent resistance to corrosion allows the foam to be a desirable candidate for various filtering applications. Further, titanium's physiological inertness makes its porous form a promising candidate for biomedical implantation devices. The largest advantage in fabricating titanium foams is that the mechanical and functional properties can be adjusted through manufacturing manipulations that vary porosity and cell morphology. The high appeal of titanium foams is directly correlated to a multi-industry demand for advancement in this technology.
Probabilistic numerics is an active field of study at the intersection of applied mathematics, statistics, and machine learning centering on the concept of uncertainty in computation. In probabilistic numerics, tasks in numerical analysis such as finding numerical solutions for integration, linear algebra, optimization and simulation and differential equations are seen as problems of statistical, probabilistic, or Bayesian inference.
{{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) CS1 maint: multiple names: authors list (link){{cite book}}: CS1 maint: multiple names: authors list (link){{cite book}}: CS1 maint: multiple names: authors list (link){{cite book}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) CS1 maint: multiple names: authors list (link){{cite journal}}: CS1 maint: DOI inactive as of November 2024 (link) CS1 maint: multiple names: authors list (link)