Quantum computing is the exploitation of collective properties of quantum states, such as superposition and entanglement, to perform computation. The devices that perform quantum computations are known as quantum computers. They are believed to be able to solve certain computational problems, such as integer factorization, substantially faster than classical computers. The study of quantum computing is a subfield of quantum information science. It is likely to expand in the next few years as the field shifts toward real-world use in pharmaceutical, data security and other applications.
Shor's algorithm is a polynomial-time quantum computer algorithm for integer factorization. Informally, it solves the following problem: Given an integer , find its prime factors. It was invented in 1994 by the American mathematician Peter Shor.
This is a timeline of quantum computing.
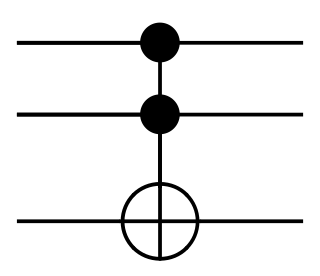
In logic circuits, the Toffoli gate, invented by Tommaso Toffoli, is a universal reversible logic gate, which means that any classical reversible circuit can be constructed from Toffoli gates. It is also known as the "controlled-controlled-not" gate, which describes its action. It has 3-bit inputs and outputs; if the first two bits are both set to 1, it inverts the third bit, otherwise all bits stay the same.
In quantum computing, a quantum algorithm is an algorithm which runs on a realistic model of quantum computation, the most commonly used model being the quantum circuit model of computation. A classical algorithm is a finite sequence of instructions, or a step-by-step procedure for solving a problem, where each step or instruction can be performed on a classical computer. Similarly, a quantum algorithm is a step-by-step procedure, where each of the steps can be performed on a quantum computer. Although all classical algorithms can also be performed on a quantum computer, the term quantum algorithm is usually used for those algorithms which seem inherently quantum, or use some essential feature of quantum computation such as quantum superposition or quantum entanglement.

A trapped ion quantum computer is one proposed approach to a large-scale quantum computer. Ions, or charged atomic particles, can be confined and suspended in free space using electromagnetic fields. Qubits are stored in stable electronic states of each ion, and quantum information can be transferred through the collective quantized motion of the ions in a shared trap. Lasers are applied to induce coupling between the qubit states or coupling between the internal qubit states and the external motional states.

Nuclear magnetic resonance quantum computing (NMRQC) is one of the several proposed approaches for constructing a quantum computer, that uses the spin states of nuclei within molecules as qubits. The quantum states are probed through the nuclear magnetic resonances, allowing the system to be implemented as a variation of nuclear magnetic resonance spectroscopy. NMR differs from other implementations of quantum computers in that it uses an ensemble of systems, in this case molecules, rather than a single pure state.
Quantum annealing (QA) is a metaheuristic for finding the global minimum of a given objective function over a given set of candidate solutions, by a process using quantum fluctuations. Quantum annealing is used mainly for problems where the search space is discrete with many local minima; such as finding the ground state of a spin glass or the traveling salesman problem. Quantum annealing was first proposed in 1988 by B. Apolloni, N. Cesa Bianchi and D. De Falco. It was formulated in its present form by T. Kadowaki and H. Nishimori (ja) in "Quantum annealing in the transverse Ising model" though a proposal in a different form had been made by A. B. Finnila, M. A. Gomez, C. Sebenik and J. D. Doll, in "Quantum annealing: A new method for minimizing multidimensional functions".

D-Wave Systems Inc. is a Canadian quantum computing company, based in Burnaby, British Columbia, Canada. D-Wave was the world's first company to sell computers to exploit quantum effects in their operation. D-Wave's early customers include Lockheed Martin, University of Southern California, Google/NASA and Los Alamos National Lab.
Integer factorization is the process of determining which prime numbers divide a given positive integer. Doing this quickly has applications in cryptography. The difficulty depends on both the size and form of the number and its prime factors; it is currently very difficult to factorize large semiprimes.
The one-way or measurement-based quantum computer (MBQC) is a method of quantum computing that first prepares an entangled resource state, usually a cluster state or graph state, then performs single qubit measurements on it. It is "one-way" because the resource state is destroyed by the measurements.
Adiabatic quantum computation (AQC) is a form of quantum computing which relies on the adiabatic theorem to do calculations and is closely related to quantum annealing.
In quantum computing, the (quantum) threshold theorem states that a quantum computer with a physical error rate below a certain threshold can, through application of quantum error correction schemes, suppress the logical error rate to arbitrarily low levels. This shows that quantum computers can be made fault-tolerant, as an analogue to von Neumann's threshold theorem for classical computation. This result was proven by the groups of Aharanov and Ben-Or; Knill, Laflamme, and Zurek; and Kitaev independently. These results built off a paper of Shor, which proved a weaker version of the threshold theorem.
Daniel Amihud Lidar is the holder of the Viterbi Professorship of Engineering at the University of Southern California, where he is a Professor of Electrical Engineering, Chemistry, Physics & Astronomy. He is the Director and co-founder of the USC Center for Quantum Information Science & Technology (CQIST) as well as Scientific Director of the USC-Lockheed Martin Quantum Computing Center, notable for his research on control of quantum systems and quantum information processing.
Linear Optical Quantum Computing or Linear Optics Quantum Computation (LOQC) is a paradigm of quantum computation, allowing universal quantum computation. LOQC uses photons as information carriers, mainly uses linear optical elements, or optical instruments to process quantum information, and uses photon detectors and quantum memories to detect and store quantum information.
Edward Farhi is a Principal Scientist at Google working on quantum computation. In 2018 he retired from his position as the Cecil and Ida Green Professor of Physics at the Massachusetts Institute of Technology. He was the Director of the Center for Theoretical Physics at MIT from 2004 until 2016. He made contributions to particle physics, general relativity and astroparticle physics before turning to his current interest, quantum computation. For a recent interview see here.
In quantum computing, quantum supremacy or quantum advantage is the goal of demonstrating that a programmable quantum device can solve a problem that no classical computer can solve in any feasible amount of time. Conceptually, quantum supremacy involves both the engineering task of building a powerful quantum computer and the computational-complexity-theoretic task of finding a problem that can be solved by that quantum computer and has a superpolynomial speedup over the best known or possible classical algorithm for that task. The term was coined by John Preskill in 2012, but the concept of a quantum computational advantage, specifically for simulating quantum systems, dates back to Yuri Manin's (1980) and Richard Feynman's (1981) proposals of quantum computing. Examples of proposals to demonstrate quantum supremacy include the boson sampling proposal of Aaronson and Arkhipov, D-Wave's specialized frustrated cluster loop problems, and sampling the output of random quantum circuits.
Continuous-variable quantum information is the area of quantum information science that makes use of physical observables, like the strength of an electromagnetic field, whose numerical values belong to continuous intervals. One primary application is quantum computing. In a sense, continuous-variable quantum computation is "analog", while quantum computation using qubits is "digital." In more technical terms, the former makes use of Hilbert spaces that are infinite-dimensional, while the Hilbert spaces for systems comprising collections of qubits are finite-dimensional. One motivation for studying continuous-variable quantum computation is to understand what resources are necessary to make quantum computers more powerful than classical ones.
In quantum computing, a qubit is a unit of information analogous to a bit in classical computing, but it is affected by quantum mechanical properties such as superposition and entanglement which allow qubits to be in some ways more powerful than classical bits for some tasks. Qubits are used in quantum circuits and quantum algorithms composed of quantum logic gates to solve computational problems, where they are used for input/output and intermediate computations.




