
A wavelet is a wave-like oscillation with an amplitude that begins at zero, increases or decreases, and then returns to zero one or more times. Wavelets are termed a "brief oscillation". A taxonomy of wavelets has been established, based on the number and direction of its pulses. Wavelets are imbued with specific properties that make them useful for signal processing.
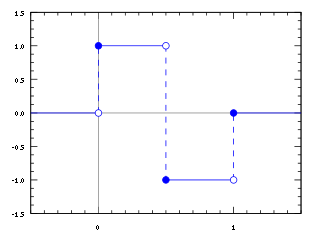
In mathematics, the Haar wavelet is a sequence of rescaled "square-shaped" functions which together form a wavelet family or basis. Wavelet analysis is similar to Fourier analysis in that it allows a target function over an interval to be represented in terms of an orthonormal basis. The Haar sequence is now recognised as the first known wavelet basis and extensively used as a teaching example.
A wave function in quantum physics is a mathematical description of the quantum state of an isolated quantum system. The wave function is a complex-valued probability amplitude, and the probabilities for the possible results of measurements made on the system can be derived from it. The most common symbols for a wave function are the Greek letters ψ and Ψ.
In mathematics, the Hermite polynomials are a classical orthogonal polynomial sequence.
Pseudo-spectral methods, also known as discrete variable representation (DVR) methods, are a class of numerical methods used in applied mathematics and scientific computing for the solution of partial differential equations. They are closely related to spectral methods, but complement the basis by an additional pseudo-spectral basis, which allows representation of functions on a quadrature grid. This simplifies the evaluation of certain operators, and can considerably speed up the calculation when using fast algorithms such as the fast Fourier transform.

The Daubechies wavelets, based on the work of Ingrid Daubechies, are a family of orthogonal wavelets defining a discrete wavelet transform and characterized by a maximal number of vanishing moments for some given support. With each wavelet type of this class, there is a scaling function which generates an orthogonal multiresolution analysis.
The fast wavelet transform is a mathematical algorithm designed to turn a waveform or signal in the time domain into a sequence of coefficients based on an orthogonal basis of small finite waves, or wavelets. The transform can be easily extended to multidimensional signals, such as images, where the time domain is replaced with the space domain. This algorithm was introduced in 1989 by Stéphane Mallat.

Coiflets are discrete wavelets designed by Ingrid Daubechies, at the request of Ronald Coifman, to have scaling functions with vanishing moments. The wavelet is near symmetric, their wavelet functions have vanishing moments and scaling functions , and has been used in many applications using Calderón–Zygmund operators.
In mathematics, Fredholm's theorems are a set of celebrated results of Ivar Fredholm in the Fredholm theory of integral equations. There are several closely related theorems, which may be stated in terms of integral equations, in terms of linear algebra, or in terms of the Fredholm operator on Banach spaces.
A multiresolution analysis (MRA) or multiscale approximation (MSA) is the design method of most of the practically relevant discrete wavelet transforms (DWT) and the justification for the algorithm of the fast wavelet transform (FWT). It was introduced in this context in 1988/89 by Stephane Mallat and Yves Meyer and has predecessors in the microlocal analysis in the theory of differential equations and the pyramid methods of image processing as introduced in 1981/83 by Peter J. Burt, Edward H. Adelson and James L. Crowley.
In mathematics, a dual wavelet is the dual to a wavelet. In general, the wavelet series generated by a square-integrable function will have a dual series, in the sense of the Riesz representation theorem. However, the dual series is not itself in general representable by a square-integrable function.
In control theory, a distributed-parameter system is a system whose state space is infinite-dimensional. Such systems are therefore also known as infinite-dimensional systems. Typical examples are systems described by partial differential equations or by delay differential equations.
A Biorthogonal wavelet is a wavelet where the associated wavelet transform is invertible but not necessarily orthogonal. Designing biorthogonal wavelets allows more degrees of freedom than orthogonal wavelets. One additional degree of freedom is the possibility to construct symmetric wavelet functions.
In mathematics, in the area of wavelet analysis, a refinable function is a function which fulfils some kind of self-similarity. A function is called refinable with respect to the mask if
In the mathematical topic of wavelet theory, the cascade algorithm is a numerical method for calculating function values of the basic scaling and wavelet functions of a discrete wavelet transform using an iterative algorithm. It starts from values on a coarse sequence of sampling points and produces values for successively more densely spaced sequences of sampling points. Because it applies the same operation over and over to the output of the previous application, it is known as the cascade algorithm.
In mathematics the division polynomials provide a way to calculate multiples of points on elliptic curves and to study the fields generated by torsion points. They play a central role in the study of counting points on elliptic curves in Schoof's algorithm.
Overcompleteness is a concept from linear algebra that is widely used in mathematics, computer science, engineering, and statistics. It was introduced by R. J. Duffin and A. C. Schaeffer in 1952.
In mathematics, a biorthogonal polynomial is a polynomial that is orthogonal to several different measures. Biorthogonal polynomials are a generalization of orthogonal polynomials and share many of their properties. There are two different concepts of biorthogonal polynomials in the literature: Iserles & Nørsett (1988) introduced the concept of polynomials biorthogonal with respect to a sequence of measures, while Szegő introduced the concept of two sequences of polynomials that are biorthogonal with respect to each other.
In applied mathematical analysis, shearlets are a multiscale framework which allows efficient encoding of anisotropic features in multivariate problem classes. Originally, shearlets were introduced in 2006 for the analysis and sparse approximation of functions . They are a natural extension of wavelets, to accommodate the fact that multivariate functions are typically governed by anisotropic features such as edges in images, since wavelets, as isotropic objects, are not capable of capturing such phenomena.
In applied mathematics, biorthogonal nearly coiflet bases are wavelet bases proposed by Lowell L. Winger. The wavelet is based on biorthogonal coiflet wavelet bases, but sacrifices its regularity to increase the filter's bandwidth, which might lead to better image compression performance.























