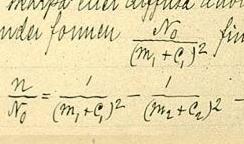Sharp series

Last updated

The sharp series is a series of spectral lines in the atomic emission spectrum caused when electrons descend from higher-energy s orbitals of an atom to the lowest available p orbital. The spectral lines include some in the visible light, and they extend into the ultraviolet. The lines get closer and closer together as the frequency increases never exceeding the series limit. The sharp series was important in the development of the understanding of electron shells and subshells in atoms. The sharp series has given the letter s to the s atomic orbital or subshell.

Contents

The sharp series has a limit given by

The series is caused by transitions to the lowest P state from higher energy S orbitals. One terminology to identify the lines is: 1P-mS [1] But note that 1P just means the lowest P state in an atom and that the modern designation would start at 2P, and is larger for higher atomic numbered atoms.

The terms can have different designations, mS for single line systems, mσ for doublets and ms for triplets. [2]

Since the P state is not the lowest energy level for the alkali atom (the S is) the sharp series will not show up as absorption in a cool gas, however it shows up as emission lines. The Rydberg correction is largest for the S term as the electron penetrates the inner core of electrons more.

The limit for the series corresponds to electron emission, where the electron has so much energy it escapes the atom. Even though the series is called sharp, the lines may not be sharp. [3]

In alkali metals the P terms are split and . This causes the spectral lines to be doublets, with a constant spacing between the two parts of the double line.

[4]

Names

The sharp series used to be called the second subordinate series, with the diffuse series being the first subordinate, both being subordinate to the principal series. [2]

Laws for alkali metals

The sharp series limit is the same as the diffuse series limit. In the late 1800s these two were termed supplementary series.

In 1896 Arthur Schuster stated his law: "If we subtract the frequency of the fundamental vibration from the convergence frequency of the principal series, we obtain the convergence frequency of the supplementary series". [5] But in the next issue of the journal he realised that Rydberg had published the idea a few months earlier. [6]

Rydberg Schuster Law: Using wave numbers, the difference between the sharp and diffuse series limits and principle series limit is the same as the first transition in the principal series.

Runge's Law: Using wave numbers the difference between the sharp series limit and fundamental series limit is the same as the first transition in the diffuse series.

Sodium

Grotrian diagram for sodium. Sharp series is due to 3p-mS transitions shown here in purple. Energy levels of sodium atom.png
Grotrian diagram for sodium. Sharp series is due to 3p-mS transitions shown here in purple.

The sharp series has wave numbers given by:

The sodium diffuse series has wave numbers given by:

when n tends to infinity the diffuse and sharp series end up with the same limit. [8]

sodium sharp series [9]
transitionwavelength 1 Åwavelength 2 Å
3P-4S11403.811381.5
3P-5S6160.756154.23
3P-6S5158.845153.40
3P-7S4751.824747.94
3P-8S4545.194541.63
3P-9S4423.354419.89
3P-10S4344.744341.49
3P-11S4291.014287.84
3P-12S4252.524249.41
3P-13S4223.24220.2
3P-14S4201.04198.0

Potassium

potassium sharp series [10]
transitionwavelength 1 Åwavelength 2 Å
4P-5S12522.112432.2
4P-6S6933.86911.1
4P-7S5801.85782.4
4P-8S5339.85323.4
4P-9S5099.25084.3
4P-10S4956.14942.0
4P-11S4863.64850.0
4P-12S4800.24786.9
4P-13S4754.64741.6

Alkaline earths

A sharp series of triplet lines is designated by series letter s and formula 1p-ms. The sharp series of singlet lines has series letter S and formula 1P-mS. [3]

Calcium

Calcium has a sharp series of triplets and a sharp series of singlets. [11]

Magnesium

Magnesium has a sharp series of triplets and a sharp series of singlets. [3]

History

At Cambridge University George Liveing and James Dewar set out to systematically measure spectra of elements from groups I, II and III in visible light and ultraviolet that would transmit through air. They noticed that lines for sodium were alternating sharp and diffuse. They were the first to use the term "sharp" for the lines. [12] They classified alkali metal spectral lines into sharp and diffuse categories. In 1890 the lines that also appeared in the absorption spectrum were termed the principal series. Rydberg continued the use of sharp and diffuse for the other lines, [13] whereas Kayser and Runge preferred to use the term second subordinate series for the sharp series. [14]

Arno Bergmann found a fourth series in infrared in 1907, and this became known as Bergmann Series or fundamental series. [14]

In 1896 Edward C. Pickering found a new series of lines in the spectrum of ζ Puppis. This was believed to be the sharp series of hydrogen. In 1915 proof was given that it was actually ionised helium - helium II. [15] [16]

Heinrich Kayser, Carl Runge and Johannes Rydberg found mathematical relations between the wave numbers of emission lines of the alkali metals. [17]

Friedrich Hund introduced the s, p, d, f notation for subshells in atoms. [17] [18] Others followed this use in the 1930s and the terminology has remained to this day.

Related Research Articles

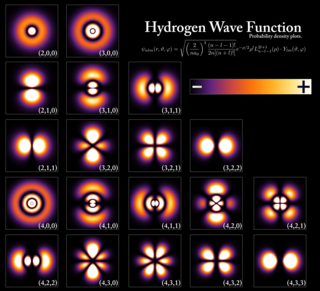
<span class="mw-page-title-main">Atomic orbital</span> Function describing an electron in an atom

In atomic theory and quantum mechanics, an atomic orbital is a function describing the location and wave-like behavior of an electron in an atom. This function can be used to calculate the probability of finding any electron of an atom in any specific region around the atom's nucleus. The term atomic orbital may also refer to the physical region or space where the electron can be calculated to be present, as predicted by the particular mathematical form of the orbital.

<span class="mw-page-title-main">Bohr model</span> Atomic model introduced by Niels Bohr in 1913

In atomic physics, the Bohr model or Rutherford–Bohr model of the atom, presented by Niels Bohr and Ernest Rutherford in 1913, consists of a small, dense nucleus surrounded by orbiting electrons. It is analogous to the structure of the Solar System, but with attraction provided by electrostatic force rather than gravity, and with the electron energies quantized.

<span class="mw-page-title-main">Energy level</span> Different states of quantum systems

A quantum mechanical system or particle that is bound—that is, confined spatially—can only take on certain discrete values of energy, called energy levels. This contrasts with classical particles, which can have any amount of energy. The term is commonly used for the energy levels of the electrons in atoms, ions, or molecules, which are bound by the electric field of the nucleus, but can also refer to energy levels of nuclei or vibrational or rotational energy levels in molecules. The energy spectrum of a system with such discrete energy levels is said to be quantized.

<span class="mw-page-title-main">Ionization energy</span> Energy needed to remove an electron

In physics and chemistry, ionization energy (IE) (American English spelling), ionisation energy (British English spelling) is the minimum energy required to remove the most loosely bound electron of an isolated gaseous atom, positive ion, or molecule. The first ionization energy is quantitatively expressed as

<span class="mw-page-title-main">Electron configuration</span> Mode of arrangement of electrons in different shells of an atom

In atomic physics and quantum chemistry, the electron configuration is the distribution of electrons of an atom or molecule in atomic or molecular orbitals. For example, the electron configuration of the neon atom is 1s2 2s2 2p6, meaning that the 1s, 2s and 2p subshells are occupied by 2, 2 and 6 electrons respectively.

In spectroscopy, the Rydberg constant, symbol for heavy atoms or for hydrogen, named after the Swedish physicist Johannes Rydberg, is a physical constant relating to the electromagnetic spectra of an atom. The constant first arose as an empirical fitting parameter in the Rydberg formula for the hydrogen spectral series, but Niels Bohr later showed that its value could be calculated from more fundamental constants according to his model of the atom.

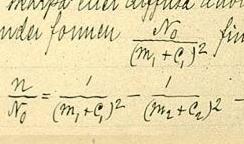
<span class="mw-page-title-main">Rydberg formula</span> Formula for spectral line wavelengths in alkali metals

In atomic physics, the Rydberg formula calculates the wavelengths of a spectral line in many chemical elements. The formula was primarily presented as a generalization of the Balmer series for all atomic electron transitions of hydrogen. It was first empirically stated in 1888 by the Swedish physicist Johannes Rydberg, then theoretically by Niels Bohr in 1913, who used a primitive form of quantum mechanics. The formula directly generalizes the equations used to calculate the wavelengths of the hydrogen spectral series.

In physics and chemistry, the Lyman series is a hydrogen spectral series of transitions and resulting ultraviolet emission lines of the hydrogen atom as an electron goes from n ≥ 2 to n = 1, the lowest energy level of the electron. The transitions are named sequentially by Greek letters: from n = 2 to n = 1 is called Lyman-alpha, 3 to 1 is Lyman-beta, 4 to 1 is Lyman-gamma, and so on. The series is named after its discoverer, Theodore Lyman. The greater the difference in the principal quantum numbers, the higher the energy of the electromagnetic emission.

Relativistic quantum chemistry combines relativistic mechanics with quantum chemistry to calculate elemental properties and structure, especially for the heavier elements of the periodic table. A prominent example is an explanation for the color of gold: due to relativistic effects, it is not silvery like most other metals.

In atomic physics, a term symbol is an abbreviated description of the total spin and orbital angular momentum quantum numbers of the electrons in a multi-electron atom. So while the word symbol suggests otherwise, it represents an actual value of a physical quantity.

<span class="mw-page-title-main">Rydberg atom</span> Excited atomic quantum state with high principal quantum number (n)

A Rydberg atom is an excited atom with one or more electrons that have a very high principal quantum number, n. The higher the value of n, the farther the electron is from the nucleus, on average. Rydberg atoms have a number of peculiar properties including an exaggerated response to electric and magnetic fields, long decay periods and electron wavefunctions that approximate, under some conditions, classical orbits of electrons about the nuclei. The core electrons shield the outer electron from the electric field of the nucleus such that, from a distance, the electric potential looks identical to that experienced by the electron in a hydrogen atom.

<span class="mw-page-title-main">Aufbau principle</span> Principle of atomic physics

The Aufbau principle, also called the Aufbau rule, states that in the ground state of an atom or ion, electrons fill subshells of the lowest available energy, then they fill subshells of higher energy. For example, the 1s subshell is filled before the 2s subshell is occupied. In this way, the electrons of an atom or ion form the most stable electron configuration possible. An example is the configuration 1s2 2s2 2p6 3s2 3p3 for the phosphorus atom, meaning that the 1s subshell has 2 electrons, and so on.

<span class="mw-page-title-main">Hydrogen spectral series</span> Important atomic emission spectra

The emission spectrum of atomic hydrogen has been divided into a number of spectral series, with wavelengths given by the Rydberg formula. These observed spectral lines are due to the electron making transitions between two energy levels in an atom. The classification of the series by the Rydberg formula was important in the development of quantum mechanics. The spectral series are important in astronomical spectroscopy for detecting the presence of hydrogen and calculating red shifts.

The Rydberg–Ritz combination principle is an empirical rule proposed by Walther Ritz in 1908 to describe the relationship of the spectral lines for all atoms, as a generalization of an earlier rule by Johannes Rydberg for the hydrogen atom and the alkali metals. The principle states that the spectral lines of any element include frequencies that are either the sum or the difference of the frequencies of two other lines. Lines of the spectra of elements could be predicted from existing lines. Since the frequency of light is proportional to the wavenumber or reciprocal wavelength, the principle can also be expressed in terms of wavenumbers which are the sum or difference of wavenumbers of two other lines.

The Rydberg states of an atom or molecule are electronically excited states with energies that follow the Rydberg formula as they converge on an ionic state with an ionization energy. Although the Rydberg formula was developed to describe atomic energy levels, it has been used to describe many other systems that have electronic structure roughly similar to atomic hydrogen. In general, at sufficiently high principal quantum numbers, an excited electron-ionic core system will have the general character of a hydrogenic system and the energy levels will follow the Rydberg formula. Rydberg states have energies converging on the energy of the ion. The ionization energy threshold is the energy required to completely liberate an electron from the ionic core of an atom or molecule. In practice, a Rydberg wave packet is created by a laser pulse on a hydrogenic atom and thus populates a superposition of Rydberg states. Modern investigations using pump-probe experiments show molecular pathways – e.g. dissociation of (NO)2 – via these special states.

A spin exchange relaxation-free (SERF) magnetometer is a type of magnetometer developed at Princeton University in the early 2000s. SERF magnetometers measure magnetic fields by using lasers to detect the interaction between alkali metal atoms in a vapor and the magnetic field.

In chemistry and atomic physics, an electron shell may be thought of as an orbit that electrons follow around an atom's nucleus. The closest shell to the nucleus is called the "1 shell" (also called the "K shell"), followed by the "2 shell" (or "L shell"), then the "3 shell" (or "M shell"), and so on farther and farther from the nucleus. The shells correspond to the principal quantum numbers (n = 1, 2, 3, 4 ...) or are labeled alphabetically with the letters used in X-ray notation (K, L, M, ...). A useful guide when understanding electron shells in atoms is to note that each row on the conventional periodic table of elements represents an electron shell.

<span class="mw-page-title-main">Diffuse series</span> Series of lines in atomic spectra

The diffuse series is a series of spectral lines in the atomic emission spectrum caused when electrons jump between the lowest p orbital and d orbitals of an atom. The total orbital angular momentum changes between 1 and 2. The spectral lines include some in the visible light, and may extend into ultraviolet or near infrared. The lines get closer and closer together as the frequency increases never exceeding the series limit. The diffuse series was important in the development of the understanding of electron shells and subshells in atoms. The diffuse series has given the letter d to the d atomic orbital or subshell.

The fundamental series is a set of spectral lines in a set caused by transition between d and f orbitals in atoms.

<span class="mw-page-title-main">Principal series (spectroscopy)</span> Series of lines in atomic spectra

In atomic emission spectroscopy, the principal series is a series of spectral lines caused when electrons move between p orbitals of an atom and the lowest available s orbital. These lines are usually found in the visible and ultraviolet portions of the electromagnetic spectrum. The principal series has given the letter p to the p atomic orbital and subshell.

References

  1. ↑ Fowler, A. (1924). "The Origin of Spectra". Journal of the Royal Astronomical Society of Canada. 18: 373–380. Bibcode:1924JRASC..18..373F.
  2. 1 2 Saunders, F. A. (1915). "Some Recent Discoveries in Spectrum Series". Astrophysical Journal. 41: 323. Bibcode:1915ApJ....41..323S. doi:10.1086/142175.
  3. 1 2 3 Saunders, F. A. (1915). "Some Recent Discoveries in Spectrum Series". Astrophysical Journal. 41: 323–327. Bibcode:1915ApJ....41..323S. doi:10.1086/142175.
  4. ↑ Rydberg, J. R. (1897). "The New Series in the Spectrum of Hydrogen". Astrophysical Journal. 6: 233–236. Bibcode:1897ApJ.....6..233R. doi:10.1086/140393.
  5. ↑ Schuster, Arthur (31 December 1986). "On a New Law Connecting the Periods of Molecular Vibrations". Nature. 55 (1418): 200–201. Bibcode:1896Natur..55..200S. doi: 10.1038/055200a0 .
  6. ↑ Schuster, Arthur (7 January 1987). "On a New Law Connecting the Periods of Molecular Vibrations". Nature. 55 (1419): 223. Bibcode:1897Natur..55..223S. doi:10.1038/055223a0. S2CID   4054702.
  7. 1 2 Atomic, Molecular and Laser Physics. Krishna Prakashan Media. p. 2.59.
  8. ↑ Sala, O.; K. Araki; L. K. Noda (September 1999). "A Procedure to Obtain the Effective Nuclear Charge from the Atomic Spectrum of Sodium" (PDF). Journal of Chemical Education. 76 (9): 1269. Bibcode:1999JChEd..76.1269S. doi:10.1021/ed076p1269.
  9. ↑ Wiese, W.; Smith, M. W.; Miles, B. M. (October 1969). Atomic Transition Probabilities Volume II Sodium Through Calcium A Critical Data Compilation (PDF). Washington: National Bureau of Standards. pp. 39–41. Archived from the original (PDF) on September 24, 2015.
  10. ↑ Wiese, W.; Smith, M. W.; Miles, B. M. (October 1969). Atomic Transition Probabilities Volume II Sodium Through Calcium A Critical Data Compilation (PDF). Washington: National Bureau of Standards. pp. 228–229. Archived from the original (PDF) on September 24, 2015.
  11. ↑ Saunders, F. A. (December 1920). "Revision of the Series in the Spectrum of Calcium". The Astrophysical Journal. 52 (5): 265. Bibcode:1920ApJ....52..265S. doi:10.1086/142578.
  12. ↑ Brand, John Charles Drury (1995-10-01). Lines Of Light: The Sources Of Dispersive Spectroscopy, 1800-1930. CRC Press. pp. 123–. ISBN   9782884491624 . Retrieved 30 December 2013.
  13. ↑ Rydberg, J. R. (April 1890). "XXXIV. On the structure of the line-spectra of the chemical elements". Philosophical Magazine. 5. 29 (179): 331–337. doi:10.1080/14786449008619945.
  14. 1 2 Mehra, Jagdish; Rechenberg, Helmut (2001-01-01). The Historical Development of Quantum Theory. Springer. pp. 165–166. ISBN   9780387951744 . Retrieved 30 December 2013.
  15. ↑ Robotti, Nadia (1983). "The Spectrum of ζ Puppis and the Historical Evolution of Empirical Data". Historical Studies in the Physical Sciences. 14 (1): 123–145. doi:10.2307/27757527. JSTOR   27757527.
  16. ↑ Mebton, Thomas E. (25 March 1915). "On the Origin of the 4686 Series". Philosophical Transactions. Retrieved 30 December 2013.
  17. 1 2 William B. Jensen (2007). "The Origin of the S, p, d, f Orbital Labels". Journal of Chemical Education. 84 (5): 757–758. Bibcode:2007JChEd..84..757J. doi:10.1021/ed084p757.
  18. ↑ Hund, Friedrich (1927). Linienspektren und Periodisches System der Elemente. Struktur der Materie in Einzeldarstellungen. Vol. 4. Springer. pp. 55–56. ISBN   9783709156568.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.