Related Research Articles

Paramagnetism is a form of magnetism whereby some materials are weakly attracted by an externally applied magnetic field, and form internal, induced magnetic fields in the direction of the applied magnetic field. In contrast with this behavior, diamagnetic materials are repelled by magnetic fields and form induced magnetic fields in the direction opposite to that of the applied magnetic field. Paramagnetic materials include most chemical elements and some compounds; they have a relative magnetic permeability slightly greater than 1 and hence are attracted to magnetic fields. The magnetic moment induced by the applied field is linear in the field strength and rather weak. It typically requires a sensitive analytical balance to detect the effect and modern measurements on paramagnetic materials are often conducted with a SQUID magnetometer.

Convection is single or multiphase fluid flow that occurs spontaneously due to the combined effects of material property heterogeneity and body forces on a fluid, most commonly density and gravity. When the cause of the convection is unspecified, convection due to the effects of thermal expansion and buoyancy can be assumed. Convection may also take place in soft solids or mixtures where particles can flow.
In the study of heat transfer, Newton's law of cooling is a physical law which states that the rate of heat loss of a body is directly proportional to the difference in the temperatures between the body and its environment. The law is frequently qualified to include the condition that the temperature difference is small and the nature of heat transfer mechanism remains the same. As such, it is equivalent to a statement that the heat transfer coefficient, which mediates between heat losses and temperature differences, is a constant.
The Biot number (Bi) is a dimensionless quantity used in heat transfer calculations, named for the eighteenth-century French physicist Jean-Baptiste Biot (1774–1862). The Biot number is the ratio of the thermal resistance for conduction inside a body to the resistance for convection at the surface of the body. This ratio indicates whether the temperature inside a body varies significantly in space when the body is heated or cooled over time by a heat flux at its surface.

Heat transfer is a discipline of thermal engineering that concerns the generation, use, conversion, and exchange of thermal energy (heat) between physical systems. Heat transfer is classified into various mechanisms, such as thermal conduction, thermal convection, thermal radiation, and transfer of energy by phase changes. Engineers also consider the transfer of mass of differing chemical species, either cold or hot, to achieve heat transfer. While these mechanisms have distinct characteristics, they often occur simultaneously in the same system.

In physics, the dynamo theory proposes a mechanism by which a celestial body such as Earth or a star generates a magnetic field. The dynamo theory describes the process through which a rotating, convecting, and electrically conducting fluid can maintain a magnetic field over astronomical time scales. A dynamo is thought to be the source of the Earth's magnetic field and the magnetic fields of Mercury and the Jovian planets.

A heat sink is a passive heat exchanger that transfers the heat generated by an electronic or a mechanical device to a fluid medium, often air or a liquid coolant, where it is dissipated away from the device, thereby allowing regulation of the device's temperature. In computers, heat sinks are used to cool CPUs, GPUs, and some chipsets and RAM modules. Heat sinks are used with other high-power semiconductor devices such as power transistors and optoelectronics such as lasers and light-emitting diodes (LEDs), where the heat dissipation ability of the component itself is insufficient to moderate its temperature.

Ferrofluid is a liquid that is attracted to the poles of a magnet. It is a colloidal liquid made of nanoscale ferromagnetic or ferrimagnetic particles suspended in a carrier fluid. Each magnetic particle is thoroughly coated with a surfactant to inhibit clumping. Large ferromagnetic particles can be ripped out of the homogeneous colloidal mixture, forming a separate clump of magnetic dust when exposed to strong magnetic fields. The magnetic attraction of tiny nanoparticles is weak enough that the surfactant's Van der Waals force is sufficient to prevent magnetic clumping or agglomeration. Ferrofluids usually do not retain magnetization in the absence of an externally applied field and thus are often classified as "superparamagnets" rather than ferromagnets.
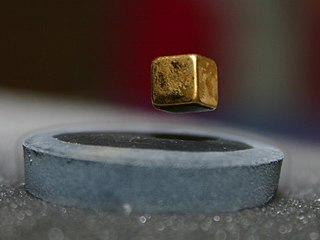
Levitation is the process by which an object is held aloft in a stable position, without mechanical support via any physical contact.
In thermodynamics, the heat transfer coefficient or film coefficient, or film effectiveness, is the proportionality constant between the heat flux and the thermodynamic driving force for the flow of heat. It is used in calculating the heat transfer, typically by convection or phase transition between a fluid and a solid. The heat transfer coefficient has SI units in watts per square meter per kelvin (W/m2K).
Thermal hydraulics is the study of hydraulic flow in thermal fluids. The area can be mainly divided into three parts: thermodynamics, fluid mechanics, and heat transfer, but they are often closely linked to each other. A common example is steam generation in power plants and the associated energy transfer to mechanical motion and the change of states of the water while undergoing this process. Thermal-hydraulic analysis can determine important parameters for reactor design such as plant efficiency and coolability of the system.

The Marangoni effect is the mass transfer along an interface between two phases due to a gradient of the surface tension. In the case of temperature dependence, this phenomenon may be called thermo-capillary convection.

Convection is the transfer of heat from one place to another due to the movement of fluid. Although often discussed as a distinct method of heat transfer, convective heat transfer involves the combined processes of conduction and advection. Convection is usually the dominant form of heat transfer in liquids and gases.

In fluid dynamics, hydrodynamic stability is the field which analyses the stability and the onset of instability of fluid flows. The study of hydrodynamic stability aims to find out if a given flow is stable or unstable, and if so, how these instabilities will cause the development of turbulence. The foundations of hydrodynamic stability, both theoretical and experimental, were laid most notably by Helmholtz, Kelvin, Rayleigh and Reynolds during the nineteenth century. These foundations have given many useful tools to study hydrodynamic stability. These include Reynolds number, the Euler equations, and the Navier–Stokes equations. When studying flow stability it is useful to understand more simplistic systems, e.g. incompressible and inviscid fluids which can then be developed further onto more complex flows. Since the 1980s, more computational methods are being used to model and analyse the more complex flows.
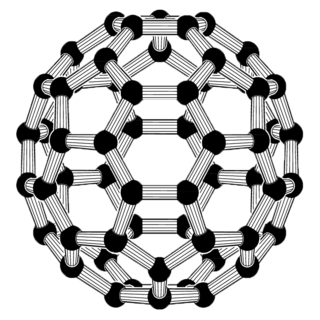
Iron oxide nanoparticles are iron oxide particles with diameters between about 1 and 100 nanometers. The two main forms are composed of magnetite and its oxidized form maghemite. They have attracted extensive interest due to their superparamagnetic properties and their potential applications in many fields including molecular imaging.
In engineering, physics, and chemistry, the study of transport phenomena concerns the exchange of mass, energy, charge, momentum and angular momentum between observed and studied systems. While it draws from fields as diverse as continuum mechanics and thermodynamics, it places a heavy emphasis on the commonalities between the topics covered. Mass, momentum, and heat transport all share a very similar mathematical framework, and the parallels between them are exploited in the study of transport phenomena to draw deep mathematical connections that often provide very useful tools in the analysis of one field that are directly derived from the others.

Geophysical fluid dynamics, in its broadest meaning, refers to the fluid dynamics of naturally occurring flows, such as lava flows, oceans, and planetary atmospheres, on Earth and other planets.
The liquid droplet radiator (LDR) or previously termed liquid droplet stream radiator is a proposed lightweight radiator for the dissipation of waste heat generated by power plants, propulsion or spacecraft systems in space.
Dimensionless numbers have an important role in analyzing the behavior of fluids and their flow as well as in other transport phenomena. They include the Reynolds and the Mach numbers, which describe as ratios the relative magnitude of fluid and physical system characteristics, such as density, viscosity, speed of sound, and flow speed. To compare a real situation with a small-scale model it is necessary to keep the important characteristic numbers the same. Names and formulation of these numbers were standardized in ISO 31-12 and in ISO 80000-11.
References
- ↑ Bednarz, Tomasz; Tagawa, Toshio; Kaneda, Masayuki; Ozoe, Hiroyuki; Szmyd, Janusz S. (2004). "Magnetic and Gravitational Convection of Air with a Coil Inclined Around the X Axis". Numerical Heat Transfer, Part A: Applications. 46 (1): 99–113. Bibcode:2004NHTA...46...99B. doi:10.1080/10407780490457464. S2CID 119902658.
- ↑ Bednarz, Tomasz; Fornalik, Elzbieta; Tagawa, Toshio; Ozoe, Hiroyuki; Szmyd, Janusz S. (2006). "Convection of Paramagnetic Fluid in a Cube Heated and Cooled from Side Walls and Placed below a Superconducting Magnet - Comparison between Experiment and Numerical Computations" (PDF). Thermal Science & Engineering. 14 (4).
- ↑ Bednarz, Tomasz; Patterson, John C.; Lei, Chengwang; Ozoe, Hiroyuki (2009). "Enhancing natural convection in a cube using a strong magnetic field — Experimental heat transfer rate measurements and flow visualization". International Communications in Heat and Mass Transfer. 36 (8): 781–786. doi:10.1016/j.icheatmasstransfer.2009.06.005.
- ↑ Bednarz, Tomasz P.; Lin, Wenxian; Patterson, John C.; Lei, Chengwang; Armfield, Steven W. (2009). "Scaling for unsteady thermo-magnetic convection boundary layer of paramagnetic fluids of Pr>1 in micro-gravity conditions". International Journal of Heat and Fluid Flow. 30 (6): 1157–1170. doi:10.1016/j.ijheatfluidflow.2009.08.003.
- ↑ Phys. Fluids 16, 2228 (2004); doi:10.1063/1.1736691 (9 pages)
- ↑ Kumar, V., Dau, V., Javanbakht, Z., Seager, A., Nguyen, N., and Woodfield, P. (2023). Updated formulation of magnetic body force in ferrofluids. International Journal of Engineering Science, 192, 103929. https://doi.org/10.1016/j.ijengsci.2023.103929