Thomas Royen

Last updated

Thomas Royen
Born
Thomas Royen

(1947-07-06) 6 July 1947 (age 76)
Frankfurt am Main, Germany
CitizenshipGermany
Alma mater Goethe University Frankfurt
University of Freiburg
Technical University Dortmund (PhD)
Known forProof of Gaussian correlation inequality
Scientific career
FieldsMathematics, Statistics
Thesis On Convergence Against Stable Laws (1975)

Thomas Royen (born 6 July 1947) is a retired German professor of statistics who has been affiliated with the University of Applied Sciences Bingen. Royen came to prominence in the spring of 2017 for a relatively simple proof for the Gaussian Correlation Inequality (GCI), a conjecture that originated in the 1950s, which he had published three years earlier without much recognition. [1] A proof of this conjecture, which lies at the intersection of geometry, probability theory and statistics, had eluded top experts for decades. [2]

Contents

Life

Youth, studies and private life

Royen was born in 1947 to Paul Royen, a professor with the institute for inorganic chemistry at the Goethe University Frankfurt and Elisabeth Royen, also a chemist. From 1966 to 1971, he studied mathematics and physics at his father's university and the University of Freiburg. After graduating, he worked as a tutor at the University of Freiburg, before transferring to the Technical University of Dortmund for his doctoral thesis. After attaining his PhD in 1975 with a thesis called Über die Konvergenz gegen stabile Gesetze (On Convergence Against Stable Laws), he worked as a Wissenschaftlicher Assistent at Dortmund University's institute for statistics. Married with children, Royen lives in Schwalbach am Taunus.[ citation needed ]

Career

In 1977, Royen started working as a statistician for the pharmaceutical company Hoechst AG. From 1979 to 1985, he worked at the company's own educational facility teaching mathematics and statistics. Starting in 1985 until becoming an emeritus in 2010, he taught statistics and mathematics at the University of Applied Sciences Bingen in Rhineland-Palatinate. [3]

Royen worked mainly on probability distributions, in particular multivariate chi-squares and gamma distributions, to improve some frequently used statistical test procedures. Nearly half of his circa 30 publications were written when he was aged over sixty. Because he was annoyed over some contradictory reviews and in a few cases also over the incompetence of a referee, he decided in his later years, when his actions had no influence anymore on his further career, to publish his papers on the online platform arXiv.org and sometimes in a less renowned Indian journal to fulfill, at least formally, the condition of a peer review. [4]

Proof of the Gaussian correlation inequality

On 17 July 2014, a few years after his retirement, when brushing his teeth, Royen had a flash of insight: how to use the Laplace transform of the multivariate gamma distribution to achieve a relatively simple proof for the Gaussian correlation inequality, a conjecture on the intersection of geometry, probability theory and statistics, formulated after work by Dunnett and Sobel (1955) and the American statistician Olive Jean Dunn (1958), [5] that had remained unsolved since then. He sent a copy of his proof to Donald Richards, an acquainted American mathematician, who had worked on a proof of the GCI for 30 years. Richards immediately saw the validity of Royen's proof and subsequently helped him to transform the mathematical formulas into LaTeX. When Royen contacted other reputed mathematicians, though, they didn't bother to investigate his proof, because Royen was relatively unknown, and these mathematicians therefore estimated the chance that Royen's proof would be false as very high. [2]

Royen published this proof in an article with the title A simple proof of the Gaussian correlation conjecture extended to multivariate gamma distributions on arXiv [6] and subsequently in the Far East Journal of Theoretical Statistics, [7] a relatively unknown periodical based in Allahabad, India, for which Royen was at the time voluntarily working as a referee himself. Due to this, his proof went at first largely unnoticed by the scientific community, [8] until in late 2015 two Polish mathematicians, Rafał Latała and Dariusz Matlak, wrote a paper in which they reorganized Royen's proof in a way that was intended to be easier to follow. [1] In July 2015, Royen supplemented his proof with a further paper in arXiv Some probability inequalities for multivariate gamma and normal distributions. [9]

A 2017 article by Natalie Wolchover about Royen's proof in Quanta Magazine resulted in greater academic and public recognition for his achievement. [10] [11]

Related Research Articles

Statistics is a field of inquiry that studies the collection, analysis, interpretation, and presentation of data. It is applicable to a wide variety of academic disciplines, from the physical and social sciences to the humanities; it is also used and misused for making informed decisions in all areas of business and government.

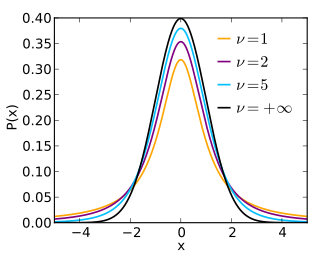
Students <i>t</i>-distribution Probability distribution

In probability and statistics, Student's t-distribution is a continuous probability distribution that generalizes the standard normal distribution. Like the latter, it is symmetric around zero and bell-shaped.

In probability theory, Chebyshev's inequality guarantees that, for a wide class of probability distributions, no more than a certain fraction of values can be more than a certain distance from the mean. Specifically, no more than 1/k2 of the distribution's values can be k or more standard deviations away from the mean. The rule is often called Chebyshev's theorem, about the range of standard deviations around the mean, in statistics. The inequality has great utility because it can be applied to any probability distribution in which the mean and variance are defined. For example, it can be used to prove the weak law of large numbers.

In probability theory and statistics, a Gaussian process is a stochastic process, such that every finite collection of those random variables has a multivariate normal distribution, i.e. every finite linear combination of them is normally distributed. The distribution of a Gaussian process is the joint distribution of all those random variables, and as such, it is a distribution over functions with a continuous domain, e.g. time or space.

Probability is a measure of the likeliness that an event will occur. Probability is used to quantify an attitude of mind towards some proposition whose truth is not certain. The proposition of interest is usually of the form "A specific event will occur." The attitude of mind is of the form "How certain is it that the event will occur?" The certainty that is adopted can be described in terms of a numerical measure, and this number, between 0 and 1 is called the probability. Probability theory is used extensively in statistics, mathematics, science and philosophy to draw conclusions about the likelihood of potential events and the underlying mechanics of complex systems.

In probability theory, the central limit theorem states that, under certain circumstances, the probability distribution of the scaled mean of a random sample converges to a normal distribution as the sample size increases to infinity. Under stronger assumptions, the Berry–Esseen theorem, or Berry–Esseen inequality, gives a more quantitative result, because it also specifies the rate at which this convergence takes place by giving a bound on the maximal error of approximation between the normal distribution and the true distribution of the scaled sample mean. The approximation is measured by the Kolmogorov–Smirnov distance. In the case of independent samples, the convergence rate is n−1/2, where n is the sample size, and the constant is estimated in terms of the third absolute normalized moment.

In probability theory and mathematical physics, a random matrix is a matrix-valued random variable—that is, a matrix in which some or all elements are random variables. Many important properties of physical systems can be represented mathematically as matrix problems. For example, the thermal conductivity of a lattice can be computed from the dynamical matrix of the particle-particle interactions within the lattice.

In mathematics, the Gaussian isoperimetric inequality, proved by Boris Tsirelson and Vladimir Sudakov, and later independently by Christer Borell, states that among all sets of given Gaussian measure in the n-dimensional Euclidean space, half-spaces have the minimal Gaussian boundary measure.

In probability theory and statistical mechanics, the Gaussian free field (GFF) is a Gaussian random field, a central model of random surfaces (random height functions). Sheffield (2007) gives a mathematical survey of the Gaussian free field.

Inequalities are very important in the study of information theory. There are a number of different contexts in which these inequalities appear.

In mathematics, the Fortuin–Kasteleyn–Ginibre (FKG) inequality is a correlation inequality, a fundamental tool in statistical mechanics and probabilistic combinatorics, due to Cees M. Fortuin, Pieter W. Kasteleyn, and Jean Ginibre (1971). Informally, it says that in many random systems, increasing events are positively correlated, while an increasing and a decreasing event are negatively correlated. It was obtained by studying the random cluster model.

This page lists articles related to probability theory. In particular, it lists many articles corresponding to specific probability distributions. Such articles are marked here by a code of the form (X:Y), which refers to number of random variables involved and the type of the distribution. For example (2:DC) indicates a distribution with two random variables, discrete or continuous. Other codes are just abbreviations for topics. The list of codes can be found in the table of contents.

<span class="mw-page-title-main">Gábor J. Székely</span>

Gábor J. Székely is a Hungarian-American statistician/mathematician best known for introducing energy statistics (E-statistics). Examples include: the distance correlation, which is a bona fide dependence measure, equals zero exactly when the variables are independent; the distance skewness, which equals zero exactly when the probability distribution is diagonally symmetric; the E-statistic for normality test; and the E-statistic for clustering.

Boson sampling is a restricted model of non-universal quantum computation introduced by Scott Aaronson and Alex Arkhipov after the original work of Lidror Troyansky and Naftali Tishby, that explored possible usage of boson scattering to evaluate expectation values of permanents of matrices. The model consists of sampling from the probability distribution of identical bosons scattered by a linear interferometer. Although the problem is well defined for any bosonic particles, its photonic version is currently considered as the most promising platform for a scalable implementation of a boson sampling device, which makes it a non-universal approach to linear optical quantum computing. Moreover, while not universal, the boson sampling scheme is strongly believed to implement computing tasks which are hard to implement with classical computers by using far fewer physical resources than a full linear-optical quantum computing setup. This advantage makes it an ideal candidate for demonstrating the power of quantum computation in the near term.

<span class="mw-page-title-main">Olive Jean Dunn</span> American mathematician

Olive Jean Dunn was an American mathematician and statistician, and professor of biostatistics at the University of California Los Angeles (UCLA). She described methods for computing confidence intervals and also codified the Bonferroni correction's application to confidence intervals. She authored the textbook Basic Statistics: A Primer for the Biomedical Sciences in 1977.

<span class="mw-page-title-main">Gaussian correlation inequality</span>

The Gaussian correlation inequality (GCI), formerly known as the Gaussian correlation conjecture (GCC), is a mathematical theorem in the fields of mathematical statistics and convex geometry.

Stephen Mitchell Samuels was a statistician and mathematician, known for his work on the secretary problem and for the Samuels Conjecture involving a Chebyshev-type inequality for sums of independent, non-negative random variables.

References

  1. 1 2 Rafal Latala and Dariusz Matlak, Royen's proof of the Gaussian correlation inequality, arXiv:1512.08776
  2. 1 2 "A Long-Sought Proof, Found and Almost Lost". Quanta Magazine . Natalie Wolchover. 28 March 2017. Retrieved 1 May 2017.
  3. ↑ "Curriculum Vitae Thomas Royen". Forschungsplattform des Landes Rheinland-Pfalz. Landesregierung Rheinland-Pfalz.
  4. ↑ "Der Beweis" (in German). Sibylle Anderl. 7 April 2017.
  5. ↑ Dunn, Olive Jean (March 1959). "Estimation of the medians for dependent variables". The Annals of Mathematical Statistics. 30 (1): 192–197. doi: 10.1214/aoms/1177706374 . JSTOR   2237135.
  6. ↑ Royen, T. (2014). "A simple proof of the Gaussian correlation conjecture extended to multivariate gamma distributions". arXiv: 1408.1028 [math.PR]. Supplemented by Royen, Thomas (2015). "Some probability inequalities for multivariate gamma and normal distributions". arXiv: 1507.00528 [math.PR].
  7. ↑ Thomas Royen: A simple proof of the Gaussian correlation conjecture extended to some multivariate gamma distributions, in: Far East Journal of Theoretical Statistics, Part 48 Nr. 2, Pushpa Publishing House, Allahabad 2014, p.139–145
  8. ↑ In the Quanta magazine article, for instance, Tilmann Gneiting, a statistician at the Heidelberg Institute for Theoretical Studies, just 65 miles from Bingen, said he was shocked to learn in July 2016, two years after the fact, that the GCI had been proved.
  9. ↑ Royen, Thomas (2 July 2015). "Some probability inequalities for multivariate gamma and normal distributions". arXiv: 1507.00528 [math.PR].
  10. ↑ Crew, Bec (4 April 2017). "This German Retiree Solved One of World's Most Complex Maths Problems - And No One Noticed". ScienceAlert . Retrieved 26 December 2020. I know of people who worked on it for 40 years," Donald Richards, a statistician from Pennsylvania State University, told Natalie Wolchover at Quanta Magazine. "I myself worked on it for 30 years.
  11. ↑ Dambeck, Holger (4 April 2017). "67-Jähriger löst altes Statistikproblem" [67-year-old solves old statistics problem]. Der Spiegel (in German). Retrieved 26 December 2020. "Ich kenne Leute, die daran 40 Jahre gearbeitet haben", sagte er dem "Quanta Magazine".
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.