In physics, an anyon is a type of quasiparticle that occurs only in two-dimensional systems, with properties much less restricted than fermions and bosons. In general, the operation of exchanging two identical particles may cause a global phase shift but cannot affect observables. Anyons are generally classified as abelian or non-abelian. Abelian anyons have been detected and play a major role in the fractional quantum Hall effect. Non-abelian anyons have not been definitively detected, although this is an active area of research.

In physics, topological order is a kind of order in the zero-temperature phase of matter. Macroscopically, topological order is defined and described by robust ground state degeneracy and quantized non-Abelian geometric phases of degenerate ground states. Microscopically, topological orders correspond to patterns of long-range quantum entanglement. States with different topological orders cannot change into each other without a phase transition.
A topological quantum computer is a theoretical quantum computer that employs two-dimensional quasiparticles called anyons, whose world lines pass around one another to form braids in a three-dimensional spacetime. These braids form the logic gates that make up the computer. The advantage of a quantum computer based on quantum braids over using trapped quantum particles is that the former is much more stable. Small, cumulative perturbations can cause quantum states to decohere and introduce errors in the computation, but such small perturbations do not change the braids' topological properties. This is like the effort required to cut a string and reattach the ends to form a different braid, as opposed to a ball bumping into a wall. Alexei Kitaev proposed topological quantum computation in 1997. While the elements of a topological quantum computer originate in a purely mathematical realm, experiments in fractional quantum Hall systems indicate these elements may be created in the real world using semiconductors made of gallium arsenide at a temperature of near absolute zero and subjected to strong magnetic fields.

A Majorana fermion, also referred to as a Majorana particle, is a fermion that is its own antiparticle. They were hypothesised by Ettore Majorana in 1937. The term is sometimes used in opposition to a Dirac fermion, which describes fermions that are not their own antiparticles.

In condensed matter physics, a string-net is an extended object whose collective behavior has been proposed as a physical mechanism for topological order by Michael A. Levin and Xiao-Gang Wen. A particular string-net model may involve only closed loops; or networks of oriented, labeled strings obeying branching rules given by some gauge group; or still more general networks.
Quantum dimer models were introduced to model the physics of resonating valence bond (RVB) states in lattice spin systems. The only degrees of freedom retained from the motivating spin systems are the valence bonds, represented as dimers which live on the lattice bonds. In typical dimer models, the dimers do not overlap.
The quantum spin Hall state is a state of matter proposed to exist in special, two-dimensional, semiconductors that have a quantized spin-Hall conductance and a vanishing charge-Hall conductance. The quantum spin Hall state of matter is the cousin of the integer quantum Hall state, and that does not require the application of a large magnetic field. The quantum spin Hall state does not break charge conservation symmetry and spin- conservation symmetry.

Xiao-Gang Wen is a Chinese-American physicist. He is a Cecil and Ida Green Professor of Physics at the Massachusetts Institute of Technology and Distinguished Visiting Research Chair at the Perimeter Institute for Theoretical Physics. His expertise is in condensed matter theory in strongly correlated electronic systems. In Oct. 2016, he was awarded the Oliver E. Buckley Condensed Matter Prize.
Subir Sachdev is Herchel Smith Professor of Physics at Harvard University specializing in condensed matter. He was elected to the U.S. National Academy of Sciences in 2014, and received the Lars Onsager Prize from the American Physical Society and the Dirac Medal from the ICTP in 2018.
A composite fermion is the topological bound state of an electron and an even number of quantized vortices, sometimes visually pictured as the bound state of an electron and, attached, an even number of magnetic flux quanta. Composite fermions were originally envisioned in the context of the fractional quantum Hall effect, but subsequently took on a life of their own, exhibiting many other consequences and phenomena.

A topological insulator is a material that behaves as an insulator in its interior but whose surface contains conducting states, meaning that electrons can only move along the surface of the material. Topological insulators have non-trivial symmetry-protected topological order; however, having a conducting surface is not unique to topological insulators, since ordinary band insulators can also support conductive surface states. What is special about topological insulators is that their surface states are symmetry-protected Dirac fermions by particle number conservation and time-reversal symmetry. In two-dimensional (2D) systems, this ordering is analogous to a conventional electron gas subject to a strong external magnetic field causing electronic excitation gap in the sample bulk and metallic conduction at the boundaries or surfaces.

David R. Nelson is an American physicist, and Arthur K. Solomon Professor of Biophysics, at Harvard University.
The toric code is a topological quantum error correcting code, and an example of a stabilizer code, defined on a two-dimensional spin lattice It is the simplest and most well studied of the quantum double models. It is also the simplest example of topological order—Z2 topological order (first studied in the context of Z2 spin liquid in 1991). The toric code can also be considered to be a Z2 lattice gauge theory in a particular limit. It was introduced by Alexei Kitaev.
The AKLT model is an extension of the one-dimensional quantum Heisenberg spin model. The proposal and exact solution of this model by Affleck, Lieb, Kennedy and Tasaki provided crucial insight into the physics of the spin-1 Heisenberg chain. It has also served as a useful example for such concepts as valence bond solid order, symmetry-protected topological order and matrix product state wavefunctions.
In condensed matter physics, a quantum spin liquid is an unusual phase of matter that can be formed by interacting quantum spins in certain magnetic materials. Quantum spin liquids (QSL) are generally characterized by their long-range quantum entanglement, fractionalized excitations, and absence of ordinary magnetic order.
The Aharonov–Casher effect is a quantum mechanical phenomenon predicted in 1984 by Yakir Aharonov and Aharon Casher, in which a traveling magnetic dipole is affected by an electric field. It is dual to the Aharonov–Bohm effect, in which the quantum phase of a charged particle depends upon which side of a magnetic flux tube it comes through. In the Aharonov–Casher effect, the particle has a magnetic moment and the tubes are charged instead. It was observed in a gravitational neutron interferometer in 1989 and later by fluxon interference of magnetic vortices in Josephson junctions. It has also been seen with electrons and atoms.

A time crystal or space-time crystal is a state of matter that repeats in time, as well as in space. Normal three-dimensional crystals have a repeating pattern in space, but remain unchanged as time passes. Time crystals repeat themselves in time as well, leading the crystal to change from moment to moment.
Symmetry-protected topological (SPT) order is a kind of order in zero-temperature quantum-mechanical states of matter that have a symmetry and a finite energy gap.
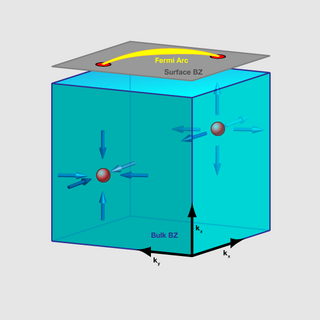
Weyl fermions are massless chiral fermions embodying the mathematical concept of a Weyl spinor. Weyl spinors in turn play an important role in quantum field theory and the Standard Model, where they are a building block for fermions in quantum field theory. Weyl spinors are a solution to the Dirac equation derived by Hermann Weyl, called the Weyl equation. For example, one-half of a charged Dirac fermion of a definite chirality is a Weyl fermion.
The term Dirac matter refers to a class of condensed matter systems which can be effectively described by the Dirac equation. Even though the Dirac equation itself was formulated for fermions, the quasi-particles present within Dirac matter can be of any statistics. As a consequence, Dirac matter can be distinguished in fermionic, bosonic or anyonic Dirac matter. Prominent examples of Dirac matter are Graphene, topological insulators, Dirac semimetals, Weyl semimetals, various high-temperature superconductors with -wave pairing and liquid Helium-3. The effective theory of such systems is classified by a specific choice of the Dirac mass, the Dirac velocity, the Dirac matrices and the space-time curvature. The universal treatment of the class of Dirac matter in terms of an effective theory leads to a common features with respect to the density of states, the heat capacity and impurity scattering.








