Topological polymers

Last updated

Topological polymers may refer to a polymeric molecule that possesses unique spatial features, such as linear, branched, or cyclic architectures. [1] It could also refer to polymer networks that exhibit distinct topologies owing to special crosslinkers. [2] When self-assembling or crosslinking in a certain way, polymeric species with simple topological identity could also demonstrate complicated topological structures in a larger spatial scale. Topological structures, along with the chemical composition, determine the macroscopic physical properties of polymeric materials. [3] [4]

Contents

Definition

Topological polymers, or polymer topology, could refer to a single polymeric chain with topological information or a polymer network with special junctions or connections. When the topology of a polymeric chain or network is investigated, the exact chemical composition is usually neglected, but the way of junctions and connections is more considered. Various topological structures, on one hand, could potentially change the interactions (van der Waals interaction, hydrogen bonding, etc.) between each of the polymer chain. On the other hand, topology also determines the hierarchical structures within a polymer network, from a microscopic level (<1 nm) to a macroscopic level (10-100 nm), which eventually affords polymeric materials with completely different physical properties, [2] such as mechanical property, [5] glass transition temperature, [6] gelation concentration. [7]

Topological polymer classification

In early 1950s, Paul J. Flory was the pioneer who developed theories to explain topology within a polymer network, and the structure-property relationships between the topology and the mechanical property, like elasticity, was initially established afterwards. [3] Later in 1980s, Bertrand Duplantier developed theories to describe any polymer network topologies using statistical mechanics, which could help to derive topology-dependent critical exponents in a polymer network. [8] In early 2000s, Yasuyuki Tezuka and coworkers were the first ones that systematically described a single molecular chain with topological information. [9]

Adapted from Y. Tezuka and coworker's description of a topological polymer chain with more generalized rules, [9] the topology notation rules are to be introduced first, followed by three classical classifications, including linear, branched and cyclic polymer topologies, and they are classified in a table reorganized and redrawn from Y. Tezuka and coworker [9] (Copyright, 2001 by American Chemical Society).

A general polymer chain could be generalized into an undirected graph with nodes (vertices or points) and edges (lines or links) based on graph theory. In a graph theory topology, two sets of nodes are present, termini and junctions. The quantity ‘degree’ represents the number of edges linked to each node, if the degree of a certain node is larger than 3 (including 3), the node is a junction, while the degree of a node is 1, the node is a terminus. There are no nodes with a degree of 2 since they could be generalized into their adjacent nodes.

As for a certain polymer, as long as the topology is fixed, a specific topology notation could be generated using the following rules:

A general polymer chain notation could be expressed as:

  1. Capitalized letter represents the main topology within a polymer, represents linear or branched topology, and Roman numerals are used to represent the number of rings in the polymer chain, represents one cycle, represents two cycles, , , , etc. represents three, four, five cycles and so on.
  2. represents the number of nodes in the graph theory topology, represents the number of termini and represents the number of junctions, and is always true.
  3. If  could represent an exclusive topology, there is no need to add more information to specify the notation. However, if multiple possibilities are present, extra information is needed.

i. For branched topology, a main chain is first selected, and the degree of each junction nodes along the chain should be noted as connected by a hyphen. If there is a side chain on any of the main chain node, should be noted with a bracket following the main chain notation.

ii. For monocyclic topology, the outward branch should be firstly identified with the number of branches at each of the junctions as connected by a hyphen. Then the topology of each branch should be identified using the rule in i as using a bracket following the notations.

iii. For multicyclic topology, superscript letter (, , and so on) is used to describe internal connections within an existing ring.

Linear

Linear topology is a special topological structure that exclusively has two nodes as the termini without any junction nodes. High-density polyethylene (HDPE) could be regarded as a linear polymer chain with very small amount of branching, the linear topology has been listed below: [9] Linear chains capable of forming intra-chain interactions can fold into a wide range of circuit topologies. Examples include biopolymers such as proteins and nucleic acids.

Linear topology table.png

Branched

When side chains are introduced into a linear polymer chain, a branched topology forms. Linear polymers are special types of branched polymers with zero junction nodes, but they are cataloged into two classifications to distinguish their special macroscopic properties. [2] Branched polymers with the same molecular weight usually demonstrate different physical properties due to that branching could generally decrease the van der Waals interactions between each of the polymer chain. Several well-known branched polymers have been synthesized, such as star-shape polymer, comb polymer and dendrimer. Selected branched topologies have been listed below: [9]

Branched topology table.png

Cyclic

Cyclic structures are of interest topologically because there are no termini in this topology and the physical property could be dramatically different as a result of the restriction of the termini.

Monocyclic

Monocyclic topology is a topological structure with only one cycle in the polymer chain, and it could be coupled with outward branching structures. Selected monocyclic topologies have been listed below: [9]

Monocyclic topology table .png

Bicyclic

Bicyclic topology refers to a structure that two cycles connected internally or externally are present in a polymer chain. Selected bicyclic topologies are listed below: [9]

Bicyclic topology table.png

Polycyclic

Similar to monocyclic and bicyclic topologies, polycyclic topologies possess more cycles in a polymer chain and are more synthetically challenging. Selected polycyclic (tricyclic) topologies are listed below: [9]

Polycyclic topology table.png

Polymer network topology

Unlike single chain polymeric species, polymer network topology is more complicated as a result of the amorphous feature so that a simple notation is usually not feasible. To analyze the topology of a network, the crosslinkers, including the branched crosslinker and cyclic crosslinker, are considered.

Branched and cyclic topology polymer network formed by monomers with two or three reactive sites. Topological polymer network.png
Branched and cyclic topology polymer network formed by monomers with two or three reactive sites.

Branched crosslinking

Branched crosslinkers are entities that do not form cyclic topologies, which could be simply understood by branched topological polymer chain above. The ‘degree’ of branched demonstrates the theoretical number of polymer strands at the junctions of the crosslinker, also known as branch functionality (f). [2]

Combining monomers with different degree of branch functionality could generate various topological network with distinct elastic property. Meanwhile, amphiphilic polymers, such as block copolymers, when forming micelle structures, could also be treated as a branched crosslinker with high degree of branch functionality.

Cyclic crosslinking

Branched crosslinkers should in principle form branched polymer network, but in practice, they could also generate loops and cycles.

Cyclic crosslinkers are more sophisticated and show multiple possibilities. Loops or cycles could form in a smaller scale between two polymer chains or in a larger scale among multiple polymer strands. Besides, bicyclic topology is likely to form if two loops are catenated or linked internally or externally. Special cyclic crosslinking is more attractive within rotaxanes or catenanes since cycles are already present in those molecules.

The characterization of cyclic topologies within a polymer network, compared to branched crosslinker, is relatively harder to perform. Conventional techniques such as rheology and tensile strength analysis are used to offer semiquantitative insights into the polymer topologies. [10] Recently, the development of multiple quantum nuclear magnetic resonance (NMR) [11] and network disassembly spectrometry (NDS) [12] techniques provides quantitative characterizations of loops or cycles in a polymer network. [2]

Topological polymer/network synthesis

Topological polymer single chain

Electrostatic self-assembly and covalent fixation to form a cyclic polymer. Cations and anions self-assemble upon dilution and react with each other upon heat. Electrostatic self-assembly and covalent fixation.png
Electrostatic self-assembly and covalent fixation to form a cyclic polymer. Cations and anions self-assemble upon dilution and react with each other upon heat.

The synthesis of branched polymers (grafted polymer, comb polymer, star-shape polymer and dendrimer) has been well developed using well-known polymerization methodology such as cationic/anionic polymerization. [14]

Unlike branched polymer chain synthesis, the synthesis of cyclic polymer is more challenging. General cyclic species involve the combination between two fragments or among several fragments. Electrostatic self-assembly and covalent fixation is one of the most effective strategies to synthesize cyclic topological polymer. The reaction is driven by the electrostatic interactions between telechelic polytetrahydrofurans with cyclic ammonium salt and pluricaboxylate counterions. Upon dilution, the anions and cations could self-assemble into a cyclic structure, followed by a covalent fixation by heat or other external stimuli to undergo ring-opening reaction and close the chain into a cycle. [13]

Topological polymer network

Polymer networks intrinsically have various spatial features due to their amorphous property within a three-dimensional network. There are generally two ways to introduce spatially unique entities into a polymer network:

Examples

The topology of a polymer chain or a polymer network is crucial in determining the macroscopic properties of a polymeric material, especially mechanical properties like elasticity and physical properties involving phase transitions. To date, several polymers with topological interest have been developed, which have been used for many applications, such as mechanical elastomer, [16] energy, [17] and so on. Below are some of the representative topological polymers or polymer networks.

Interpenetrating polymer

Interpenetration polymers are polymer networks involving two and more polymer strands which are spatially intertwining with each other to form unique spatial topologies. [18]

Dendrimer

Dendrimer is a special branched polymers with a larger fraction of terminal nodes compared to the junction nodes and could be used for applications in drug delivery [19] or catalysis. [20]

Polyrotaxane

Polyrotaxane is a polymer chain or a polymer network with mechanical interlock structures between ring-like molecules and polymer chain, where both the rings and the linear polymer chain could serve as the crosslinker to form a polymer network. [21]

Related Research Articles

<span class="mw-page-title-main">Graph theory</span> Area of discrete mathematics

In mathematics and computer science, graph theory is the study of graphs, which are mathematical structures used to model pairwise relations between objects. A graph in this context is made up of vertices which are connected by edges. A distinction is made between undirected graphs, where edges link two vertices symmetrically, and directed graphs, where edges link two vertices asymmetrically. Graphs are one of the principal objects of study in discrete mathematics.

<span class="mw-page-title-main">Homological algebra</span> Branch of mathematics

Homological algebra is the branch of mathematics that studies homology in a general algebraic setting. It is a relatively young discipline, whose origins can be traced to investigations in combinatorial topology and abstract algebra at the end of the 19th century, chiefly by Henri Poincaré and David Hilbert.

In mathematics, the term homology, originally introduced in algebraic topology, has three primary, closely-related usages. The most direct usage of the term is to take the homology of a chain complex, resulting in a sequence of abelian groups called homology groups. This operation, in turn, allows one to associate various named homologies or homology theories to various other types of mathematical objects. Lastly, since there are many homology theories for topological spaces that produce the same answer, one also often speaks of the homology of a topological space. There is also a related notion of the cohomology of a cochain complex, giving rise to various cohomology theories, in addition to the notion of the cohomology of a topological space.

<span class="mw-page-title-main">Scale-free network</span> Network whose degree distribution follows a power law

A scale-free network is a network whose degree distribution follows a power law, at least asymptotically. That is, the fraction P(k) of nodes in the network having k connections to other nodes goes for large values of k as

Noncommutative geometry (NCG) is a branch of mathematics concerned with a geometric approach to noncommutative algebras, and with the construction of spaces that are locally presented by noncommutative algebras of functions, possibly in some generalized sense. A noncommutative algebra is an associative algebra in which the multiplication is not commutative, that is, for which does not always equal ; or more generally an algebraic structure in which one of the principal binary operations is not commutative; one also allows additional structures, e.g. topology or norm, to be possibly carried by the noncommutative algebra of functions.

<span class="mw-page-title-main">Cyclic order</span> Alternative mathematical ordering

In mathematics, a cyclic order is a way to arrange a set of objects in a circle.[nb] Unlike most structures in order theory, a cyclic order is not modeled as a binary relation, such as "a < b". One does not say that east is "more clockwise" than west. Instead, a cyclic order is defined as a ternary relation [a, b, c], meaning "after a, one reaches b before c". For example, [June, October, February], but not [June, February, October], cf. picture. A ternary relation is called a cyclic order if it is cyclic, asymmetric, transitive, and connected. Dropping the "connected" requirement results in a partial cyclic order.

A graphical model or probabilistic graphical model (PGM) or structured probabilistic model is a probabilistic model for which a graph expresses the conditional dependence structure between random variables. They are commonly used in probability theory, statistics—particularly Bayesian statistics—and machine learning.

In computer science, a topological sort or topological ordering of a directed graph is a linear ordering of its vertices such that for every directed edge (u,v) from vertex u to vertex v, u comes before v in the ordering. For instance, the vertices of the graph may represent tasks to be performed, and the edges may represent constraints that one task must be performed before another; in this application, a topological ordering is just a valid sequence for the tasks. Precisely, a topological sort is a graph traversal in which each node v is visited only after all its dependencies are visited. A topological ordering is possible if and only if the graph has no directed cycles, that is, if it is a directed acyclic graph (DAG). Any DAG has at least one topological ordering, and algorithms are known for constructing a topological ordering of any DAG in linear time. Topological sorting has many applications, especially in ranking problems such as feedback arc set. Topological sorting is also possible when the DAG has disconnected components.

<span class="mw-page-title-main">Bifurcation theory</span> Study of sudden qualitative behavior changes caused by small parameter changes

Bifurcation theory is the mathematical study of changes in the qualitative or topological structure of a given family of curves, such as the integral curves of a family of vector fields, and the solutions of a family of differential equations. Most commonly applied to the mathematical study of dynamical systems, a bifurcation occurs when a small smooth change made to the parameter values of a system causes a sudden 'qualitative' or topological change in its behavior. Bifurcations occur in both continuous systems and discrete systems.

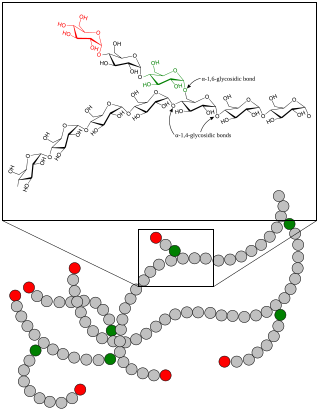
<span class="mw-page-title-main">Branching (polymer chemistry)</span> Attachment of side chains to the backbone chain of a polymer

In polymer chemistry, branching is the regular or irregular attachment of side chains to a polymer's backbone chain. It occurs by the replacement of a substituent on a monomer subunit by another covalently-bonded chain of that polymer; or, in the case of a graft copolymer, by a chain of another type. Branched polymers have more compact and symmetrical molecular conformations, and exhibit intra-heterogeneous dynamical behavior with respect to the unbranched polymers. In crosslinking rubber by vulcanization, short sulfur branches link polyisoprene chains into a multiple-branched thermosetting elastomer. Rubber can also be so completely vulcanized that it becomes a rigid solid, so hard it can be used as the bit in a smoking pipe. Polycarbonate chains can be crosslinked to form the hardest, most impact-resistant thermosetting plastic, used in safety glasses.

The circuit topology of an electronic circuit is the form taken by the network of interconnections of the circuit components. Different specific values or ratings of the components are regarded as being the same topology. Topology is not concerned with the physical layout of components in a circuit, nor with their positions on a circuit diagram; similarly to the mathematical concept of topology, it is only concerned with what connections exist between the components. Numerous physical layouts and circuit diagrams may all amount to the same topology.

<span class="mw-page-title-main">Evolving network</span>

Evolving networks are networks that change as a function of time. They are a natural extension of network science since almost all real world networks evolve over time, either by adding or removing nodes or links over time. Often all of these processes occur simultaneously, such as in social networks where people make and lose friends over time, thereby creating and destroying edges, and some people become part of new social networks or leave their networks, changing the nodes in the network. Evolving network concepts build on established network theory and are now being introduced into studying networks in many diverse fields.

<span class="mw-page-title-main">Polymer architecture</span>

Polymer architecture in polymer science relates to the way branching leads to a deviation from a strictly linear polymer chain. Branching may occur randomly or reactions may be designed so that specific architectures are targeted. It is an important microstructural feature. A polymer's architecture affects many of its physical properties including solution viscosity, melt viscosity, solubility in various solvents, glass transition temperature and the size of individual polymer coils in solution.

Rigidity theory, or topological constraint theory, is a tool for predicting properties of complex networks based on their composition. It was introduced by James Charles Phillips in 1979 and 1981, and refined by Michael Thorpe in 1983. Inspired by the study of the stability of mechanical trusses as pioneered by James Clerk Maxwell, and by the seminal work on glass structure done by William Houlder Zachariasen, this theory reduces complex molecular networks to nodes constrained by rods, thus filtering out microscopic details that ultimately don't affect macroscopic properties. An equivalent theory was developed by P. K. Gupta and A. R. Cooper in 1990, where rather than nodes representing atoms, they represented unit polytopes. An example of this would be the SiO tetrahedra in pure glassy silica. This style of analysis has applications in biology and chemistry, such as understanding adaptability in protein-protein interaction networks. Rigidity theory applied to the molecular networks arising from phenotypical expression of certain diseases may provide insights regarding their structure and function.

<span class="mw-page-title-main">Circuit topology</span> Graph topology applied to electrical and communications circuits, or biomolecules

The circuit topology of a folded linear polymer refers to the arrangement of its intra-molecular contacts. Examples of linear polymers with intra-molecular contacts are nucleic acids and proteins. Proteins fold via the formation of contacts of various natures, including hydrogen bonds, disulfide bonds, and beta-beta interactions. RNA molecules fold by forming hydrogen bonds between nucleotides, forming nested or non-nested structures. Contacts in the genome are established via protein bridges including CTCF and cohesins and are measured by technologies including Hi-C. Circuit topology categorises the topological arrangement of these physical contacts, that are referred to as hard contacts. Furthermore, chains can fold via knotting. Circuit topology uses a similar language to categorise both "soft" and "hard" contacts, and provides a full description of a folded linear chain. In this framework, a "circuit" refers to a segment of the chain where each contact site within the segment forms connections with other contact sites within the same segment, and thus is not left unpaired. A folded chain can thus be studied based on its constituting circuits.

In organosulfur chemistry, the thiol-ene reaction is an organic reaction between a thiol and an alkene to form a thioether. This reaction was first reported in 1905, but it gained prominence in the late 1990s and early 2000s for its feasibility and wide range of applications. This reaction is accepted as a click chemistry reaction given the reactions' high yield, stereoselectivity, high rate, and thermodynamic driving force.

<span class="mw-page-title-main">Knotted polymers</span>

Single Chain Cyclized/Knotted Polymers are a new class of polymer architecture with a general structure consisting of multiple intramolecular cyclization units within a single polymer chain. Such a structure was synthesized via the controlled polymerization of multivinyl monomers, which was first reported in Dr. Wenxin Wang's research lab. These multiple intramolecular cyclized/knotted units mimic the characteristics of complex knots found in proteins and DNA which provide some elasticity to these structures. Of note, 85% of elasticity in natural rubber is due to knot-like structures within its molecular chain.
An intramolecular cyclization reaction is where the growing polymer chain reacts with a vinyl functional group on its own chain, rather than with another growing chain in the reaction system. In this way the growing polymer chain covalently links to itself in a fashion similar to that of a knot in a piece of string. As such, single chain cyclized/knotted polymers consist of many of these links, as opposed to other polymer architectures including branched and crosslinked polymers that are formed by two or more polymer chains in combination.

In mathematics, particularly in functional analysis and topology, closed graph is a property of functions. A function f : X → Y between topological spaces has a closed graph if its graph is a closed subset of the product space X × Y. A related property is open graph.

The unified scattering function was proposed in 1995 as a universal approach to describe small-angle X-ray, and neutron scattering from disordered systems that display hierarchical structure.

This is a glossary for the terminology in a mathematical field of functional analysis.

References

  1. ↑ Tezuka Y, Oike H (July 2002). "Topological polymer chemistry". Progress in Polymer Science. 27 (6): 1069–1122. doi:10.1016/S0079-6700(02)00009-6.
  2. 1 2 3 4 5 6 7 Gu Y, Zhao J, Johnson JA (March 2019). "A (Macro)Molecular-Level Understanding of Polymer Network Topology". Trends in Chemistry. 1 (3): 318–334. doi: 10.1016/j.trechm.2019.02.017 .
  3. 1 2 Flory PJ (June 1985). "Network topology and the theory of rubber elasticity". British Polymer Journal. 17 (2): 96–102. doi:10.1002/pi.4980170202.
  4. ↑ Wang R, Johnson JA, Olsen BD (March 2017). "Odd–Even Effect of Junction Functionality on the Topology and Elasticity of Polymer Networks". Macromolecules. 50 (6): 2556–2564. Bibcode:2017MaMol..50.2556W. doi:10.1021/acs.macromol.6b01912. ISSN   0024-9297.
  5. ↑ Gu Y, Alt EA, Wang H, Li X, Willard AP, Johnson JA (August 2018). "Photoswitching topology in polymer networks with metal-organic cages as crosslinks". Nature. 560 (7716): 65–69. Bibcode:2018Natur.560...65G. doi:10.1038/s41586-018-0339-0. PMID   30022167.
  6. ↑ Phillips, J.C. (May 1981). "Topology of covalent non-crystalline solids III: Kinetic model of the glass transition". Journal of Non-Crystalline Solids. 44 (1): 17–30. Bibcode:1981JNCS...44...17P. doi:10.1016/0022-3093(81)90129-0.
  7. ↑ Hall CC, Zhou C, Danielsen SP, Lodge TP (2016-03-22). "Formation of Multicompartment Ion Gels by Stepwise Self-Assembly of a Thermoresponsive ABC Triblock Terpolymer in an Ionic Liquid". Macromolecules. 49 (6): 2298–2306. Bibcode:2016MaMol..49.2298H. doi:10.1021/acs.macromol.5b02789. ISSN   0024-9297.
  8. ↑ Duplantier B (February 1989). "Statistical mechanics of polymer networks of any topology". Journal of Statistical Physics. 54 (3–4): 581–680. Bibcode:1989JSP....54..581D. doi:10.1007/BF01019770.
  9. 1 2 3 4 5 6 7 8 Tezuka Y, Oike H (November 2001). "Topological polymer chemistry: systematic classification of nonlinear polymer topologies". Journal of the American Chemical Society. 123 (47): 11570–6. doi:10.1021/ja0114409. PMID   11716710.
  10. ↑ Stadler FJ (May 2013). "Quantifying primary loops in polymer gels by linear viscoelasticity". Proceedings of the National Academy of Sciences of the United States of America. 110 (22): E1972. Bibcode:2013PNAS..110E1972S. doi: 10.1073/pnas.1303135110 . PMC   3670358 . PMID   23633573.
  11. ↑ Lange F, Schwenke K, Kurakazu M, Akagi Y, Chung UI, Lang M, Sommer JU, Sakai T, Saalwächter K (2011-12-27). "Connectivity and Structural Defects in Model Hydrogels: A Combined Proton NMR and Monte Carlo Simulation Study". Macromolecules. 44 (24): 9666–9674. Bibcode:2011MaMol..44.9666L. doi:10.1021/ma201847v. ISSN   0024-9297.
  12. ↑ Zhou H, Woo J, Cok AM, Wang M, Olsen BD, Johnson JA (November 2012). "Counting primary loops in polymer gels". Proceedings of the National Academy of Sciences of the United States of America. 109 (47): 19119–24. Bibcode:2012PNAS..10919119Z. doi: 10.1073/pnas.1213169109 . PMC   3511143 . PMID   23132947.
  13. 1 2 Oike H, Imaizumi H, Mouri T, Yoshioka Y, Uchibori A, Tezuka Y (October 2000). "Designing Unusual Polymer Topologies by Electrostatic Self-Assembly and Covalent Fixation". Journal of the American Chemical Society. 122 (40): 9592–9599. doi:10.1021/ja001736z.
  14. ↑ Yates CR, Hayes W (July 2004). "Synthesis and applications of hyperbranched polymers". European Polymer Journal. 40 (7): 1257–1281. doi:10.1016/j.eurpolymj.2004.02.007.
  15. ↑ Gao H, Matyjaszewski K (April 2009). "Synthesis of functional polymers with controlled architecture by CRP of monomers in the presence of cross-linkers: From stars to gels". Progress in Polymer Science. 34 (4): 317–350. doi:10.1016/j.progpolymsci.2009.01.001.
  16. ↑ Urayama K, Kawamura T, Kohjiya S (January 2009). "Structure–mechanical property correlations of model siloxane elastomers with controlled network topology". Polymer. 50 (2): 347–356. doi:10.1016/j.polymer.2008.10.027.
  17. ↑ Schüler F, Kerscher B, Beckert F, Thomann R, Mülhaupt R (January 2013). "Hyperbranched polymeric ionic liquids with onion-like topology as transporters and compartmentalized systems". Angewandte Chemie. 52 (1): 455–8. doi:10.1002/anie.201205130. PMID   23125048.
  18. ↑ Eddaoudi M, Kim J, Rosi N, Vodak D, Wachter J, O'Keeffe M, Yaghi OM (January 2002). "Systematic design of pore size and functionality in isoreticular MOFs and their application in methane storage". Science. 295 (5554): 469–72. Bibcode:2002Sci...295..469E. doi:10.1126/science.1067208. PMID   11799235. S2CID   39662564.
  19. ↑ D'Emanuele A, Attwood D (December 2005). "Dendrimer-drug interactions". Advanced Drug Delivery Reviews. 57 (15): 2147–62. doi:10.1016/j.addr.2005.09.012. PMID   16310283.
  20. ↑ Crooks RM, Zhao M, Sun L, Chechik V, Yeung LK (March 2001). "Dendrimer-encapsulated metal nanoparticles: synthesis, characterization, and applications to catalysis". Accounts of Chemical Research. 34 (3): 181–90. CiteSeerX   10.1.1.471.3897 . doi:10.1021/ar000110a. PMID   11263876.
  21. ↑ Delaviz Y, Gibson HW (September 1992). "Macrocyclic polymers. 2. Synthesis of poly(amide crown ethers) based on bis(5-carboxy-1,3-phenylene)-32-crown-10. Network formation through threading". Macromolecules. 25 (19): 4859–4862. Bibcode:1992MaMol..25.4859D. doi:10.1021/ma00045a005. ISSN   0024-9297.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.