Witting polytope

Last updated December 30, 2022

Witting polytope

Schläfli symbol	₃{3}₃{3}₃{3}₃
Coxeter diagram
Cells	240 ₃{3}₃{3}₃
Faces	2160 ₃{3}₃
Edges	2160 ₃{}
Vertices	240
Petrie polygon	30-gon
van Oss polygon	90 ₃{4}₃
Shephard group	L₄ = ₃[3]₃[3]₃[3]₃, order 155,520
Dual polyhedron	Self-dual
Properties	Regular

In 4-dimensional complex geometry, the Witting polytope is a regular complex polytope, named as: ₃{3}₃{3}₃{3}₃, and Coxeter diagram . It has 240 vertices, 2160 ₃{} edges, 2160 ₃{3}₃ faces, and 240 ₃{3}₃{3}₃ cells. It is self-dual. Each vertex belongs to 27 edges, 72 faces, and 27 cells, corresponding to the Hessian polyhedron vertex figure.

Symmetry

Its symmetry by ₃[3]₃[3]₃[3]₃ or , order 155,520.^[1] It has 240 copies of , order 648 at each cell.^[2]

Structure

The configuration matrix is:^[3] $\left[{\begin{smallmatrix}240&27&72&27\\3&2160&8&8\\8&8&2160&3\\27&72&27&240\end{smallmatrix}}\right]$

The number of vertices, edges, faces, and cells are seen in the diagonal of the matrix. These are computed by the order of the group divided by the order of the subgroup, by removing certain complex reflections, shown with X below. The number of elements of the k-faces are seen in rows below the diagonal. The number of elements in the vertex figure, etc., are given in rows above the digonal.

L₄	k-face	f_k	f₀	f₁	f₂	f₃	k-figure	Notes
L₃	( )	f₀	240	27	72	27	₃{3}₃{3}₃	L₄/L₃ = 216*6!/27/4! = 240
L₂L₁	₃{ }	f₁	3	2160	8	8	₃{3}₃	L₄/L₂L₁ = 216*6!/4!/3 = 2160
L₂L₁	₃{3}₃	f₂	8	8	2160	3	₃{ }	L₄/L₂L₁ = 216*6!/4!/3 = 2160
L₃	₃{3}₃{3}₃	f₃	27	72	27	240	( )	L₄/L₃ = 216*6!/27/4! = 240

Coordinates

Its 240 vertices are given coordinates in $\mathbb {C} ^{4}$ :

(0, ±ω^μ, -±ω^ν, ±ω^λ) (-±ω^μ, 0, ±ω^ν, ±ω^λ) (±ω^μ, -±ω^ν, 0, ±ω^λ) (-±ω^λ, -±ω^μ, -±ω^ν, 0)	(±iω^λ√3, 0, 0, 0) (0, ±iω^λ√3, 0, 0) (0, 0, ±iω^λ√3, 0) (0, 0, 0, ±iω^λ√3)

where $\omega ={\tfrac {-1+i{\sqrt {3}}}{2}},\lambda ,\nu ,\mu =0,1,2$ .

The last 6 points form hexagonal holes on one of its 40 diameters. There are 40 hyperplanes contain central ₃{3}₃{4}₂, figures, with 72 vertices.

Witting configuration

Coxeter named it after Alexander Witting for being a Witting configuration in complex projective 3-space:^[4]

\left[{\begin{smallmatrix}40&12&12\\2&240&2\\12&12&40\end{smallmatrix}}\right]

or

\left[{\begin{smallmatrix}40&9&12\\4&90&4\\12&9&40\end{smallmatrix}}\right]

The Witting configuration is related to the finite space PG(3,2²), consisting of 85 points, 357 lines, and 85 planes.^[5]

Related real polytope

Its 240 vertices are shared with the real 8-dimensional polytope 4₂₁, . Its 2160 3-edges are sometimes drawn as 6480 simple edges, slightly less than the 6720 edges of 4₂₁. The 240 difference is accounted by 40 central hexagons in 4₂₁ whose edges are not included in ₃{3}₃{3}₃{3}₃.^[6]

The honeycomb of Witting polytopes

The regular Witting polytope has one further stage as a 4-dimensional honeycomb, . It has the Witting polytope as both its facets, and vertex figure. It is self-dual, and its dual coincides with itself.^[7]

Hyperplane sections of this honeycomb include 3-dimensional honeycombs .

The honeycomb of Witting polytopes has a real representation as the 8-dimensional polytope 5₂₁, .

Its f-vector element counts are in proportion: 1, 80, 270, 80, 1.^[8] The configuration matrix for the honeycomb is:

L₅	k-face	f_k	f₀	f₁	f₂	f₃	f₄	k-figure	Notes
L₄	( )	f₀	N	240	2160	2160	240	₃{3}₃{3}₃{3}₃	L₅/L₄ = N
L₃L₁	₃{ }	f₁	3	80N	27	72	27	₃{3}₃{3}₃	L₅/L₃L₁ = 80N
L₂L₂	₃{3}₃	f₂	8	8	270N	8	8	₃{3}₃	L₅/L₂L₂ = 270N
L₃L₁	₃{3}₃{3}₃	f₃	27	72	27	80N	3	₃{}	L₅/L₃L₁ = 80N
L₄	₃{3}₃{3}₃{3}₃	f₄	240	2160	2160	240	N	( )	L₅/L₄ = N

Notes

↑ Coxeter Regular Convex Polytopes, 12.5 The Witting polytope
↑ Coxeter, Complex Regular Polytopes, p.134
↑ Coxeter, Complex Regular polytopes, p.132
↑ Alexander Witting, Ueber Jacobi'sche Functionen k^ter Ordnung Zweier Variabler, Mathemematische Annalen 29 (1887), 157-70, see especially p.169
↑ Coxeter, Complex regular polytopes, p.133
↑ Coxeter, Complex Regular Polytopes, p.134
↑ Coxeter, Complex Regular Polytopes, p.135
↑ Coxeter Regular Convex Polytopes, 12.5 The Witting polytope

Related Research Articles

In geometry, a tesseract is the four-dimensional analogue of the cube; the tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of six square faces, the hypersurface of the tesseract consists of eight cubical cells. The tesseract is one of the six convex regular 4-polytopes.

In geometry, the 24-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol {3,4,3}. It is also called C₂₄, or the icositetrachoron, octaplex (short for "octahedral complex"), icosatetrahedroid, octacube, hyper-diamond or polyoctahedron, being constructed of octahedral cells.

In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol {3,3,3}. It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C₅, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is the 4-simplex (Coxeter's $polytope), the simplest possible convex 4-polytope, and is analogous to the tetrahedron in three dimensions and the triangle in two dimensions. The 5-cell is a 4-dimensional pyramid with a tetrahedral base and four tetrahedral sides.$

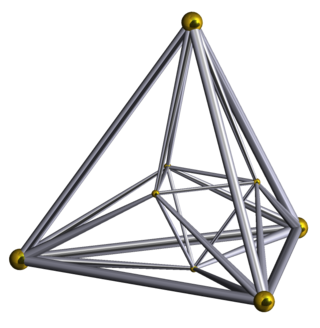
In geometry, the 16-cell is the regular convex 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol {3,3,4}. It is one of the six regular convex 4-polytopes first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century. It is also called C₁₆, hexadecachoron, or hexdecahedroid [sic?].

In four-dimensional geometry, a runcinated 5-cell is a convex uniform 4-polytope, being a runcination of the regular 5-cell.

In geometry, a truncated 5-cell is a uniform 4-polytope formed as the truncation of the regular 5-cell.

In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.

In geometry, a complex polytope is a generalization of a polytope in real space to an analogous structure in a complex Hilbert space, where each real dimension is accompanied by an imaginary one.

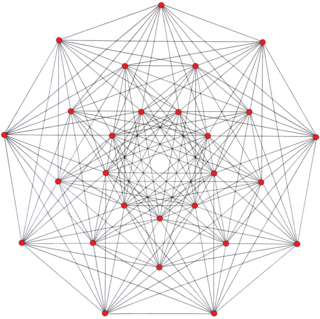
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos⁻¹(1/5), or approximately 78.46°.

In geometry, a 6-orthoplex, or 6-cross polytope, is a regular 6-polytope with 12 vertices, 60 edges, 160 triangle faces, 240 tetrahedron cells, 192 5-cell 4-faces, and 64 5-faces.

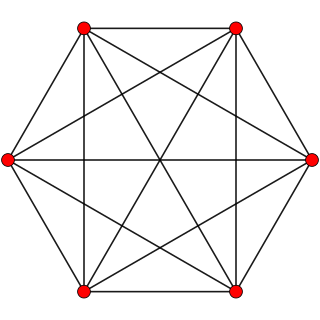
In five-dimensional geometry, a rectified 5-simplex is a convex uniform 5-polytope, being a rectification of the regular 5-simplex.

In 6-dimensional geometry, the 1₂₂ polytope is a uniform polytope, constructed from the E₆ group. It was first published in E. L. Elte's 1912 listing of semiregular polytopes, named as V₇₂ (for its 72 vertices).

In 8-dimensional geometry, the 1₄₂ is a uniform 8-polytope, constructed within the symmetry of the E₈ group.

In 8-dimensional geometry, the 2₄₁ is a uniform 8-polytope, constructed within the symmetry of the E₈ group.

In 8-dimensional geometry, the 4₂₁ is a semiregular uniform 8-polytope, constructed within the symmetry of the E₈ group. It was discovered by Thorold Gosset, published in his 1900 paper. He called it an 8-ic semi-regular figure.

In 7-dimensional geometry, the 3₃₁ honeycomb is a uniform honeycomb, also given by Schläfli symbol {3,3,3,3^3,1} and is composed of 3₂₁ and 7-simplex facets, with 56 and 576 of them respectively around each vertex.

In geometry, the 5₂₁ honeycomb is a uniform tessellation of 8-dimensional Euclidean space. The symbol 5₂₁ is from Coxeter, named for the length of the 3 branches of its Coxeter-Dynkin diagram.

In geometry, the simplectic honeycomb is a dimensional infinite series of honeycombs, based on the $affine Coxeter group symmetry. It is represented by a Coxeter-Dynkin diagram as a cyclic graph of n + 1 nodes with one node ringed. It is composed of n -simplex facets, along with all rectified n -simplices. It can be thought of as an n -dimensional hypercubic honeycomb that has been subdivided along all hyperplanes, then stretched along its main diagonal until the simplices on the ends of the hypercubes become regular. The vertex figure of an n - simplex honeycomb is an expanded n -simplex.$

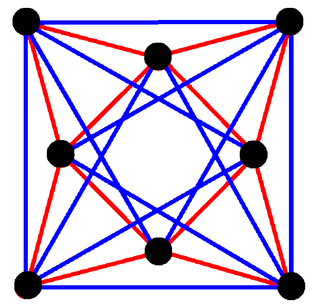
In geometry, the Hessian polyhedron is a regular complex polyhedron ₃{3}₃{3}₃, , in $. It has 27 vertices, 72 3 {} edges, and 27 3 {3} 3 faces. It is self-dual.$

In geometry, the Möbius–Kantor polygon is a regular complex polygon ₃{3}₃, , in $. 3 {3} 3 has 8 vertices, and 8 edges. It is self-dual. Every vertex is shared by 3 triangular edges. Coxeter named it a Möbius-Kantor polygon for sharing the complex configuration structure as the Möbius-Kantor configuration, (8 3).$

References

Coxeter, H. S. M. and Moser, W. O. J.; Generators and Relations for Discrete Groups (1965), esp pp 67–80.
Coxeter, H. S. M.; Regular Complex Polytopes, Cambridge University Press, second edition (1991). pp. 132–5, 143, 146, 152.
Coxeter, H. S. M. and Shephard, G.C.; Portraits of a family of complex polytopes, Leonardo Vol 25, No 3/4, (1992), pp 239–244

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Coxeter Regular Convex Polytopes, 12.5 The Witting polytope

[2] Coxeter, Complex Regular Polytopes, p.134

[3] Coxeter, Complex Regular polytopes, p.132

[4] Alexander Witting, Ueber Jacobi'sche Functionen k^ter Ordnung Zweier Variabler, Mathemematische Annalen 29 (1887), 157-70, see especially p.169

[5] Coxeter, Complex regular polytopes, p.133

[6] Coxeter, Complex Regular Polytopes, p.134

[7] Coxeter, Complex Regular Polytopes, p.135

[8] Coxeter Regular Convex Polytopes, 12.5 The Witting polytope

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]