
Yegor (Egor) Ivanovich Zolotaryov ( ‹See Tfd› Russian : Его́р Ива́нович Золотарёв) (31 March 1847, Saint Petersburg – 19 July 1878, Saint Petersburg) was a Russian mathematician.

Yegor (Egor) Ivanovich Zolotaryov ( ‹See Tfd› Russian : Его́р Ива́нович Золотарёв) (31 March 1847, Saint Petersburg – 19 July 1878, Saint Petersburg) was a Russian mathematician.
Yegor was born as a son of Agafya Izotovna Zolotaryova and the merchant Ivan Vasilevich Zolotaryov in Saint Petersburg, Imperial Russia. In 1857 he began to study at the fifth St Petersburg gymnasium, a school which centred on mathematics and natural science. He finished it with the silver medal in 1863. In the same year he was allowed to be an auditor at the physico-mathematical faculty of St Petersburg university.
He had not been able to become a student before 1864 because he was too young. Among his academic teachers were Somov, Chebyshev and Aleksandr Korkin, with whom he would have a tight scientific friendship. In November 1867 he defended his Kandidat thesis “About the Integration of Gyroscope Equations”, after 10 months there followed his thesis pro venia legendi About one question on Minima. With this work he was given the right to teach as a private lecturer at St Petersburg university.
He first lectured on differential calculus to science students (until summer 1871), later integral calculus and analysis to beginners of mathematics. Except for a short pause he lectured on elliptic functions to students of higher semesters during his whole job as lecturer and professor.
In December 1869, Zolotaryov defended his master's thesis “About the Solution of the Indefinite Equation of Third Degree x³ + Ay³ + A²z³ - 3Axyz = 1”.
He took his first trip abroad in 1872 and visited Berlin and Heidelberg. In Berlin he attended Weierstrass' "theory of analytic functions", in Heidelberg Koenigsberger's.
In 1874, Zolotaryov become a member of the university staff as a lecturer and in the same year he defended his doctoral thesis “Theory of integer Complex Numbers with an Application to Integral Calculus”. The algorithm Zolotaryov proved there was created by Chebyshev and that algorithm allowed to see whether integral of the form
was elementary, in this case representable in logarithms. This was a question Chebyshev had been interested in since the beginning of his research.
Starting at the beginning of the winter semester 1876 Zolotaryov was appointed extraordinary professor, and after the death of academician Somov he became his successor as an adjunct of the Academy of Sciences.
Egor Ivanovich Zolotaryov's steep career ended abruptly with his early death. He was on his way to his dacha when he was run over by a train in the Tsarskoe Selo station. On 19 July 1878 he died from blood poisoning.
Yegor Ivanovich is not to be confused with the probabilist Vladimir Mikhaelovich Zolotaryov, Kolmogorov's disciple, who worked on stable distributions with well known results on their parametrization. [1] [2]

Andrey Andreyevich Markov was a Russian mathematician best known for his work on stochastic processes. A primary subject of his research later became known as the Markov chain. He was also a strong, close to master-level, chess player.

Aleksandr Mikhailovich Lyapunov was a Russian mathematician, mechanician and physicist. His surname is variously romanized as Ljapunov, Liapunov, Liapounoff or Ljapunow. He was the son of the astronomer Mikhail Lyapunov and the brother of the pianist and composer Sergei Lyapunov.
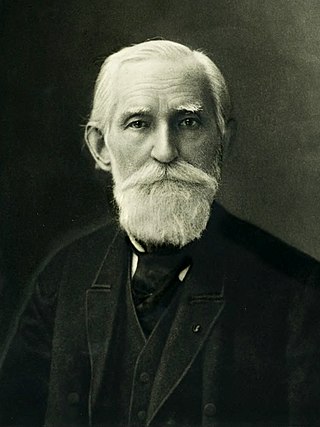
Pafnuty Lvovich Chebyshev was a Russian mathematician and considered to be the founding father of Russian mathematics.
Multilinear algebra is the study of functions with multiple vector-valued arguments, with the functions being linear maps with respect to each argument. It involves concepts such as matrices, tensors, multivectors, systems of linear equations, higher-dimensional spaces, determinants, inner and outer products, and dual spaces. It is a mathematical tool used in engineering, machine learning, physics, and mathematics.
In symbolic computation, the Risch algorithm is a method of indefinite integration used in some computer algebra systems to find antiderivatives. It is named after the American mathematician Robert Henry Risch, a specialist in computer algebra who developed it in 1968.

Paul Albert Gordan was a Jewish-German mathematician, a student of Carl Jacobi at the University of Königsberg before obtaining his PhD at the University of Breslau (1862), and a professor at the University of Erlangen-Nuremberg.
Hilbert's nineteenth problem is one of the 23 Hilbert problems, set out in a list compiled by David Hilbert in 1900. It asks whether the solutions of regular problems in the calculus of variations are always analytic. Informally, and perhaps less directly, since Hilbert's concept of a "regular variational problem" identifies this precisely as a variational problem whose Euler–Lagrange equation is an elliptic partial differential equation with analytic coefficients, Hilbert's nineteenth problem, despite its seemingly technical statement, simply asks whether, in this class of partial differential equations, any solution inherits the relatively simple and well understood property of being an analytic function from the equation it satisfies. Hilbert's nineteenth problem was solved independently in the late 1950s by Ennio De Giorgi and John Forbes Nash, Jr.
In number theory, Zolotarev's lemma states that the Legendre symbol

Dimitrie D. Pompeiu was a Romanian mathematician, professor at the University of Bucharest, titular member of the Romanian Academy, and President of the Chamber of Deputies.
Aleksandr Nikolayevich Korkin was a Russian mathematician. He made contribution to the development of partial differential equations, and was second only to Chebyshev among the founders of the Saint Petersburg Mathematical School. Among others, his students included Yegor Ivanovich Zolotarev.

Paul David Gustav du Bois-Reymond was a German mathematician who was born in Berlin and died in Freiburg. He was the brother of Emil du Bois-Reymond.

Thierry Aubin was a French mathematician who worked at the Centre de Mathématiques de Jussieu, and was a leading expert on Riemannian geometry and non-linear partial differential equations. His fundamental contributions to the theory of the Yamabe equation led, in conjunction with results of Trudinger and Schoen, to a proof of the Yamabe Conjecture: every compact Riemannian manifold can be conformally rescaled to produce a manifold of constant scalar curvature. Along with Yau, he also showed that Kähler manifolds with negative first Chern classes always admit Kähler–Einstein metrics, a result closely related to the Calabi conjecture. The latter result, established by Yau, provides the largest class of known examples of compact Einstein manifolds. Aubin was the first mathematician to propose the Cartan–Hadamard conjecture.
In mathematics, a perfect lattice is a lattice in a Euclidean vector space, that is completely determined by the set S of its minimal vectors in the sense that there is only one positive definite quadratic form taking value 1 at all points of S. Perfect lattices were introduced by Korkine & Zolotareff (1877). A strongly perfect lattice is one whose minimal vectors form a spherical 4-design. This notion was introduced by Venkov (2001).
In mathematics, a eutactic lattice (or eutactic form) is a lattice in Euclidean space whose minimal vectors form a eutactic star. This means they have a set of positive eutactic coefficientsci such that (v, v) = Σci(v, mi)2 where the sum is over the minimal vectors mi. "Eutactic" is derived from the Greek language, and means "well-situated" or "well-arranged".

Georgios Remoundos was a Greek mathematician and a founding member of the Academy of Athens in 1926.
Adolphe Buhl was a French mathematician and astronomer.

Dmitry Fyodorovich Selivanov, was a Russian mathematician, known for his work on differential calculus and finite difference calculus.
In mathematics, Zolotarev polynomials are polynomials used in approximation theory. They are sometimes used as an alternative to the Chebyshev polynomials where accuracy of approximation near the origin is of less importance. Zolotarev polynomials differ from the Chebyshev polynomials in that two of the coefficients are fixed in advance rather than allowed to take on any value. The Chebyshev polynomials of the first kind are a special case of Zolotarev polynomials. These polynomials were introduced by Russian mathematician Yegor Ivanovich Zolotarev in 1868.
The Korkine–Zolotarev (KZ) lattice basis reduction algorithm or Hermite–Korkine–Zolotarev (HKZ) algorithm is a lattice reduction algorithm.