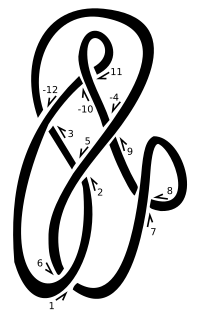In the mathematical field of topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life, such as those in shoelaces and rope, a mathematical knot differs in that the ends are joined so it cannot be undone, the simplest knot being a ring. In mathematical language, a knot is an embedding of a circle in 3-dimensional Euclidean space, . Two mathematical knots are equivalent if one can be transformed into the other via a deformation of upon itself ; these transformations correspond to manipulations of a knotted string that do not involve cutting it or passing through itself.

Peter Guthrie Tait FRSE was a Scottish mathematical physicist and early pioneer in thermodynamics. He is best known for the mathematical physics textbook Treatise on Natural Philosophy, which he co-wrote with Lord Kelvin, and his early investigations into knot theory.

In mathematics, the Borromean rings are three simple closed curves in three-dimensional space that are topologically linked and cannot be separated from each other, but that break apart into two unknotted and unlinked loops when any one of the three is cut or removed. Most commonly, these rings are drawn as three circles in the plane, in the pattern of a Venn diagram, alternatingly crossing over and under each other at the points where they cross. Other triples of curves are said to form the Borromean rings as long as they are topologically equivalent to the curves depicted in this drawing.
In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a Laurent polynomial in the variable with integer coefficients.

In knot theory, a knot or link diagram is alternating if the crossings alternate under, over, under, over, as one travels along each component of the link. A link is alternating if it has an alternating diagram.
In mathematics, Khovanov homology is an oriented link invariant that arises as the homology of a chain complex. It may be regarded as a categorification of the Jones polynomial.
In the mathematical theory of knots, a finite type invariant, or Vassiliev invariant, is a knot invariant that can be extended to an invariant of certain singular knots that vanishes on singular knots with m + 1 singularities and does not vanish on some singular knot with 'm' singularities. It is then said to be of type or order m.
In the mathematical field of knot theory, the bracket polynomial is a polynomial invariant of framed links. Although it is not an invariant of knots or links, a suitably "normalized" version yields the famous knot invariant called the Jones polynomial. The bracket polynomial plays an important role in unifying the Jones polynomial with other quantum invariants. In particular, Kauffman's interpretation of the Jones polynomial allows generalization to invariants of 3-manifolds.
In the mathematical field of knot theory, a chiral knot is a knot that is not equivalent to its mirror image. An oriented knot that is equivalent to its mirror image is an amphicheiral knot, also called an achiral knot. The chirality of a knot is a knot invariant. A knot's chirality can be further classified depending on whether or not it is invertible.
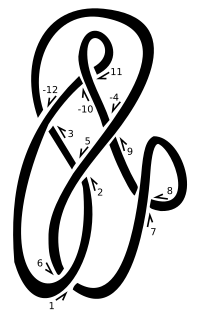
In the mathematical field of knot theory, the Dowker–Thistlethwaite (DT) notation or code, for a knot is a sequence of even integers. The notation is named after Clifford Hugh Dowker and Morwen Thistlethwaite, who refined a notation originally due to Peter Guthrie Tait.
In knot theory, a virtual knot is a generalization of knots in 3-dimensional Euclidean space, R3, to knots in thickened surfaces modulo an equivalence relation called stabilization/destabilization. Here is required to be closed and oriented. Virtual knots were first introduced by Kauffman (1999).
In the mathematical field of knot theory, the Arf invariant of a knot, named after Cahit Arf, is a knot invariant obtained from a quadratic form associated to a Seifert surface. If F is a Seifert surface of a knot, then the homology group H1(F, Z/2Z) has a quadratic form whose value is the number of full twists mod 2 in a neighborhood of an embedded circle representing an element of the homology group. The Arf invariant of this quadratic form is the Arf invariant of the knot.

Louis Hirsch Kauffman is an American mathematician, topologist, and professor of mathematics in the Department of Mathematics, Statistics, and Computer science at the University of Illinois at Chicago. He is known for the introduction and development of the bracket polynomial and the Kauffman polynomial.
In knot theory, the Kauffman polynomial is a 2-variable knot polynomial due to Louis Kauffman. It is initially defined on a link diagram as

Morwen Bernard Thistlethwaite is a knot theorist and professor of mathematics for the University of Tennessee in Knoxville. He has made important contributions to both knot theory and Rubik's Cube group theory.
William W. Menasco is a topologist and a professor at the University at Buffalo. He is best known for his work in knot theory.
In the mathematical theory of knots, a flype is a kind of manipulation of knot and link diagrams used in the Tait flyping conjecture. It consists of twisting a part of a knot, a tangle T, by 180 degrees. Flype comes from a Scots word meaning to fold or to turn back. Two reduced alternating diagrams of an alternating link can be transformed to each other using flypes. This is the Tait flyping conjecture, proven in 1991 by Morwen Thistlethwaite and William Menasco.
In mathematics, especially in the area of topology known as knot theory, an invertible knot is a knot that can be continuously deformed to itself, but with its orientation reversed. A non-invertible knot is any knot which does not have this property. The invertibility of a knot is a knot invariant. An invertible link is the link equivalent of an invertible knot.
Hernando Burgos Soto is a Canadian writer and mathematician, professor of mathematics at George Brown College. He is the author of several math papers in which he introduced some mathematics concepts and extended to tangles some celebrated results of knot theory about the Khovanov homology and the Jones polynomial. During his career as a mathematician, his interests have included Mathematical Statistics, Knot Theory, Algebraic Topology and more recently Mathematical Finance. He is comfortable writing in English and Spanish. When writing in Spanish, he works in the area of prose fiction writing short stories. Some of his short stories were published at the website Cuentos y Cuentos.