In statistical mechanics, the virial theorem provides a general equation that relates the average over time of the total kinetic energy of a stable system of discrete particles, bound by a conservative force, with that of the total potential energy of the system. Mathematically, the theorem states
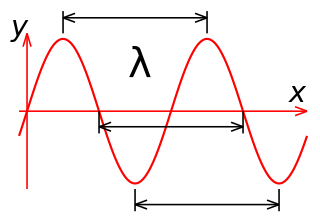
In physics and mathematics, wavelength or spatial period of a wave or periodic function is the distance over which the wave's shape repeats. In other words, it is the distance between consecutive corresponding points of the same phase on the wave, such as two adjacent crests, troughs, or zero crossings. Wavelength is a characteristic of both traveling waves and standing waves, as well as other spatial wave patterns. The inverse of the wavelength is called the spatial frequency. Wavelength is commonly designated by the Greek letter lambda (λ). The term "wavelength" is also sometimes applied to modulated waves, and to the sinusoidal envelopes of modulated waves or waves formed by interference of several sinusoids.
In physics, a Langevin equation is a stochastic differential equation describing how a system evolves when subjected to a combination of deterministic and fluctuating ("random") forces. The dependent variables in a Langevin equation typically are collective (macroscopic) variables changing only slowly in comparison to the other (microscopic) variables of the system. The fast (microscopic) variables are responsible for the stochastic nature of the Langevin equation. One application is to Brownian motion, which models the fluctuating motion of a small particle in a fluid.
In physics, specifically in quantum mechanics, a coherent state is the specific quantum state of the quantum harmonic oscillator, often described as a state which has dynamics most closely resembling the oscillatory behavior of a classical harmonic oscillator. It was the first example of quantum dynamics when Erwin Schrödinger derived it in 1926, while searching for solutions of the Schrödinger equation that satisfy the correspondence principle. The quantum harmonic oscillator arise in the quantum theory of a wide range of physical systems. For instance, a coherent state describes the oscillating motion of a particle confined in a quadratic potential well. The coherent state describes a state in a system for which the ground-state wavepacket is displaced from the origin of the system. This state can be related to classical solutions by a particle oscillating with an amplitude equivalent to the displacement.

Deposition is the geological process in which sediments, soil and rocks are added to a landform or landmass. Wind, ice, water, and gravity transport previously weathered surface material, which, at the loss of enough kinetic energy in the fluid, is deposited, building up layers of sediment.

The Drude model of electrical conduction was proposed in 1900 by Paul Drude to explain the transport properties of electrons in materials. Basically, Ohm's law was well established and stated that the current J and voltage V driving the current are related to the resistance R of the material. The inverse of the resistance is known as the conductance. When we consider a metal of unit length and unit cross sectional area, the conductance is known as the conductivity, which is the inverse of resistivity. The Drude model attempts to explain the resistivity of a conductor in terms of the scattering of electrons by the relatively immobile ions in the metal that act like obstructions to the flow of electrons.

In fluid dynamics, a wind wave, or wind-generated water wave, is a surface wave that occurs on the free surface of bodies of water as a result of the wind blowing over the water's surface. The contact distance in the direction of the wind is known as the fetch. Waves in the oceans can travel thousands of kilometers before reaching land. Wind waves on Earth range in size from small ripples to waves over 30 m (100 ft) high, being limited by wind speed, duration, fetch, and water depth.
The Green–Kubo relations give the exact mathematical expression for a transport coefficients in terms of the integral of the equilibrium time correlation function of the time derivative of a corresponding microscopic variable :
In fluid dynamics, dispersion of water waves generally refers to frequency dispersion, which means that waves of different wavelengths travel at different phase speeds. Water waves, in this context, are waves propagating on the water surface, with gravity and surface tension as the restoring forces. As a result, water with a free surface is generally considered to be a dispersive medium.

In geology, ripple marks are sedimentary structures and indicate agitation by water or wind.
When waves travel into areas of shallow water, they begin to be affected by the ocean bottom. The free orbital motion of the water is disrupted, and water particles in orbital motion no longer return to their original position. As the water becomes shallower, the swell becomes higher and steeper, ultimately assuming the familiar sharp-crested wave shape. After the wave breaks, it becomes a wave of translation and erosion of the ocean bottom intensifies.

In fluid dynamics, the Boussinesq approximation for water waves is an approximation valid for weakly non-linear and fairly long waves. The approximation is named after Joseph Boussinesq, who first derived them in response to the observation by John Scott Russell of the wave of translation. The 1872 paper of Boussinesq introduces the equations now known as the Boussinesq equations.

In fluid dynamics, a Stokes wave is a nonlinear and periodic surface wave on an inviscid fluid layer of constant mean depth. This type of modelling has its origins in the mid 19th century when Sir George Stokes – using a perturbation series approach, now known as the Stokes expansion – obtained approximate solutions for nonlinear wave motion.

In fluid dynamics, wave shoaling is the effect by which surface waves, entering shallower water, change in wave height. It is caused by the fact that the group velocity, which is also the wave-energy transport velocity, changes with water depth. Under stationary conditions, a decrease in transport speed must be compensated by an increase in energy density in order to maintain a constant energy flux. Shoaling waves will also exhibit a reduction in wavelength while the frequency remains constant.

In fluid dynamics, the Ursell number indicates the nonlinearity of long surface gravity waves on a fluid layer. This dimensionless parameter is named after Fritz Ursell, who discussed its significance in 1953.
In fluid dynamics, Airy wave theory gives a linearised description of the propagation of gravity waves on the surface of a homogeneous fluid layer. The theory assumes that the fluid layer has a uniform mean depth, and that the fluid flow is inviscid, incompressible and irrotational. This theory was first published, in correct form, by George Biddell Airy in the 19th century.
In physical oceanography, undertow is the undercurrent that moves offshore while waves approach the shore. Undertow is a natural and universal feature for almost any large body of water; it is a return flow compensating for the onshore-directed average transport of water by the waves in the zone above the wave troughs. The undertow's flow velocities are generally strongest in the surf zone, where the water is shallow and the waves are high due to shoaling.
Tides in marginal seas are tides affected by their location in semi-enclosed areas along the margins of continents and differ from tides in the open oceans. Tides are water level variations caused by the gravitational interaction between the Moon, the Sun and the Earth. The resulting tidal force is a secondary effect of gravity: it is the difference between the actual gravitational force and the centrifugal force. While the centrifugal force is constant across the Earth, the gravitational force is dependent on the distance between the two bodies and is therefore not constant across the Earth. The tidal force is thus the difference between these two forces on each location on the Earth.
Nonlinear tides are generated by hydrodynamic distortions of tides. A tidal wave is said to be nonlinear when its shape deviates from a pure sinusoidal wave. In mathematical terms, the wave owes its nonlinearity due to the nonlinear advection and frictional terms in the governing equations. These become more important in shallow-water regions such as in estuaries. Nonlinear tides are studied in the fields of coastal morphodynamics, coastal engineering and physical oceanography. The nonlinearity of tides has important implications for the transport of sediment.

Internal wave breaking is a process during which internal gravity waves attain a large amplitude compared to their length scale, become nonlinearly unstable and finally break. This process is accompanied by turbulent dissipation and mixing. As internal gravity waves carry energy and momentum from the environment of their inception, breaking and subsequent turbulent mixing affects the fluid characteristics in locations of breaking. Consequently, internal wave breaking influences even the large scale flows and composition in both the ocean and the atmosphere. In the atmosphere, momentum deposition by internal wave breaking plays a key role in atmospheric phenomena such as the Quasi-Biennial Oscillation and the Brewer-Dobson Circulation. In the deep ocean, mixing induced by internal wave breaking is an important driver of the meridional overturning circulation. On smaller scales, breaking-induced mixing is important for sediment transport and for nutrient supply to the photic zone. Most breaking of oceanic internal waves occurs in continental shelves, well below the ocean surface, which makes it a difficult phenomenon to observe.


























