Abacus school

Last updated

Abacus school is a term applied to any Italian school or tutorial after the 13th century, whose commerce-directed curriculum placed special emphasis on mathematics, such as algebra, among other subjects. [1] These schools sprang up after the publication of Fibonacci's Book of the Abacus and his introduction of the Hindu–Arabic numeral system. In Fibonacci's viewpoint, this system, originating in India around 400 BCE, and later adopted by the Arabs, was simpler and more practical than using the existing Roman numeric tradition. Italian merchants and traders quickly adopted the structure as a means of producing accountants, clerks, and so on, and subsequently abacus schools for students were established. [2] These were done in many ways: communes could appeal to patrons to support the institution and find masters; religious institutions could finance and oversee the curriculum; independent masters could teach pupils. Unless they were selected for teaching occupations that were salaried, most masters taught students who could pay as this was their main source of income. [3]

Contents

The words abacus or abaco refers to calculations, especially the subject of direct calculations, and does not imply the use of an abacus. [4]

Significance

Abacus schools were significant for a couple of reasons:

Firstly, because mathematics was associated with many professions, including trade, [5] there was an increasing need to do away with the old Roman numeral system which produced too many errors. [6] The number of Roman characters a merchant needed to memorize to carry out financial transactions as opposed to Hindu-numerals made the switch practical. Commercialists were first introduced to this new system through Leonardo Fibonacci, who came from a business family and had studied Arabic math. Being convinced of its uses, abacus schools were therefore created and dominated by wealthy merchants, with some exceptions. [5] [7] Sons could now be trained by the best and brightest teachers to take over their family business and the fortunate poor had more access to a variety of vocations. [8] Morality also played a role in determining the school attendance of commoners. [9]

Secondly, reading, writing, and some elementary math as job requirements for general occupations [10] meant that literacy levels rose with the number of ordinary students attending institutions or being tutored at home. Sailors, for example, who wished to climb the social ladder had to present literacy and arithmetic skills on their résumé. [11] Aspiring abbaco masters themselves need have studied only elementary, or secondary abbaco in order to teach others. [12]

School system

Italian abacus school systems differed more in their establishment than in their curriculum during the Middle Ages. For example, institutions and appointed educators were set up in a number of ways, either through commune patronage or independent masters' personal funds. Some abbaco teachers tutored privately in homes. All instructors, however, were contractually bound to their agreement which usually meant that they could supplement their salary with tuition fees or other rates. [13] Curriculum for Abbaco masters was also universal, in that lessons were directed towards solving commercial problems. [14] Still, these primary and secondary math schools were not to be confused with university level math subjects. [15]

Establishment

Wealthy merchants, because of their substantial influence on public governments and their desire to educate their sons in commercial mathematics, initiated constructions of schools with the support of other parents. [16] Communal governments then proceeded to attract abbaco masters from elsewhere, so as to avoid partiality and inevitable dispute, and to select the best according to qualification, age and salary request. They drew up contracts that specified the number of years an appointed master must work, the number of students he was allowed to teach, and the certain percentage of fees he was to return to the commune. [17] Contracts usually ranged from twenty to thirty ducats or florins, depending on the currency used, for one to three years. In return, communes, would grant tax exemptions (full or half), rights to collect fees (tuition fees, textbook and school supply sales), and a house for personal use over and above the masters annual salary. [18]

Independent teachers could also be hired by the commune, but for lower wages. [19] Most times, freelance masters were contracted by a group of parents in a similar fashion to that of communal agreements, thus establishing their own school if the number of students being tutored was significant in size. [20] Abbaco apprentices training to become masters could also tutor household children and pay for their studies simultaneously. Upon graduation, however, apprentices were required to teach elsewhere for fear of stealing the master's students and income. [21]

Curriculum

Arithmetic, geometry, bookkeeping, reading and writing in the vernacular were the basic elementary and secondary subjects in the abbaco syllabus for most institutions, which began in the fall, Mondays through Saturdays. [22] Although Grendler states that the hours students spent in school could be very long and lasting half a year to eight months, [23] Merry Wiesner-Hanks takes the opposite view: boys and girls only spent half a day for up to four months. [24] Here, Grendler may be considering the wealthy commoners and higher social classes, whereas Wiesner-Hanks may be looking at the working classes.

Mathematical problems dealt with the everyday exchange of different types of goods or monies of differing values, whether it was in demand or in good quality, and how much of it was being traded. Other problems dealt with distribution of profits, where each member invested a certain sum and may have later withdrawn a portion of that amount. Labor contracts too, where the employer agreed to a certain wage over the course of a certain term for a certain type of work that produced a specific amount of goods, but the employee decided to leave after a while, were brought up. Bookkeeping taught students to note weight, length, size and other quantitative and qualitative information of goods. [25]

See also

Notes

  1. ↑ Michael of Rhodes Project Team, Dibner Institute, and WGBH Interactive. "Abacus School". Glossary. Archived March 14, 2008, at the Wayback Machine Michael of Rhodes. Institute and Museum of the History of Science. 2005. Sunday, March 23, 2008.
  2. ↑ Michael of Rhodes Project Team, Dibner Institute, and WGBH Interactive. "Mathematics: The Abacus Tradition". Archived November 15, 2009, at the Wayback Machine A Mariner's Knowledge. Michael of Rhodes. Institute and Museum of the History of Science. 2005. Sunday, March 23, 2008.
  3. ↑ Grendler, 1989, Page 5.
  4. ↑ Leonardo Fibonacci. “Fibonacci’s Liber Abaci: Leonardo Pisano’s Book of Calculation”. Contributor Laurence Edward Sigler. [1202]. Springer. 2003. Page 4.
  5. 1 2 Grendler, 1989, Page 22.
  6. ↑ Robert M. Yerkes. “The Use of Roman Numerals”. Science. New series. Vol. 20. No. 505. American Association for the Advancement of Science. September 2, 1904. Pages 309-310.
  7. ↑ Hans Baron. “The Social Background of Political Liberty in the Early Italian Renaissance”. Comparative Studies in Society and History. Vol. 2. No. 4. Cambridge University Press. July 1960. Page 440.
  8. ↑ Grendler, 1989, Page 104.
  9. ↑ Grendler, 1989, Page 13.
  10. ↑ Grendler, 1989, Page 12.
  11. ↑ Michael of Rhodes Project Team, Dibner Institute, and WGBH Interactive. "Michael's Life". Archived April 20, 2008, at the Wayback Machine Michael of Rhodes. Institute and Museum of the History of Science. 2005. Sunday, March 23, 2008.
  12. ↑ Grendler, 1989, Page 36.
  13. ↑ Grendler, 1989, Page 15.
  14. ↑ Michael of Rhodes Project Team, Dibner Institute, and WGBH Interactive. "Mathematics: Why Math?". Archived February 18, 2007, at the Wayback Machine A Mariner's Knowledge. Michael of Rhodes. Institute and Museum of the History of Science. 2005. Sunday, March 23, 2008.
  15. ↑ Grendler, 1989, Page 306.
  16. ↑ Grendler, 1989, Page 2.
  17. ↑ Grendler, 1989, Page 19.
  18. ↑ Grendler, 1989, pages 15–17, 31.
  19. ↑ Grendler, 1989, Page 33.
  20. ↑ Grendler, 1989, Page 30.
  21. ↑ Grendler, 1989, page 41.
  22. ↑ Grendler, 1989, Page 22, 34.
  23. ↑ Grendler, 1989, Page 34.
  24. ↑ Merry E. Wiesner-Hanks. “Early Modern Europe, 1450-1789”. Cambridge: Cambridge University Press, 2006. Page 120.
  25. ↑ Luis Radford. “On the Epistemological Limits of Language: Mathematical Knowledge and Social Practice During the Renaissance”. Educational Studies in Mathematics. Vol. 52. No. 2. Springer. 2003. Pages 127-129, 131)

Related Research Articles

<span class="mw-page-title-main">Abacus</span> Calculating tool

An abacus, also called a counting frame, is a hand-operated calculating tool which was used from ancient times in the ancient Near East, Europe, China, and Russia, until the adoption of the Hindu-Arabic numeral system. An abacus consists of a two-dimensional array of slidable beads. In their earliest designs, the beads could be loose on a flat surface or sliding in grooves. Later the beads were made to slide on rods and built into a frame, allowing faster manipulation.

The ten Arabic numerals 0, 1, 2, 3, 4, 5, 6, 7, 8, and 9 are the most commonly used symbols for writing numbers. The term often also implies a positional notation using the numerals, as well as the use of a decimal base, in particular when contrasted with other systems such as Roman numerals. However, the symbols are also used to write numbers in other bases such as octal, as well as for writing non-numerical information such as trademarks or license plates identifiers.

<span class="mw-page-title-main">History of mathematics</span>

The history of mathematics deals with the origin of discoveries in mathematics and the mathematical methods and notation of the past. Before the modern age and the worldwide spread of knowledge, written examples of new mathematical developments have come to light only in a few locales. From 3000 BC the Mesopotamian states of Sumer, Akkad and Assyria, followed closely by Ancient Egypt and the Levantine state of Ebla began using arithmetic, algebra and geometry for purposes of taxation, commerce, trade and also in the patterns in nature, the field of astronomy and to record time and formulate calendars.

<span class="mw-page-title-main">Fibonacci</span> Italian mathematician (c. 1170–1245)

Fibonacci, also known as Leonardo Bonacci, Leonardo of Pisa, or Leonardo Bigollo Pisano, was an Italian mathematician from the Republic of Pisa, considered to be "the most talented Western mathematician of the Middle Ages".

<span class="mw-page-title-main">Luca Pacioli</span> Italian mathematician and cleric

Fra. Luca Bartolomeo de Pacioli was an Italian mathematician, Franciscan friar, collaborator with Leonardo da Vinci, and an early contributor to the field now known as accounting. He is referred to as the father of accounting and bookkeeping and he was the first person to publish a work on the double-entry system of book-keeping on the continent. He was also called Luca di Borgo after his birthplace, Borgo Sansepolcro, Tuscany.

0 (zero) is a number representing an empty quantity. Adding 0 to any number leaves that number unchanged. In mathematical terminology, 0 is the additive identity of the integers, rational numbers, real numbers, and complex numbers, as well as other algebraic structures. Multiplying any number by 0 has the result 0, and consequently, division by zero has no meaning in arithmetic.

<i>Liber Abaci</i> Mathematics book written in 1202 by Fibonacci

Liber Abaci is a historic 1202 Latin manuscript on arithmetic by Leonardo of Pisa, posthumously known as Fibonacci.

A numerical digit is a single symbol used alone or in combinations, to represent numbers in a positional numeral system. The name "digit" comes from the fact that the ten digits of the hands correspond to the ten symbols of the common base 10 numeral system, i.e. the decimal digits.

<span class="mw-page-title-main">Algorism</span> Mathematical technique for arithmetic

Algorism is the technique of performing basic arithmetic by writing numbers in place value form and applying a set of memorized rules and facts to the digits. One who practices algorism is known as an algorist. This positional notation system has largely superseded earlier calculation systems that used a different set of symbols for each numerical magnitude, such as Roman numerals, and in some cases required a device such as an abacus.

<span class="mw-page-title-main">University of Parma</span> Public university in Parma, Italy

The University of Parma is a public university in Parma, Emilia-Romagna, Italy. It is organised in nine departments. As of 2016 the University of Parma has about 26,000 students.

<span class="mw-page-title-main">Latin school</span> School type in Europe from the 14th to 19th centuries

The Latin school was the grammar school of 14th- to 19th-century Europe, though the latter term was much more common in England. Other terms used include Lateinschule in Germany, or later Gymnasium. Latin schools were also established in Colonial America.

In Italy, the laurea is the main post-secondary academic degree. The name originally referred literally to the laurel wreath, since ancient times a sign of honor and now worn by Italian students right after their official graduation ceremony and sometimes during the graduation party. A graduate is known as a laureato, literally "crowned with laurel."

<span class="mw-page-title-main">Hindu–Arabic numeral system</span> Most common system for writing numbers

The Hindu–Arabic numeral system or Indo-Arabic numeral system is a positional base ten numeral system for representing integers; its extension to non-integers is the decimal numeral system, which is presently the most common numeral system.

The Book of Squares, (Liber Quadratorum in the original Latin) is a book on algebra by Leonardo Fibonacci, published in 1225. It was dedicated to Frederick II, Holy Roman Emperor.

After being brought to Pisa by Master Dominick to the feet of your celestial majesty, most glorious prince, Lord F.,

<span class="mw-page-title-main">Bern Dibner</span> American historian

Bern Dibner was an electrical engineer, industrialist, and historian of science and technology. He originated two major US library collections in the history of science and technology.

Europe's achievements in science and technology have been significant and research and development efforts form an integral part of the European economy. Europe has been the home of some of the most prominent researchers in various scientific disciplines, notably physics, mathematics, chemistry and engineering. Scientific research in Europe is supported by industry, by the European universities and by several scientific institutions. All the raw output of scientific research from Europe consistently ranks among the world's best.

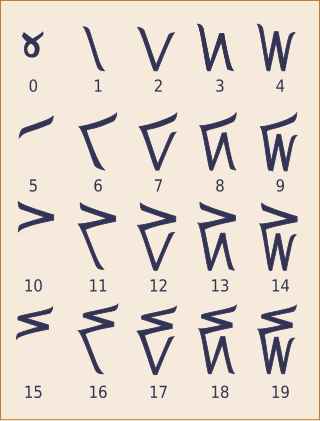
<span class="mw-page-title-main">Kaktovik numerals</span> Inuit numeral system for a base-20 counting system

The Kaktovik numerals or Kaktovik Iñupiaq numerals are a base-20 system of numerical digits created by Alaskan Iñupiat. They are visually iconic, with shapes that indicate the number being represented.

<span class="mw-page-title-main">Mathematics and art</span> Relationship between mathematics and art

Mathematics and art are related in a variety of ways. Mathematics has itself been described as an art motivated by beauty. Mathematics can be discerned in arts such as music, dance, painting, architecture, sculpture, and textiles. This article focuses, however, on mathematics in the visual arts.

<span class="mw-page-title-main">Henry W. Gould</span> American mathematician

Henry Wadsworth Gould is a Professor Emeritus of Mathematics at West Virginia University.

<i>Summa de arithmetica</i> Renaissance mathematics textbook

Summa de arithmetica, geometria, proportioni et proportionalita is a book on mathematics written by Luca Pacioli and first published in 1494. It contains a comprehensive summary of Renaissance mathematics, including practical arithmetic, basic algebra, basic geometry and accounting, written for use as a textbook and reference work.

References

Further reading

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.