In a chemical reaction, chemical equilibrium is the state in which both the reactants and products are present in concentrations which have no further tendency to change with time, so that there is no observable change in the properties of the system. This state results when the forward reaction proceeds at the same rate as the reverse reaction. The reaction rates of the forward and backward reactions are generally not zero, but they are equal. Thus, there are no net changes in the concentrations of the reactants and products. Such a state is known as dynamic equilibrium.

Friction is the force resisting the relative motion of solid surfaces, fluid layers, and material elements sliding against each other. Types of friction include dry, fluid, lubricated, skin, and internal.
In physics, mean free path is the average distance over which a moving particle travels before substantially changing its direction or energy, typically as a result of one or more successive collisions with other particles.
Tribology is the science and engineering of understanding friction, lubrication and wear phenomena for interacting surfaces in relative motion. It is highly interdisciplinary, drawing on many academic fields, including physics, chemistry, materials science, mathematics, biology and engineering. The fundamental objects of study in tribology are tribosystems, which are physical systems of contacting surfaces. Subfields of tribology include biotribology, nanotribology and space tribology. It is also related to other areas such as the coupling of corrosion and tribology in tribocorrosion and the contact mechanics of how surfaces in contact deform. Approximately 20% of the total energy expenditure of the world is due to the impact of friction and wear in the transportation, manufacturing, power generation, and residential sectors.

Projectile motion is a form of motion experienced by an object or particle that is projected in a gravitational field, such as from Earth's surface, and moves along a curved path under the action of gravity only. In the particular case of projectile motion on Earth, most calculations assume the effects of air resistance are passive and negligible.

In mechanics, the normal force is the component of a contact force that is perpendicular to the surface that an object contacts. In this instance normal is used in the geometric sense and means perpendicular, as opposed to the common language use of normal meaning "ordinary" or "expected". A person standing still on a platform is acted upon by gravity, which would pull them down towards the Earth's core unless there were a countervailing force from the resistance of the platform's molecules, a force which is named the "normal force".

Rolling resistance, sometimes called rolling friction or rolling drag, is the force resisting the motion when a body rolls on a surface. It is mainly caused by non-elastic effects; that is, not all the energy needed for deformation of the wheel, roadbed, etc., is recovered when the pressure is removed. Two forms of this are hysteresis losses, and permanent (plastic) deformation of the object or the surface. Note that the slippage between the wheel and the surface also results in energy dissipation. Although some researchers have included this term in rolling resistance, some suggest that this dissipation term should be treated separately from rolling resistance because it is due to the applied torque to the wheel and the resultant slip between the wheel and ground, which is called slip loss or slip resistance. In addition, only the so-called slip resistance involves friction, therefore the name "rolling friction" is to an extent a misnomer.
In fluid dynamics, drag, sometimes referred to as fluid resistance, is a force acting opposite to the relative motion of any object, moving with respect to a surrounding fluid. This can exist between two fluid layers, two solid surfaces, or between a fluid and solid surface. Drag forces tend to decrease fluid velocity relative to the solid object in the fluid's path.

A skid mark is the visible mark left by any solid which moves against another, and is an important aspect of trace evidence analysis in forensic science and forensic engineering. Skid marks caused by tires on roads occur when a vehicle wheel stops rolling and slides or spins on the surface of the road. Skid marks can be analyzed to find the maximum and minimum vehicle speed prior to an impact or incident. Skidding can also occur on black ice or diesel deposits on the road and may not leave a mark at all.

A leadscrew, also known as a power screw or translation screw, is a screw used as a linkage in a machine, to translate turning motion into linear motion. Because of the large area of sliding contact between their male and female members, screw threads have larger frictional energy losses compared to other linkages. They are not typically used to carry high power, but more for intermittent use in low power actuator and positioner mechanisms. Leadscrews are commonly used in linear actuators, machine slides, vises, presses, and jacks. Leadscrews are a common component in electric linear actuators.
A banked turn is a turn or change of direction in which the vehicle banks or inclines, usually towards the inside of the turn. For a road or railroad this is usually due to the roadbed having a transverse down-slope towards the inside of the curve. The bank angle is the angle at which the vehicle is inclined about its longitudinal axis with respect to the horizontal.

Uniform Tire Quality Grading, commonly abbreviated as UTQG, is a set of standards for passenger car tires that measures a tire's treadwear, temperature resistance and traction. The UTQG was created by the National Highway Traffic Safety Administration in 1978, a branch of the United States Department of Transportation (DOT). All passenger car tires manufactured for sale in the United States since March 31, 1979 are federally mandated to have the UTQG ratings on their sidewall as part of the DOT approval process, in which non-DOT approved tires are not legal for street use in the United States. Light truck tires are not required to have a UTQG. It is not to be confused with the tire code, a supplemental and global standard measuring tire dimensions, load-bearing ability and maximum speed, maintained by tire industry trade organizations and the International Organization for Standardization.

Bicycle and motorcycle dynamics is the science of the motion of bicycles and motorcycles and their components, due to the forces acting on them. Dynamics falls under a branch of physics known as classical mechanics. Bike motions of interest include balancing, steering, braking, accelerating, suspension activation, and vibration. The study of these motions began in the late 19th century and continues today.
Headway is the distance or duration between vehicles in a transit system measured in space or time. The minimum headway is the shortest such distance or time achievable by a system without a reduction in the speed of vehicles. The precise definition varies depending on the application, but it is most commonly measured as the distance from the tip of one vehicle to the tip of the next one behind it. It can be expressed as the distance between vehicles, or as time it will take for the trailing vehicle to cover that distance. A "shorter" headway signifies closer spacing between the vehicles. Airplanes operate with headways measured in hours or days, freight trains and commuter rail systems might have headways measured in parts of an hour, metro and light rail systems operate with headways on the order of 90 seconds to 20 minutes, and vehicles on a freeway can have as little as 2 seconds headway between them.
Sliding is a type of motion between two surfaces in contact. This can be contrasted to rolling motion. Both types of motion may occur in bearings.
In the design of fluid bearings, the Sommerfeld number (S) is a dimensionless quantity used extensively in hydrodynamic lubrication analysis. The Sommerfeld number is very important in lubrication analysis because it contains all the variables normally specified by the designer.
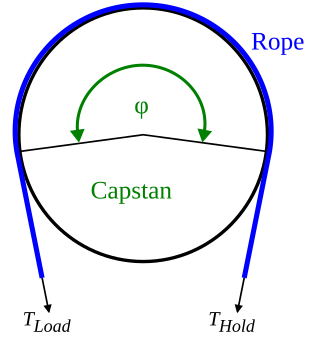
The capstan equation or belt friction equation, also known as Euler-Eytelwein formula, relates the hold-force to the load-force if a flexible line is wound around a cylinder.

The geometric design of roads is the branch of highway engineering concerned with the positioning of the physical elements of the roadway according to standards and constraints. The basic objectives in geometric design are to optimize efficiency and safety while minimizing cost and environmental damage. Geometric design also affects an emerging fifth objective called "livability", which is defined as designing roads to foster broader community goals, including providing access to employment, schools, businesses and residences, accommodate a range of travel modes such as walking, bicycling, transit, and automobiles, and minimizing fuel use, emissions and environmental damage.
Contact mechanics is the study of the deformation of solids that touch each other at one or more points. This can be divided into compressive and adhesive forces in the direction perpendicular to the interface, and frictional forces in the tangential direction. Frictional contact mechanics is the study of the deformation of bodies in the presence of frictional effects, whereas frictionless contact mechanics assumes the absence of such effects.
In legal terminology, the assured clear distance ahead (ACDA) is the distance ahead of any terrestrial locomotive device such as a land vehicle, typically an automobile, or watercraft, within which they should be able to bring the device to a halt. It is one of the most fundamental principles governing ordinary care and the duty of care for all methods of conveyance, and is frequently used to determine if a driver is in proper control and is a nearly universally implicit consideration in vehicular accident liability. The rule is a precautionary trivial burden required to avert the great probable gravity of precious life loss and momentous damage. Satisfying the ACDA rule is necessary but not sufficient to comply with the more generalized basic speed law, and accordingly, it may be used as both a layman's criterion and judicial test for courts to use in determining if a particular speed is negligent, but not to prove it is safe. As a spatial standard of care, it also serves as required explicit and fair notice of prohibited conduct so unsafe speed laws are not void for vagueness. The concept has transcended into accident reconstruction and engineering.






























