
In mathematics, two quantities are in the golden ratio if their ratio is the same as the ratio of their sum to the larger of the two quantities. Expressed algebraically, for quantities and with ,
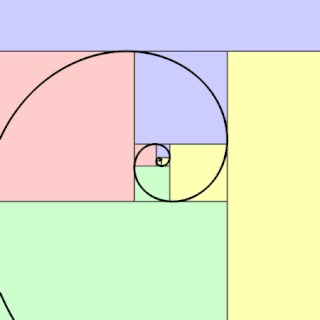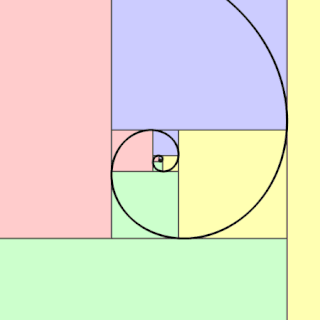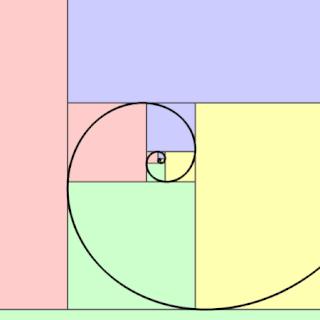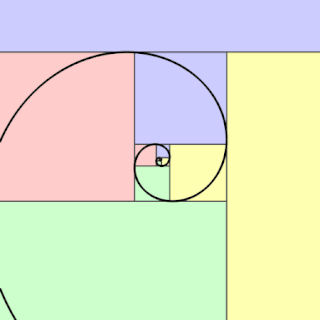
In geometry, a golden spiral is a logarithmic spiral whose growth factor is φ, the golden ratio. That is, a golden spiral gets wider by a factor of φ for every quarter turn it makes.

In geometry, a golden rectangle is a rectangle whose side lengths are in the golden ratio, , which is , where is approximately 1.618.

In mathematics, two quantities are in the silver ratio if the ratio of the smaller of those two quantities to the larger quantity is the same as the ratio of the larger quantity to the sum of the smaller quantity and twice the larger quantity. This defines the silver ratio as an irrational mathematical constant, whose value of one plus the square root of 2 is approximately 2.4142135623. Its name is an allusion to the golden ratio; analogously to the way the golden ratio is the limiting ratio of consecutive Fibonacci numbers, the silver ratio is the limiting ratio of consecutive Pell numbers. The silver ratio is denoted by δS.

Baroness Ingrid Daubechies is a Belgian physicist and mathematician. She is best known for her work with wavelets in image compression.

In mathematics, the plastic ratio is a geometrical proportion close to 53/40. Its true value is the real solution of the equation .

Donald in Mathmagic Land is a 1959 American live-action animated featurette produced by Walt Disney Productions and featuring Donald Duck. The short was directed by Hamilton Luske and was released on June 26, 1959. It was nominated for an Academy Award for Best Documentary at the 32nd Academy Awards, and became a widely viewed educational film in American schools of the 1960s and beyond.

Daina Taimiņa is a Latvian mathematician, retired adjunct associate professor of mathematics at Cornell University, known for developing a way of modeling hyperbolic geometry with crocheted objects.
Abū Kāmil Shujāʿ ibn Aslam ibn Muḥammad Ibn Shujāʿ was a prominent Egyptian mathematician during the Islamic Golden Age. He is considered the first mathematician to systematically use and accept irrational numbers as solutions and coefficients to equations. His mathematical techniques were later adopted by Fibonacci, thus allowing Abu Kamil an important part in introducing algebra to Europe.

Ideas from mathematics have been used as inspiration for fiber arts including quilt making, knitting, cross-stitch, crochet, embroidery and weaving. A wide range of mathematical concepts have been used as inspiration including topology, graph theory, number theory and algebra. Some techniques such as counted-thread embroidery are naturally geometrical; other kinds of textile provide a ready means for the colorful physical expression of mathematical concepts.
Jessica Katherine Sklar is a mathematician interested in abstract algebra, recreational mathematics, mathematics and art, and mathematics and popular culture. She is a professor of mathematics at Pacific Lutheran University, and former head of the mathematics department at Pacific Lutheran.

Mathematical beauty is the aesthetic pleasure derived from the abstractness, purity, simplicity, depth or orderliness of mathematics. Mathematicians may express this pleasure by describing mathematics as beautiful or describe mathematics as an art form, or, at a minimum, as a creative activity.

Mathematics and art are related in a variety of ways. Mathematics has itself been described as an art motivated by beauty. Mathematics can be discerned in arts such as music, dance, painting, architecture, sculpture, and textiles. This article focuses, however, on mathematics in the visual arts.

In the geometry of tessellations, a rep-tile or reptile is a shape that can be dissected into smaller copies of the same shape. The term was coined as a pun on animal reptiles by recreational mathematician Solomon W. Golomb and popularized by Martin Gardner in his "Mathematical Games" column in the May 1963 issue of Scientific American. In 2012 a generalization of rep-tiles called self-tiling tile sets was introduced by Lee Sallows in Mathematics Magazine.

Alexander Bellos is a British writer, broadcaster and mathematics communicator. He is the author of books about Brazil and mathematics, as well as having a column in The Guardian newspaper.
Jennifer McLoud-Mann is an American mathematician known for her 2015 discovery, with Casey Mann and undergraduate student David Von Derau, of the 15th and last class of convex pentagons to tile the plane. She is a professor of mathematics at the University of Washington Bothell, where she is currently the Vice Dean of Curriculum & Instruction of the School of STEM. Beyond tiling, her research interests include knot theory and combinatorics.
Susan Goldstine is an American mathematician active in mathematics and fiber arts. She is a professor of mathematics at St. Mary's College of Maryland, and the Steven Muller Distinguished Professor in the Sciences at St. Mary's College.

Solving the Riddle of Phyllotaxis: Why the Fibonacci Numbers and the Golden Ratio Occur in Plants is a book on the mathematics of plant structure, and in particular on phyllotaxis, the arrangement of leaves on plant stems. It was written by Irving Adler, and published in 2012 by World Scientific. The Basic Library List Committee of the Mathematical Association of America has suggested its inclusion in undergraduate mathematics libraries.

Henry Segerman is an Associate Professor of mathematics at Oklahoma State University in Stillwater, Oklahoma who does research in three-dimensional geometry and topology, especially three-manifolds, triangulations and hyperbolic geometry.

Mathemalchemy is a traveling art installation dedicated to a celebration of the intersection of art and mathematics. It is a collaborative work led by Duke University mathematician Ingrid Daubechies and fiber artist Dominique Ehrmann. The cross-disciplinary team of 24 people, who collectively built the installation during the calendar years 2020 and 2021, includes artists, mathematicians, and craftspeople who employed a wide variety of materials to illustrate, amuse, and educate the public on the wonders, mystery, and beauty of mathematics. Including the core team of 24, about 70 people contributed in some way to the realization of Mathemalchemy.

















