In kinematics, an eight-bar linkage is a mechanism with one degree of freedom that is constructed from eight links and ten joints. [1] These linkages are rare compared to four-bar and six-bar linkages, but two well-known examples are the Peaucellier linkage and the linkage designed by Theo Jansen for his walking machines.
Eight-bar linkages are classified by how many binary, ternary and quaternary links they have. A binary link connects two joints, a ternary link connects three joints and a quaternary link connects four joints. There are three classes of eight-bar linkage denoted (4, 4, 0, 0), (5, 2, 1, 0) and (6, 0, 2, 0), distinguished by the count of binary, ternary and quaternary links, when read from left to right---the final zero is traditionally added to the class label though no eight-bar linkage has a quintanary link.. [2]
There are sixteen different topologies of eight-bar linkages which are distinguished by their non-isomorphic linkage graphs. Of these 16 topologies, nine are in class (4, 4, 0, 0), five are in (5, 2, 1, 0) and two in (6, 0, 2, 0).
The Peaucellier linkage (or Peaucellier–Lipkin cell, or Peaucellier–Lipkin Inversor) is an eight-bar linkage constructed from hinged joints that traces a pure straight line from a rotary input. It is named after Charles-Nicolas Peaucellier (1832–1913), a French army officer, and Yom Tov Lipman Lipkin (1846–1876), a Lithuanian Jew and son of the famed Rabbi Israel Salanter. [3] [4]
This linkage clearly consists of eight bars when the ground frame is counted as a bar. The Chebychev–Grübler–Kutzbach criterion shows that an eight-bar linkage must have ten single degree-of-freedom joints, while the Peaucellier linkage appears to have only six hinged joints. This is resolved by noting that four of the hinged joints each connect three bars. This is considered to be a special case of two joints that are located in the same place. Thus, six plus four provides the 10 one degree-of-freedom joints.
The Peaucellier linkage is a (4, 4, 0, 0) eight-bar linkage, which means four of the bars have two joints and four bars have three joints.
The eight bars of the Jansen linkage, which include the ground frame, are readily identified, and include two triangular links. In this case only seven of the 10 hinged joints are readily identified. However, there are three joints that connect three links. The first is the end of the drive crank, the second is the other base pivot and the third is one side of the triangle that forms the lower leg. Separating these overlapping joints provides three additional joints so there are 10 single degree-of-freedom joints.
The Jansen linkage is of type (5, 2, 1, 0), because the upper triangular link supports four joints, two of which overlap at the ground joint, the lower triangular link and the input crank connect three joints and are ternary links. The remaining five links, which includes the ground link, are binary links.
A machine is a physical system that uses power to apply forces and control movement to perform an action. The term is commonly applied to artificial devices, such as those employing engines or motors, but also to natural biological macromolecules, such as molecular machines. Machines can be driven by animals and people, by natural forces such as wind and water, and by chemical, thermal, or electrical power, and include a system of mechanisms that shape the actuator input to achieve a specific application of output forces and movement. They can also include computers and sensors that monitor performance and plan movement, often called mechanical systems.
Franz Reuleaux was a German mechanical engineer and a lecturer of the Berlin Royal Technical Academy, later appointed as the president of the academy. He was often called the father of kinematics. He was a leader in his profession, contributing to many important domains of science and knowledge.
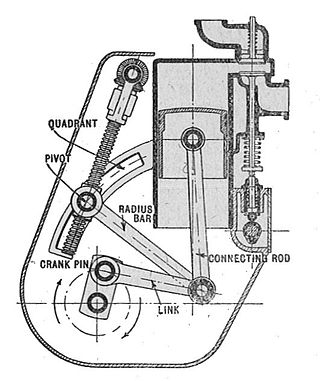
In kinematics, the parallel motion linkage is a six-bar mechanical linkage invented by the Scottish engineer James Watt in 1784 for the double-acting Watt steam engine. It allows a rod moving practically straight up and down to transmit motion to a beam moving in an arc, without putting significant sideways strain on the rod.
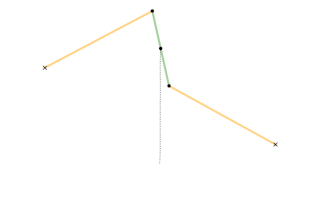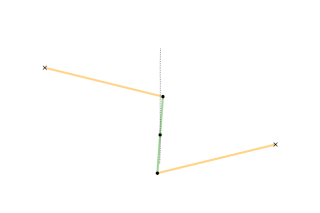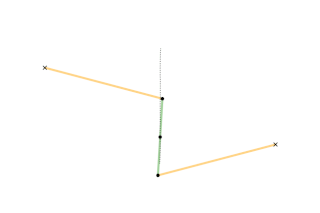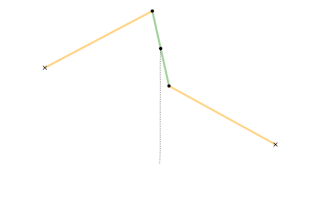
In kinematics, Watt's linkage is a type of mechanical linkage invented by James Watt in which the central moving point of the linkage is constrained to travel on a nearly straight line. It was described in Watt's patent specification of 1784 for the Watt steam engine.
In the study of mechanisms, a four-bar linkage, also called a four-bar, is the simplest closed-chain movable linkage. It consists of four bodies, called bars or links, connected in a loop by four joints. Generally, the joints are configured so the links move in parallel planes, and the assembly is called a planar four-bar linkage. Spherical and spatial four-bar linkages also exist and are used in practice.
A mechanical linkage is an assembly of systems connected to manage forces and movement. The movement of a body, or link, is studied using geometry so the link is considered to be rigid. The connections between links are modeled as providing ideal movement, pure rotation or sliding for example, and are called joints. A linkage modeled as a network of rigid links and ideal joints is called a kinematic chain.
The Peaucellier–Lipkin linkage, invented in 1864, was the first true planar straight line mechanism – the first planar linkage capable of transforming rotary motion into perfect straight-line motion, and vice versa. It is named after Charles-Nicolas Peaucellier (1832–1913), a French army officer, and Yom Tov Lipman Lipkin (1846–1876), a Lithuanian Jew and son of the famed Rabbi Israel Salanter.
In physics, the degrees of freedom (DOF) of a mechanical system is the number of independent parameters that define its configuration or state. It is important in the analysis of systems of bodies in mechanical engineering, structural engineering, aerospace engineering, robotics, and other fields.
In mechanical engineering, an overconstrained mechanism is a linkage that has more degrees of freedom than is predicted by the mobility formula. The mobility formula evaluates the degree of freedom of a system of rigid bodies that results when constraints are imposed in the form of joints between the links.
In classical mechanics, a kinematic pair is a connection between two physical objects that imposes constraints on their relative movement (kinematics). German engineer Franz Reuleaux introduced the kinematic pair as a new approach to the study of machines that provided an advance over the motion of elements consisting of simple machines.
In mechanical engineering, a kinematic chain is an assembly of rigid bodies connected by joints to provide constrained motion that is the mathematical model for a mechanical system. As the word chain suggests, the rigid bodies, or links, are constrained by their connections to other links. An example is the simple open chain formed by links connected in series, like the usual chain, which is the kinematic model for a typical robot manipulator.
A straight-line mechanism is a mechanism that converts any type of rotary or angular motion to perfect or near-perfect straight-line motion, or vice versa. Straight-line motion is linear motion of definite length or "stroke", every forward stroke being followed by a return stroke, giving reciprocating motion. The first such mechanism, patented in 1784 by James Watt, produced approximate straight-line motion, referred to by Watt as parallel motion.
The Sarrus linkage, invented in 1853 by Pierre Frédéric Sarrus, is a mechanical linkage to convert a limited circular motion to a linear motion or vice versa without reference guideways. It is a spatial six-bar linkage (6R) with two groups of three parallel adjacent joint-axes.
In kinematics, cognate linkages are linkages that ensure the same coupler curve geometry or input-output relationship, while being dimensionally dissimilar. In case of four-bar linkage coupler cognates, the Roberts–Chebyshev Theorem, after Samuel Roberts and Pafnuty Chebyshev, states that each coupler curve can be generated by three different four-bar linkages. These four-bar linkages can be constructed using similar triangles and parallelograms, and the Cayley diagram.
The Chebychev–Grübler–Kutzbach criterion determines the number of degrees of freedom of a kinematic chain, that is, a coupling of rigid bodies by means of mechanical constraints. These devices are also called linkages.
The Klannlinkage is a planar mechanism designed to simulate the gait of legged animal and function as a wheel replacement, a leg mechanism. The linkage consists of the frame, a crank, two grounded rockers, and two couplers all connected by pivot joints. It was developed by Joe Klann in 1994 as an expansion of Burmester curves which are used to develop four-bar double-rocker linkages such as harbor crane booms. It is categorized as a modified Stephenson type III kinematic chain.
In engineering, a mechanism is a device that transforms input forces and movement into a desired set of output forces and movement. Mechanisms generally consist of moving components which may include:
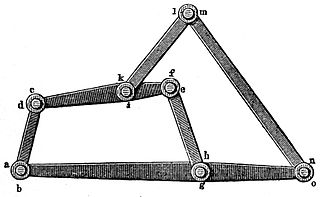
In mechanics, a six-bar linkage is a mechanism with one degree of freedom that is constructed from six links and seven joints. An example is the Klann linkage used to drive the legs of a walking machine.
A leg mechanism is a mechanical system designed to provide a propulsive force by intermittent frictional contact with the ground. This is in contrast with wheels or continuous tracks which are intended to maintain continuous frictional contact with the ground. Mechanical legs are linkages that can have one or more actuators, and can perform simple planar or complex motion. Compared to a wheel, a leg mechanism is potentially better fitted to uneven terrain, as it can step over obstacles.
In kinematics, a five-bar linkage is a mechanism with two degrees of freedom that is constructed from five links that are connected together in a closed chain. All links are connected to each other by five joints in series forming a loop. One of the links is the ground or base. This configuration is also called a pantograph, however, it is not to be confused with the parallelogram-copying linkage pantograph.