
A biological membrane, biomembrane or cell membrane is a selectively permeable membrane that separates the interior of a cell from the external environment or creates intracellular compartments by serving as a boundary between one part of the cell and another. Biological membranes, in the form of eukaryotic cell membranes, consist of a phospholipid bilayer with embedded, integral and peripheral proteins used in communication and transportation of chemicals and ions. The bulk of lipids in a cell membrane provides a fluid matrix for proteins to rotate and laterally diffuse for physiological functioning. Proteins are adapted to high membrane fluidity environment of the lipid bilayer with the presence of an annular lipid shell, consisting of lipid molecules bound tightly to the surface of integral membrane proteins. The cell membranes are different from the isolating tissues formed by layers of cells, such as mucous membranes, basement membranes, and serous membranes.

In cell biology, a vesicle is a structure within or outside a cell, consisting of liquid or cytoplasm enclosed by a lipid bilayer. Vesicles form naturally during the processes of secretion (exocytosis), uptake (endocytosis), and the transport of materials within the plasma membrane. Alternatively, they may be prepared artificially, in which case they are called liposomes. If there is only one phospholipid bilayer, the vesicles are called unilamellar liposomes; otherwise they are called multilamellar liposomes. The membrane enclosing the vesicle is also a lamellar phase, similar to that of the plasma membrane, and intracellular vesicles can fuse with the plasma membrane to release their contents outside the cell. Vesicles can also fuse with other organelles within the cell. A vesicle released from the cell is known as an extracellular vesicle.

The lipid bilayer is a thin polar membrane made of two layers of lipid molecules. These membranes are flat sheets that form a continuous barrier around all cells. The cell membranes of almost all organisms and many viruses are made of a lipid bilayer, as are the nuclear membrane surrounding the cell nucleus, and membranes of the membrane-bound organelles in the cell. The lipid bilayer is the barrier that keeps ions, proteins and other molecules where they are needed and prevents them from diffusing into areas where they should not be. Lipid bilayers are ideally suited to this role, even though they are only a few nanometers in width, because they are impermeable to most water-soluble (hydrophilic) molecules. Bilayers are particularly impermeable to ions, which allows cells to regulate salt concentrations and pH by transporting ions across their membranes using proteins called ion pumps.
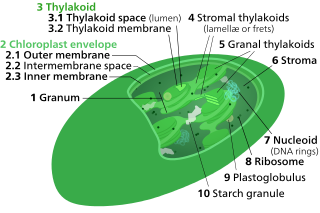
Thylakoids are membrane-bound compartments inside chloroplasts and cyanobacteria. They are the site of the light-dependent reactions of photosynthesis. Thylakoids consist of a thylakoid membrane surrounding a thylakoid lumen. Chloroplast thylakoids frequently form stacks of disks referred to as grana. Grana are connected by intergranal or stromal thylakoids, which join granum stacks together as a single functional compartment.

The fluid mosaic model explains various characteristics regarding the structure of functional cell membranes. According to this biological model, there is a lipid bilayer in which protein molecules are embedded. The phospholipid bilayer gives fluidity and elasticity to the membrane. Small amounts of carbohydrates are also found in the cell membrane. The biological model, which was devised by Seymour Jonathan Singer and Garth L. Nicolson in 1972, describes the cell membrane as a two-dimensional liquid that restricts the lateral diffusion of membrane components. Such domains are defined by the existence of regions within the membrane with special lipid and protein cocoon that promote the formation of lipid rafts or protein and glycoprotein complexes. Another way to define membrane domains is the association of the lipid membrane with the cytoskeleton filaments and the extracellular matrix through membrane proteins. The current model describes important features relevant to many cellular processes, including: cell-cell signaling, apoptosis, cell division, membrane budding, and cell fusion. The fluid mosaic model is the most acceptable model of the plasma membrane. In this definition of the cell membrane, its main function is to act as a barrier between the contents inside the cell and the extracellular environment.
Phosphatidic acids are anionic phospholipids important to cell signaling and direct activation of lipid-gated ion channels. Hydrolysis of phosphatidic acid gives rise to one molecule each of glycerol and phosphoric acid and two molecules of fatty acids. They constitute about 0.25% of phospholipids in the bilayer.

SNARE proteins – "SNAPREceptors" – are a large protein family consisting of at least 24 members in yeasts, more than 60 members in mammalian cells, and some numbers in plants. The primary role of SNARE proteins is to mediate the fusion of vesicles with the target membrane; this notably mediates exocytosis, but can also mediate the fusion of vesicles with membrane-bound compartments. The best studied SNAREs are those that mediate the release of synaptic vesicles containing neurotransmitters in neurons. These neuronal SNAREs are the targets of the neurotoxins responsible for botulism and tetanus produced by certain bacteria.

ADP-ribosylation factor GTPase-activating protein 1 is an enzyme that in humans is encoded by the ARFGAP1 gene. Two transcript variants encoding different isoforms have been found for this gene.

Erich Sackmann is a German experimental physicist and a pioneer of biophysics in Europe.
Lipid bilayer mechanics is the study of the physical material properties of lipid bilayers, classifying bilayer behavior with stress and strain rather than biochemical interactions. Local point deformations such as membrane protein interactions are typically modelled with the complex theory of biological liquid crystals but the mechanical properties of a homogeneous bilayer are often characterized in terms of only three mechanical elastic moduli: the area expansion modulus Ka, a bending modulus Kb and an edge energy . For fluid bilayers the shear modulus is by definition zero, as the free rearrangement of molecules within plane means that the structure will not support shear stresses. These mechanical properties affect several membrane-mediated biological processes. In particular, the values of Ka and Kb affect the ability of proteins and small molecules to insert into the bilayer. Bilayer mechanical properties have also been shown to alter the function of mechanically activated ion channels.
One property of a lipid bilayer is the relative mobility (fluidity) of the individual lipid molecules and how this mobility changes with temperature. This response is known as the phase behavior of the bilayer. Broadly, at a given temperature a lipid bilayer can exist in either a liquid or a solid phase. The solid phase is commonly referred to as a “gel” phase. All lipids have a characteristic temperature at which they undergo a transition (melt) from the gel to liquid phase. In both phases the lipid molecules are constrained to the two dimensional plane of the membrane, but in liquid phase bilayers the molecules diffuse freely within this plane. Thus, in a liquid bilayer a given lipid will rapidly exchange locations with its neighbor millions of times a second and will, through the process of a random walk, migrate over long distances.

In membrane biology, fusion is the process by which two initially distinct lipid bilayers merge their hydrophobic cores, resulting in one interconnected structure. If this fusion proceeds completely through both leaflets of both bilayers, an aqueous bridge is formed and the internal contents of the two structures can mix. Alternatively, if only one leaflet from each bilayer is involved in the fusion process, the bilayers are said to be hemifused. In hemifusion, the lipid constituents of the outer leaflet of the two bilayers can mix, but the inner leaflets remain distinct. The aqueous contents enclosed by each bilayer also remain separated.
A model lipid bilayer is any bilayer assembled in vitro, as opposed to the bilayer of natural cell membranes or covering various sub-cellular structures like the nucleus. They are used to study the fundamental properties of biological membranes in a simplified and well-controlled environment, and increasingly in bottom-up synthetic biology for the construction of artificial cells. A model bilayer can be made with either synthetic or natural lipids. The simplest model systems contain only a single pure synthetic lipid. More physiologically relevant model bilayers can be made with mixtures of several synthetic or natural lipids.
Membrane curvature is the geometrical measure or characterization of the curvature of membranes. The membranes can be naturally occurring or man-made (synthetic). An example of naturally occurring membrane is the lipid bilayer of cells, also known as cellular membranes. Synthetic membranes can be obtained by preparing aqueous solutions of certain lipids. The lipids will then "aggregate" and form various phases and structures. According to the conditions and the chemical structures of the lipid, different phases will be observed. For instance, the lipid POPC tends to form lamellar vesicles in solution, whereas smaller lipids, such as detergents, will form micelles if the CMC was reached. There are five commonly proposed mechanisms by which membrane curvature is created, maintained, or controlled: lipid composition, shaped transmembrane proteins, protein motif insertion/BAR domains, protein scaffolding, and cytoskeleton scaffolding.
Hydrophobic mismatch is the difference between the thicknesses of hydrophobic regions of a transmembrane protein and of the biological membrane it spans. In order to avoid unfavorable exposure of hydrophobic surfaces to water, the hydrophobic regions of transmembrane proteins are expected to have approximately the same thickness as the hydrophobic region of the surrounding lipid bilayer. Nevertheless, the same membrane protein can be encountered in bilayers of different thickness. In eukaryotic cells, the plasma membrane is thicker than the membranes of the endoplasmic reticulum. Yet all proteins that are abundant in the plasma membrane are initially integrated into the endoplasmic reticulum upon synthesis on ribosomes. Transmembrane peptides or proteins and surrounding lipids can adapt to the hydrophobic mismatch by different means.
Mechanosensitive channels (MSCs), mechanosensitive ion channels or stretch-gated ion channels are membrane proteins capable of responding to mechanical stress over a wide dynamic range of external mechanical stimuli. They are present in the membranes of organisms from the three domains of life: bacteria, archaea, and eukarya. They are the sensors for a number of systems including the senses of touch, hearing and balance, as well as participating in cardiovascular regulation and osmotic homeostasis (e.g. thirst). The channels vary in selectivity for the permeating ions from nonselective between anions and cations in bacteria, to cation selective allowing passage Ca2+, K+ and Na+ in eukaryotes, and highly selective K+ channels in bacteria and eukaryotes.
Vesicle fusion is the merging of a vesicle with other vesicles or a part of a cell membrane. In the latter case, it is the end stage of secretion from secretory vesicles, where their contents are expelled from the cell through exocytosis. Vesicles can also fuse with other target cell compartments, such as a lysosome. Exocytosis occurs when secretory vesicles transiently dock and fuse at the base of cup-shaped structures at the cell plasma membrane called porosome, the universal secretory machinery in cells. Vesicle fusion may depend on SNARE proteins in the presence of increased intracellular calcium (Ca2+) concentration.

The Saffman–Delbrück model describes a lipid membrane as a thin layer of viscous fluid, surrounded by a less viscous bulk liquid. This picture was originally proposed to determine the diffusion coefficient of membrane proteins, but has also been used to describe the dynamics of fluid domains within lipid membranes. The Saffman–Delbrück formula is often applied to determine the size of an object embedded in a membrane from its observed diffusion coefficient, and is characterized by the weak logarithmic dependence of diffusion constant on object radius.

The cell membrane is a biological membrane that separates and protects the interior of a cell from the outside environment. The cell membrane consists of a lipid bilayer, made up of two layers of phospholipids with cholesterols interspersed between them, maintaining appropriate membrane fluidity at various temperatures. The membrane also contains membrane proteins, including integral proteins that span the membrane and serve as membrane transporters, and peripheral proteins that loosely attach to the outer (peripheral) side of the cell membrane, acting as enzymes to facilitate interaction with the cell's environment. Glycolipids embedded in the outer lipid layer serve a similar purpose. The cell membrane controls the movement of substances in and out of a cell, being selectively permeable to ions and organic molecules. In addition, cell membranes are involved in a variety of cellular processes such as cell adhesion, ion conductivity, and cell signalling and serve as the attachment surface for several extracellular structures, including the cell wall and the carbohydrate layer called the glycocalyx, as well as the intracellular network of protein fibers called the cytoskeleton. In the field of synthetic biology, cell membranes can be artificially reassembled.
A unilamellar liposome is a spherical liposome, a vesicle, bounded by a single bilayer of an amphiphilic lipid or a mixture of such lipids, containing aqueous solution inside the chamber. Unilamellar liposomes are used to study biological systems and to mimic cell membranes, and are classified into three groups based on their size: small unilamellar liposomes/vesicles (SUVs) that with a size range of 20–100 nm, large unilamellar liposomes/vesicles (LUVs) with a size range of 100–1000 nm and giant unilamellar liposomes/vesicles (GUVs) with a size range of 1-200 µm. GUVs are mostly used as models for biological membranes in research work. Animal cells are 10–30 µm and plant cells are typically 10–100 µm. Even smaller cell organelles such as mitochondria are typically 1-2 µm. Therefore, a proper model should account for the size of the specimen being studied. In addition, the size of vesicles dictates their membrane curvature which is an important factor in studying fusion proteins. SUVs have a higher membrane curvature and vesicles with high membrane curvature can promote membrane fusion faster than vesicles with lower membrane curvature such as GUVs.











































