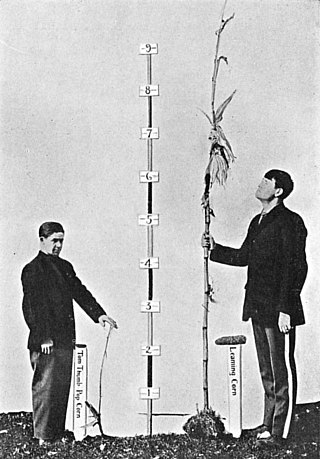
Heritability is a statistic used in the fields of breeding and genetics that estimates the degree of variation in a phenotypic trait in a population that is due to genetic variation between individuals in that population. The concept of heritability can be expressed in the form of the following question: "What is the proportion of the variation in a given trait within a population that is not explained by the environment or random chance?"
Twin studies are studies conducted on identical or fraternal twins. They aim to reveal the importance of environmental and genetic influences for traits, phenotypes, and disorders. Twin research is considered a key tool in behavioral genetics and in related fields, from biology to psychology. Twin studies are part of the broader methodology used in behavior genetics, which uses all data that are genetically informative – siblings studies, adoption studies, pedigree, etc. These studies have been used to track traits ranging from personal behavior to the presentation of severe mental illnesses such as schizophrenia.
A quantitative trait locus (QTL) is a locus that correlates with variation of a quantitative trait in the phenotype of a population of organisms. QTLs are mapped by identifying which molecular markers correlate with an observed trait. This is often an early step in identifying the actual genes that cause the trait variation.
Genetic association is when one or more genotypes within a population co-occur with a phenotypic trait more often than would be expected by chance occurrence.
In genetics, concordance is the probability that a pair of individuals will both have a certain characteristic given that one of the pair has the characteristic. Concordance can be measured with concordance rates, reflecting the odds of one person having the trait if the other does. Important clinical examples include the chance of offspring having a certain disease if the mother has it, if the father has it, or if both parents have it. Concordance among siblings is similarly of interest: what are the odds of a subsequent offspring having the disease if an older child does? In research, concordance is often discussed in the context of both members of a pair of twins. Twins are concordant when both have or both lack a given trait. The ideal example of concordance is that of identical twins, because the genome is the same, an equivalence that helps in discovering causation via deconfounding, regarding genetic effects versus epigenetic and environmental effects.
Coalescent theory is a model of how alleles sampled from a population may have originated from a common ancestor. In the simplest case, coalescent theory assumes no recombination, no natural selection, and no gene flow or population structure, meaning that each variant is equally likely to have been passed from one generation to the next. The model looks backward in time, merging alleles into a single ancestral copy according to a random process in coalescence events. Under this model, the expected time between successive coalescence events increases almost exponentially back in time. Variance in the model comes from both the random passing of alleles from one generation to the next, and the random occurrence of mutations in these alleles.

In genomics, a genome-wide association study, is an observational study of a genome-wide set of genetic variants in different individuals to see if any variant is associated with a trait. GWA studies typically focus on associations between single-nucleotide polymorphisms (SNPs) and traits like major human diseases, but can equally be applied to any other genetic variants and any other organisms.
In multivariate quantitative genetics, a genetic correlation is the proportion of variance that two traits share due to genetic causes, the correlation between the genetic influences on a trait and the genetic influences on a different trait estimating the degree of pleiotropy or causal overlap. A genetic correlation of 0 implies that the genetic effects on one trait are independent of the other, while a correlation of 1 implies that all of the genetic influences on the two traits are identical. The bivariate genetic correlation can be generalized to inferring genetic latent variable factors across > 2 traits using factor analysis. Genetic correlation models were introduced into behavioral genetics in the 1970s–1980s.
Behavioural genetics, also referred to as behaviour genetics, is a field of scientific research that uses genetic methods to investigate the nature and origins of individual differences in behaviour. While the name "behavioural genetics" connotes a focus on genetic influences, the field broadly investigates the extent to which genetic and environmental factors influence individual differences, and the development of research designs that can remove the confounding of genes and environment. Behavioural genetics was founded as a scientific discipline by Francis Galton in the late 19th century, only to be discredited through association with eugenics movements before and during World War II. In the latter half of the 20th century, the field saw renewed prominence with research on inheritance of behaviour and mental illness in humans, as well as research on genetically informative model organisms through selective breeding and crosses. In the late 20th and early 21st centuries, technological advances in molecular genetics made it possible to measure and modify the genome directly. This led to major advances in model organism research and in human studies, leading to new scientific discoveries.
Nested association mapping (NAM) is a technique designed by the labs of Edward Buckler, James Holland, and Michael McMullen for identifying and dissecting the genetic architecture of complex traits in corn. It is important to note that nested association mapping is a specific technique that cannot be performed outside of a specifically designed population such as the Maize NAM population, the details of which are described below.
The missing heritability problem refers to the difference between heritability estimates from genetic data and heritability estimates from twin and family data across many physical and mental traits, including diseases, behaviors, and other phenotypes. This is a problem that has significant implications for medicine, since a person's susceptibility to disease may depend more on the combined effect of all the genes in the background than on the disease genes in the foreground, or the role of genes may have been severely overestimated.
Predictive genomics is at the intersection of multiple disciplines: predictive medicine, personal genomics and translational bioinformatics. Specifically, predictive genomics deals with the future phenotypic outcomes via prediction in areas such as complex multifactorial diseases in humans. To date, the success of predictive genomics has been dependent on the genetic framework underlying these applications, typically explored in genome-wide association (GWA) studies. The identification of associated single-nucleotide polymorphisms underpin GWA studies in complex diseases that have ranged from Type 2 Diabetes (T2D), Age-related macular degeneration (AMD) and Crohn's disease.

Michael Edward "Mike" Goddard is a professorial fellow in animal genetics at the University of Melbourne, Australia.

Genetic variance is a concept outlined by the English biologist and statistician Ronald Fisher in his fundamental theorem of natural selection. In his 1930 book The Genetical Theory of Natural Selection, Fisher postulates that the rate of change of biological fitness can be calculated by the genetic variance of the fitness itself. Fisher tried to give a statistical formula about how the change of fitness in a population can be attributed to changes in the allele frequency. Fisher made no restrictive assumptions in his formula concerning fitness parameters, mate choices or the number of alleles and loci involved.
A human disease modifier gene is a modifier gene that alters expression of a human gene at another locus that in turn causes a genetic disease. Whereas medical genetics has tended to distinguish between monogenic traits, governed by simple, Mendelian inheritance, and quantitative traits, with cumulative, multifactorial causes, increasing evidence suggests that human diseases exist on a continuous spectrum between the two.

In genetics, a polygenic score (PGS) is a number that summarizes the estimated effect of many genetic variants on an individual's phenotype. The PGS is also called the polygenic index (PGI) or genome-wide score; in the context of disease risk, it is called a polygenic risk score or genetic risk score. The score reflects an individual's estimated genetic predisposition for a given trait and can be used as a predictor for that trait. It gives an estimate of how likely an individual is to have a given trait based only on genetics, without taking environmental factors into account; and it is typically calculated as a weighted sum of trait-associated alleles.

Complex traits are phenotypes that are controlled by two or more genes and do not follow Mendel’s Law of Dominance. They may have a range of expression which is typically continuous. Both environmental and genetic factors often impact the variation in expression. Human height is a continuous trait meaning that there is a wide range of heights. There are an estimated 50 genes that affect the height of a human. Environmental factors, like nutrition, also play a role in a human’s height. Other examples of complex traits include: crop yield, plant color, and many diseases including diabetes and Parkinson's disease. One major goal of genetic research today is to better understand the molecular mechanisms through which genetic variants act to influence complex traits. Complex Traits are also known as polygenic traits and multigenic traits.
The infinitesimal model, also known as the polygenic model, is a widely used statistical model in quantitative genetics and in genome-wide association studies. Originally developed in 1918 by Ronald Fisher, it is based on the idea that variation in a quantitative trait is influenced by an infinitely large number of genes, each of which makes an infinitely small (infinitesimal) contribution to the phenotype, as well as by environmental factors. In "The Correlation between Relatives on the Supposition of Mendelian Inheritance", the original 1918 paper introducing the model, Fisher showed that if a trait is polygenic, "then the random sampling of alleles at each gene produces a continuous, normally distributed phenotype in the population". However, the model does not necessarily imply that the trait must be normally distributed, only that its genetic component will be so around the average of that of the individual's parents. The model served to reconcile Mendelian genetics with the continuous distribution of quantitative traits documented by Francis Galton.
The Omnigenic Model, first proposed by Evan A. Boyle, Yang I. Li, and Jonathan K. Pritchard, describes a hypothesis regarding the heritability of complex traits. Expanding beyond polygenes, the authors propose that all genes expressed within a cell affect the expression of a given trait. In addition, the model states that the peripheral genes, ones that do not have a direct impact on expression, explain more heritability of traits than core genes, ones that have a direct impact on expression. The process that the authors propose that facilitates this effect is called “network pleiotropy”, in which peripheral genes can affect core genes, not by having a direct effect, but rather by virtue of being mediated within the same cell.
Personality traits are patterns of thoughts, feelings and behaviors that reflect the tendency to respond in certain ways under certain circumstances.





